Exam 7: Applications of Trigonometry and Vectors
Exam 1: Trigonometric Functions188 Questions
Exam 2: Acute Angles and Right Triangles204 Questions
Exam 3: Radian Measure and the Unit Circle167 Questions
Exam 4: Graphs of the Circular Functions137 Questions
Exam 5: Trigonometric Identities321 Questions
Exam 6: Inverse Circular Functions and Trigonometric Equations179 Questions
Exam 7: Applications of Trigonometry and Vectors103 Questions
Exam 8: Complex Numbers, Polar Equations, and Parametric Equations60 Questions
Select questions type
Find the component form of the indicated vector.
-Let . Find .
Free
(Multiple Choice)
4.8/5  (37)
(37)
Correct Answer:
B
Determine whether there is sufficient information for solving a triangle, with the given combination of angles and sides,
by the law of sines.
-A, c, and a
Free
(Multiple Choice)
4.9/5  (35)
(35)
Correct Answer:
A
Find the magnitude and direction angle (to the nearest tenth) for each vector. Give the measure of the direction angle as
an angle in [0,360°].
--12, 5
Free
(Multiple Choice)
4.9/5  (42)
(42)
Correct Answer:
A
Solve the problem.
-A ship leaves point A and travels directly north to point B. From point B, the ship then travels due east to point C. The magnitude of the vector from point A to point C is 140. Find the magnitude of
The northern vector. Find the magnitude of the eastern vector.
(Multiple Choice)
4.8/5  (35)
(35)
Find the angle between the pair of vectors to the nearest tenth of a degree.
-
(Multiple Choice)
4.9/5  (37)
(37)
Solve the problem.
-Two airplanes leave an airport at the same time, one going northwest (bearing 135°) at 414 mph and the other going east at 343 mph. How far apart are the planes after 4 hours (to the nearest
Mile)?
(Multiple Choice)
4.8/5  (38)
(38)
Draw a sketch to represent the vector. Refer to the vectors pictured here. 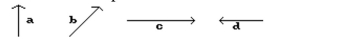 -
-
(Multiple Choice)
4.7/5  (32)
(32)
Find the magnitude and direction angle (to the nearest tenth) for each vector. Give the measure of the direction angle as
an angle in [0,360°].
-
(Multiple Choice)
4.9/5  (30)
(30)
Find the missing parts of the triangle. Round to the nearest tenth when necessary or to the nearest minute as appropriate.
-AB = 321 yd AC = 188 yd
BC = 161 yd
(Multiple Choice)
4.9/5  (36)
(36)
Find the missing parts of the triangle. Round to the nearest tenth when necessary or to the nearest minute as appropriate.
-a = 26 ft b = 39 ft
C = 40 ft
(Multiple Choice)
4.7/5  (36)
(36)
Find the component form of the indicated vector.
-Let . Find .
(Multiple Choice)
4.9/5  (29)
(29)
Solve the problem.
-An airplane is sighted at the same time by two ground observers who are 2 miles apart and both directly west of the airplane. They report the angles of elevation as 12° and 21°. How high is the
Airplane? Round to the nearest hundredth of a mile.
(Multiple Choice)
4.8/5  (31)
(31)
Solve the problem.
-A pilot wants to fly on a bearing of 62.5°. By flying due east, he finds that a 51-mph wind, blowing from the south, puts him on course. Find the ground speed of the plane.
(Multiple Choice)
4.7/5  (41)
(41)
Find the angle between the pair of vectors to the nearest tenth of a degree.
-6i + 3j, -2i + 3j
(Multiple Choice)
4.8/5  (42)
(42)
Find the missing parts of the triangle.
-A = 78° a = 36 yd
B = 73 yd
If necessary, round angles to the nearest degree and side lengths to the nearest yard.
(Multiple Choice)
4.8/5  (27)
(27)
Find the magnitude and direction angle (to the nearest tenth) for each vector. Give the measure of the direction angle as
an angle in [0,360°].
--16, 0
(Multiple Choice)
4.7/5  (34)
(34)
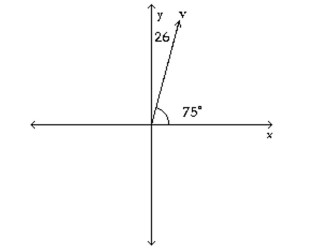
Determine whether there is sufficient information for solving a triangle, with the given combination of angles and sides,
by the law of sines.
-
(Multiple Choice)
4.9/5  (46)
(46)
Find the component form of the indicated vector.
-Let . Find .
(Multiple Choice)
4.8/5  (34)
(34)
Find the angle between the pair of vectors to the nearest tenth of a degree.
-
(Multiple Choice)
4.9/5  (40)
(40)
Solve the problem.
-Suppose you would like to cross a 219-foot wide river in a boat. Assume that the boat can travel 38 mph relative to the water and that the current is flowing west at the rate of 7 mph. What bearing
Should be chosen so that the boat will land at a point exactly across from its starting point? Give
Your answer to the nearest tenth of a degree.
(Multiple Choice)
4.8/5  (37)
(37)
Showing 1 - 20 of 103
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)