Exam 4: Exponential and Logarithmic Functions
Exam 1: Equations and Inequalities420 Questions
Exam 2: Functions and Graphs83 Questions
Exam 3: Polynomial and Rational Functions98 Questions
Exam 4: Exponential and Logarithmic Functions268 Questions
Exam 5: Systems of Equations and Inequalities287 Questions
Exam 6: Matrices and Determinants152 Questions
Exam 7: Conic Sections120 Questions
Exam 8: Sequences, Induction, and Probability303 Questions
Select questions type
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.8/5  (39)
(39)
Solve the problem.
-The size of the bear population at a national park increases at the rate of per year. If the size of the current population is 157 , find how many bears there should be in 6 years. Use the function and round to the nearest whole number.
(Multiple Choice)
4.8/5  (37)
(37)
Use Natural Logarithms
Evaluate or simplify the expression without using a calculator.
-
(Multiple Choice)
4.9/5  (34)
(34)
Use properties of logarithms to condense the logarithmic expression. Write the expression as a single logarithm
whose coefficient is 1. Where possible, evaluate logarithmic expressions.
-
(Multiple Choice)
4.8/5  (34)
(34)
Solve the equation by expressing each side as a power of the same base and then equating exponents.
-
(Multiple Choice)
4.9/5  (43)
(43)
Use properties of logarithms to condense the logarithmic expression. Write the expression as a single logarithm
whose coefficient is 1. Where possible, evaluate logarithmic expressions.
-
(Multiple Choice)
4.9/5  (34)
(34)
Graph the function.
-Use the graph of to obtain the graph of .

(Multiple Choice)
4.8/5  (43)
(43)
Graph the function.
-Use the graph of to obtain the graph of .
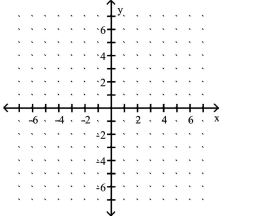
(Multiple Choice)
4.8/5  (30)
(30)
Use the Definition of a Logarithm to Solve Logarithmic Equations
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.8/5  (34)
(34)
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.7/5  (41)
(41)
Use the One-to-One Property of Logarithms to Solve Logarithmic Equations
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.9/5  (32)
(32)
Use the One-to-One Property of Logarithms to Solve Logarithmic Equations
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.9/5  (39)
(39)
Use the Power Rule
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.9/5  (38)
(38)
Solve the equation by expressing each side as a power of the same base and then equating exponents.
-
(Multiple Choice)
4.9/5  (36)
(36)
Use the One-to-One Property of Logarithms to Solve Logarithmic Equations
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.9/5  (42)
(42)
Use the Definition of a Logarithm to Solve Logarithmic Equations
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.8/5  (32)
(32)
Graph the function.
-Use the graph of to obtain the graph of .
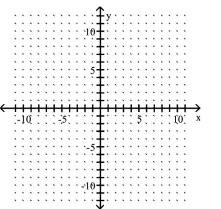
(Multiple Choice)
4.9/5  (30)
(30)
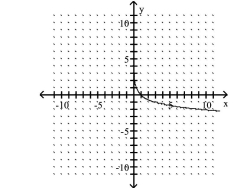
The graph of a logarithmic function is given. Select the function for the graph from the options.
-
(Multiple Choice)
4.7/5  (31)
(31)
Showing 61 - 80 of 268
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)