Exam 4: Exponential and Logarithmic Functions
Exam 1: Equations and Inequalities420 Questions
Exam 2: Functions and Graphs83 Questions
Exam 3: Polynomial and Rational Functions98 Questions
Exam 4: Exponential and Logarithmic Functions268 Questions
Exam 5: Systems of Equations and Inequalities287 Questions
Exam 6: Matrices and Determinants152 Questions
Exam 7: Conic Sections120 Questions
Exam 8: Sequences, Induction, and Probability303 Questions
Select questions type
Model Exponential Growth and Decay
Solve.
-The value of a particular investment follows a pattern of exponential growth. In the year 2000 , you invested money in a money market account. The value of your investment years after 2000 is given by the exponential growth model . When will the account be worth ?
(Multiple Choice)
4.9/5  (31)
(31)
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.9/5  (40)
(40)
Use the Definition of a Logarithm to Solve Logarithmic Equations
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.8/5  (30)
(30)
Use Compound Interest Formulas
Use the compound interest formulas A
-Suppose that you have $11,000 to invest. Which investment yields the greater return over 9 years: 7.5% compounded continuously or 7.6% compounded semiannually?
(Multiple Choice)
4.8/5  (40)
(40)
Solve the equation by expressing each side as a power of the same base and then equating exponents.
-
(Multiple Choice)
4.8/5  (26)
(26)
Solve the equation by expressing each side as a power of the same base and then equating exponents.
-
(Multiple Choice)
4.9/5  (33)
(33)
Use properties of logarithms to condense the logarithmic expression. Write the expression as a single logarithm
whose coefficient is 1. Where possible, evaluate logarithmic expressions.
-
(Multiple Choice)
4.8/5  (34)
(34)
Use the Power Rule
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.9/5  (30)
(30)
Use Natural Logarithms
Evaluate or simplify the expression without using a calculator.
-
(Multiple Choice)
4.9/5  (41)
(41)
Graph the function.
-Use the graph of to obtain the graph of .
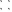
(Multiple Choice)
4.9/5  (37)
(37)
Solve the exponential equation. Use a calculator to obtain a decimal approximation, correct to two decimal places,
for the solution.
-
(Multiple Choice)
4.7/5  (30)
(30)
Use Compound Interest Formulas
Use the compound interest formulas A
-Find the accumulated value of an investment of $19,000 at 8% compounded annually for 10 years.
(Multiple Choice)
4.9/5  (34)
(34)
Solve the problem.
-The population in a particular country is growing at the rate of per year. If people lived there in 1999 , how many will there be in the year 2004 ? Use and round to the nearest ten-thousand.
(Multiple Choice)
4.9/5  (33)
(33)
Use the Power Rule
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.9/5  (37)
(37)
Solve the exponential equation. Use a calculator to obtain a decimal approximation, correct to two decimal places,
for the solution.
-
(Multiple Choice)
4.7/5  (35)
(35)
Use properties of logarithms to condense the logarithmic expression. Write the expression as a single logarithm
whose coefficient is 1. Where possible, evaluate logarithmic expressions.
-
(Multiple Choice)
4.9/5  (39)
(39)
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.9/5  (32)
(32)
Solve the problem.
-The rabbit population in a forest area grows at the rate of monthly. If there are 190 rabbits in July, find how many rabbits (rounded to the nearest whole number) should be expected by next July. Use
(Multiple Choice)
4.9/5  (37)
(37)
Showing 101 - 120 of 268
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)