Exam 4: Exponential and Logarithmic Functions
Exam 1: Equations and Inequalities420 Questions
Exam 2: Functions and Graphs83 Questions
Exam 3: Polynomial and Rational Functions98 Questions
Exam 4: Exponential and Logarithmic Functions268 Questions
Exam 5: Systems of Equations and Inequalities287 Questions
Exam 6: Matrices and Determinants152 Questions
Exam 7: Conic Sections120 Questions
Exam 8: Sequences, Induction, and Probability303 Questions
Select questions type
Use the One-to-One Property of Logarithms to Solve Logarithmic Equations
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.8/5  (25)
(25)
Use the Power Rule
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.8/5  (30)
(30)
Solve the problem.
-The formula models the population of a particular city, in thousands, years after 1998 . When will the population of the city reach 353 thousand?
(Multiple Choice)
4.7/5  (31)
(31)
The graph of an exponential function is given. Select the function for the graph from the functions listed.
-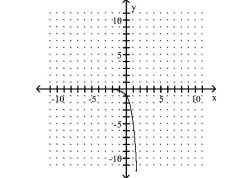
(Multiple Choice)
4.9/5  (47)
(47)
The graph of a logarithmic function is given. Select the function for the graph from the options.
-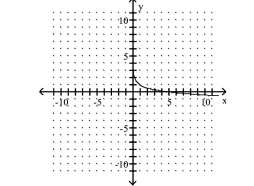
(Multiple Choice)
4.7/5  (28)
(28)
Use the Quotient Rule
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.9/5  (29)
(29)
Graph the function.
-Use the graph of to obtain the graph of

(Multiple Choice)
4.9/5  (38)
(38)
Use common logarithms or natural logarithms and a calculator to evaluate to four decimal places
-
(Multiple Choice)
5.0/5  (38)
(38)
Solve the problem.
-Use the mathematical model for power gain, , where is the output power in watts and is the input power in watts. Determine the power gain , in decibels, for an amplifier with an output of 19 watts and an input of watts. Round to five decimal places if necessary.
(Multiple Choice)
4.7/5  (31)
(31)
The graph of a logarithmic function is given. Select the function for the graph from the options.
-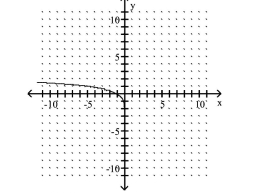
(Multiple Choice)
4.8/5  (41)
(41)
Solve the problem.
-Larry has $2200 to invest and needs $2700 in 12 years. What annual rate of return will he need to get in order to accomplish his goal, if interest is compounded continuously? (Round your answer to two
Decimals.)
(Multiple Choice)
4.8/5  (24)
(24)
Use the One-to-One Property of Logarithms to Solve Logarithmic Equations
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.9/5  (39)
(39)
Use the One-to-One Property of Logarithms to Solve Logarithmic Equations
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.9/5  (38)
(38)
Use the Quotient Rule
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.8/5  (38)
(38)
Model Exponential Growth and Decay
Solve.
-The population of a particular country was 23 million in 1981 ; in 1987 , it was 32 million. The exponential growth function describes the population of this country t years after 1981. Use the fact that 6 years after 1981 the population increased by 9 million to find to three decimal places.
(Multiple Choice)
4.7/5  (40)
(40)
Use properties of logarithms to expand the logarithmic expression as much as possible. Where possible, evaluate
logarithmic expressions without using a calculator.
-
(Multiple Choice)
4.9/5  (31)
(31)
Approximate the number using a calculator. Round your answer to three decimal places.
-
(Multiple Choice)
4.8/5  (39)
(39)
Showing 141 - 160 of 268
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)