Explore all topics
Start Free Trial
Need Help? Contact us
back arrowfull exam logo
search
ai logoChat with AI
Servicesarrow
Discoverarrow

Topics

Explore all topics
Discover more topics

Exam 11: Introduction to Trigonometry

arrow
Exam 1: Line and Angle Relationships13 Questionsadd course
Exam 2: Parallel Lines13 Questionsadd course
Exam 3: Triangles16 Questionsadd course
Exam 4: Quadrilaterals14 Questionsadd course
Exam 5: Similar Triangles12 Questionsadd course
Exam 6: Circles10 Questionsadd course
Exam 7: Locus and Concurrence4 Questionsadd course
Exam 8: Areas of Polygons and Circles5 Questionsadd course
Exam 9: Surfaces and Solids4 Questionsadd course
Exam 10: Analytical Geometry8 Questionsadd course
Exam 11: Introduction to Trigonometry4 Questionsadd course
Exam 12: Geometry Problems: Complementary Angles, Collinear Points, and Similar Triangles916 Questionsadd course
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags
search iconSearch Question
flashcardsStudy Flashcards
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags

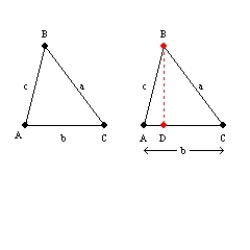-Use the drawings provided to prove the following theorem. The area of an acute triangle equals one-half the product of the lengths of two sides of a triangle and the sine of the included angle. Given: Acute Prove: -Use the drawings provided to prove the following theorem. "The area of an acute triangle equals one-half the product of the lengths of two sides of a triangle and the sine of the included angle." Given: Acute -Use the drawings provided to prove the following theorem. The area of an acute triangle equals one-half the product of the lengths of two sides of a triangle and the sine of the included angle. Given: Acute Prove: Prove: -Use the drawings provided to prove the following theorem. The area of an acute triangle equals one-half the product of the lengths of two sides of a triangle and the sine of the included angle. Given: Acute Prove: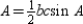

Free
(Essay)
4.8/5
(40)
Question 1
Correct Answer:
Verified

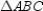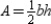The area of The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . is given by The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . . 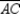Considering the auxiliary altitude The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . from vertex B
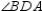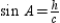to side The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . , we see that The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . is a right angle. 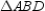Then The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . 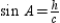in right triangle The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . .
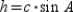From The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . , it follows that The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . . By substitution, the area formula The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . becomes The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes so we have . so we have The area of is given by . Considering the auxiliary altitude from vertex B to side , we see that is a right angle. Then in right triangle . From , it follows that . By substitution, the area formula becomes 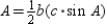so we have . .

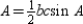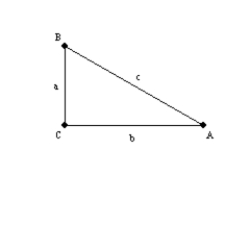-For the right triangle with right , prove that . -For the right triangle -For the right triangle with right , prove that . with right -For the right triangle with right , prove that . , prove that -For the right triangle with right , prove that . .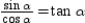

Free
(Essay)
4.8/5
(35)
Question 2
Correct Answer:
Verified

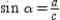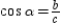By definition, By definition, , , and . Then , which can also be written . Then . Thus, . , By definition, , , and . Then , which can also be written . Then . Thus, . , and By definition, , , and . Then , which can also be written . Then . Thus, . . Then By definition, , , and . Then , which can also be written . Then . Thus, . , which can also be written By definition, , , and . Then , which can also be written . Then . Thus, . . Then By definition, , , and . Then , which can also be written . Then . Thus, . . Thus, By definition, , , and 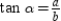. Then 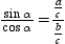, which can also be written 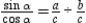. Then 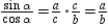. Thus, . .

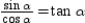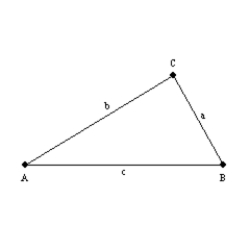-For the right triangle with right , prove that . -For the right triangle -For the right triangle with right , prove that . with right -For the right triangle with right , prove that . , prove that -For the right triangle with right , prove that . .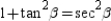

Free
(Essay)
4.8/5
(37)
Question 3
Correct Answer:
Verified

In right triangle In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . , In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . . Because In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . is the reciprocal of In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . , we know that In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . . Now In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . , so In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . or In right triangle 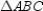, 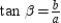. Because 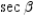is the reciprocal of 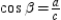, we know that 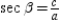. Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . .
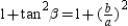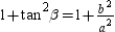Then In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . . Because In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . is a right triangle with right angle at vertex C, In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . . Then In right triangle , . Because is the reciprocal of , we know that . Now , so or . Then . Because is a right triangle with right angle at vertex C, . Then ; that is, . ; that is, In right triangle , . Because is the reciprocal of , we know that . Now , so or 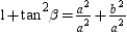. Then 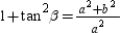. Because 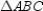is a right triangle with right angle at vertex C, 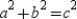. Then 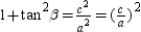; that is, . .

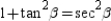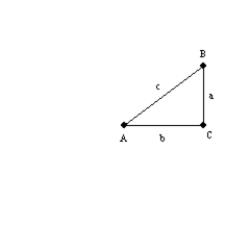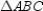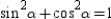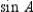-For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and have the same meaning. -For the right triangle -For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and have the same meaning. with right -For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and have the same meaning. 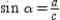, prove that -For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and have the same meaning. . 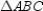Note that -For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and have the same meaning. 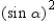and -For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and have the same meaning. are the same and that -For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and have the same meaning. as shown in -For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and have the same meaning. ; also, -For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and have the same meaning. and -For the right triangle with right , prove that . Note that and are the same and that as shown in ; also, and 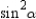have the same meaning. have the same meaning.

(Essay)
4.8/5
(40)
Question 4
close modal

Filters

  • Essay(0)
  • Multiple Choice(0)
  • Short Answer(0)
  • True False(0)
  • Matching(0)