Explore all topics
Start Free Trial
Need Help? Contact us
back arrowfull exam logo
search
ai logoChat with AI
Servicesarrow
Discoverarrow

Topics

Explore all topics
Discover more topics

Exam 2: Parallel Lines

arrow
Exam 1: Line and Angle Relationships13 Questionsadd course
Exam 2: Parallel Lines13 Questionsadd course
Exam 3: Triangles16 Questionsadd course
Exam 4: Quadrilaterals14 Questionsadd course
Exam 5: Similar Triangles12 Questionsadd course
Exam 6: Circles10 Questionsadd course
Exam 7: Locus and Concurrence4 Questionsadd course
Exam 8: Areas of Polygons and Circles5 Questionsadd course
Exam 9: Surfaces and Solids4 Questionsadd course
Exam 10: Analytical Geometry8 Questionsadd course
Exam 11: Introduction to Trigonometry4 Questionsadd course
Exam 12: Geometry Problems: Complementary Angles, Collinear Points, and Similar Triangles916 Questionsadd course
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags
search iconSearch Question
flashcardsStudy Flashcards
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags

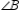Use an indirect proof to complete the following problem. Given: Use an indirect proof to complete the following problem. Given: (not shown) Prove: and cannot both be right angles. (not shown) Prove: Use an indirect proof to complete the following problem. Given: (not shown) Prove: and cannot both be right angles. and Use an indirect proof to complete the following problem. Given: (not shown) Prove: and cannot both be right angles. cannot both be right angles.

Free
(Essay)
4.9/5
(30)
Question 1
Correct Answer:
Verified

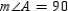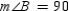Suppose that Suppose that and are both be right angles. Then and . By the Protractor Postulate, . Then . But this last statement contradicts the fact that the sum of teh three interior angles of a triangle is exactly 180. Thus, the supposition must be false and it follows that and cannot both be right angles. and Suppose that and are both be right angles. Then and . By the Protractor Postulate, . Then . But this last statement contradicts the fact that the sum of teh three interior angles of a triangle is exactly 180. Thus, the supposition must be false and it follows that and cannot both be right angles. are both be right angles. 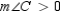Then Suppose that and are both be right angles. Then and . By the Protractor Postulate, . Then . But this last statement contradicts the fact that the sum of teh three interior angles of a triangle is exactly 180. Thus, the supposition must be false and it follows that and cannot both be right angles. 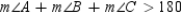and Suppose that and are both be right angles. Then and . By the Protractor Postulate, . Then . But this last statement contradicts the fact that the sum of teh three interior angles of a triangle is exactly 180. Thus, the supposition must be false and it follows that and cannot both be right angles. .
By the Protractor Postulate, Suppose that and are both be right angles. Then and . By the Protractor Postulate, . Then . But this last statement contradicts the fact that the sum of teh three interior angles of a triangle is exactly 180. Thus, the supposition must be false and it follows that and cannot both be right angles. . Then Suppose that and are both be right angles. Then and . By the Protractor Postulate, . Then . But this last statement contradicts the fact that the sum of teh three interior angles of a triangle is exactly 180. Thus, the supposition must be false and it follows that and cannot both be right angles. . But this last
statement contradicts the fact that the sum of teh three interior angles of a triangle is exactly 180. Thus, the supposition must be false and it follows that Suppose that and are both be right angles. Then and . By the Protractor Postulate, . Then . But this last statement contradicts the fact that the sum of teh three interior angles of a triangle is exactly 180. Thus, the supposition must be false and it follows that and cannot both be right angles. and Suppose that and are both be right angles. Then and . By the Protractor Postulate, . Then . But this last statement contradicts the fact that the sum of teh three interior angles of a triangle is exactly 180. Thus, the supposition must be false and it follows that and cannot both be right angles. cannot both be right angles.

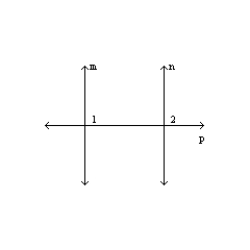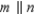-Supply missing statements and missing reasons for the following proof. Given: and transversal p; is a right angle Prove: is a right angle S1. and transversal p R1. S2. R2. S3. R3. Congruent measures have equal measures. S4. R4. S5. R5. Substitution Property of Equality S6. R6. Definition of a right angle -Supply missing statements and missing reasons for the following proof. Given: -Supply missing statements and missing reasons for the following proof. Given: and transversal p; is a right angle Prove: is a right angle S1. and transversal p R1. S2. R2. S3. R3. Congruent measures have equal measures. S4. R4. S5. R5. Substitution Property of Equality S6. R6. 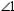Definition of a right angle and transversal p; -Supply missing statements and missing reasons for the following proof. Given: and transversal p; is a right angle Prove: is a right angle S1. and transversal p R1. S2. R2. S3. R3. Congruent measures have equal measures. S4. R4. S5. R5. Substitution Property of Equality S6. R6. 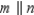Definition of a right angle is a right angle Prove: -Supply missing statements and missing reasons for the following proof. Given: and transversal p; is a right angle Prove: is a right angle S1. and transversal p R1. S2. R2. S3. R3. Congruent measures have equal measures. S4. R4. S5. R5. Substitution Property of Equality S6. R6. Definition of a right angle is a right angle S1. -Supply missing statements and missing reasons for the following proof. Given: and transversal p; is a right angle Prove: is a right angle S1. and transversal p R1. S2. R2. S3. R3. Congruent measures have equal measures. S4. R4. S5. R5. Substitution Property of Equality S6. R6. Definition of a right angle and transversal p R1. S2. -Supply missing statements and missing reasons for the following proof. Given: and transversal p; is a right angle Prove: is a right angle S1. and transversal p R1. S2. R2. S3. R3. Congruent measures have equal measures. S4. R4. S5. R5. Substitution Property of Equality S6. R6. Definition of a right angle R2. S3. R3. Congruent measures have equal measures. S4. -Supply missing statements and missing reasons for the following proof. Given: and transversal p; is a right angle Prove: is a right angle S1. and transversal p R1. S2. R2. S3. R3. Congruent measures have equal measures. S4. 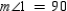R4. S5. R5. Substitution Property of Equality S6. R6. Definition of a right angle R4. S5. R5. Substitution Property of Equality S6. R6. Definition of a right angle

Free
(Essay)
4.8/5
(39)
Question 2
Correct Answer:
Verified

R1. Given
R2. If 2 parallel lines are cut by a trans, corresponding angles are congruent.
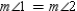S3. R1. Given R2. If 2 parallel lines are cut by a trans, corresponding angles are congruent. S3. R4. Given S5. S6. is a right angle R4. Given
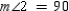S5. R1. Given R2. If 2 parallel lines are cut by a trans, corresponding angles are congruent. S3. R4. Given S5. S6. is a right angle S6. R1. Given R2. If 2 parallel lines are cut by a trans, corresponding angles are congruent. S3. R4. Given S5. S6. is a right angle is a right angle

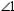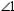Use an indirect proof to complete the following problem. Given: Use an indirect proof to complete the following problem. Given: and are supplementary (no drawing) Prove: and are not both obtuse angles. and Use an indirect proof to complete the following problem. Given: and are supplementary (no drawing) Prove: and are not both obtuse angles. are supplementary (no drawing) Prove: Use an indirect proof to complete the following problem. Given: and are supplementary (no drawing) Prove: and are not both obtuse angles. and Use an indirect proof to complete the following problem. Given: and are supplementary (no drawing) Prove: and are not both obtuse angles. are not both obtuse angles.

Free
(Essay)
4.9/5
(31)
Question 3
Correct Answer:
Verified

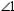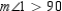Suppose that Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. and Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. are both obtuse angles. 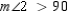Then Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. 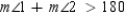and Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. .
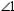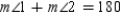It follows that Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. . But it is given that Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. 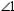and Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. are supplementary, so that Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. .
With a contradiction of the known fact, it follows that the supposition must be false; thus, Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. and Suppose that and are both obtuse angles. Then and . It follows that . But it is given that and are supplementary, so that . With a contradiction of the known fact, it follows that the supposition must be false; thus, and are not both obtuse angles. are not both obtuse angles.

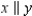-Supply missing statements and reasons for the foloowing proof. Given: is supplementary to Prove: S1. R1. S2. is supp. to R2. If the ext. sides of 2 adj. angles form a line, the angles are supp. S3. R3. Angles supp. to the same angle are congruent. S4. R4. -Supply missing statements and reasons for the foloowing proof. Given: -Supply missing statements and reasons for the foloowing proof. Given: is supplementary to Prove: S1. R1. S2. is supp. to R2. If the ext. sides of 2 adj. angles form a line, the angles are supp. S3. R3. Angles supp. to the same angle are congruent. S4. R4. is supplementary to -Supply missing statements and reasons for the foloowing proof. Given: is supplementary to Prove: S1. R1. S2. is supp. to R2. If the ext. sides of 2 adj. angles form a line, the angles are supp. S3. R3. Angles supp. to the same angle are congruent. S4. R4. Prove: -Supply missing statements and reasons for the foloowing proof. Given: is supplementary to Prove: S1. R1. S2. is supp. to R2. If the ext. sides of 2 adj. angles form a line, the angles are supp. S3. R3. Angles supp. to the same angle are congruent. S4. R4. S1. R1. S2. -Supply missing statements and reasons for the foloowing proof. Given: is supplementary to Prove: S1. R1. S2. is supp. to R2. If the ext. sides of 2 adj. angles form a line, the angles are supp. S3. R3. Angles supp. to the same angle are congruent. S4. R4. is supp. to -Supply missing statements and reasons for the foloowing proof. Given: is supplementary to Prove: S1. R1. S2. is supp. to R2. If the ext. sides of 2 adj. angles form a line, the angles are supp. S3. R3. Angles supp. to the same angle are congruent. S4. R4. R2. If the ext. sides of 2 adj. angles form a line, the angles are supp. S3. R3. Angles supp. to the same angle are congruent. S4. R4.

(Essay)
4.8/5
(39)
Question 4

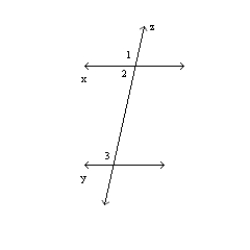-In the figure, x || y and transversal z. Explain why and must be supplementary. -In the figure, x || y and transversal z. Explain why -In the figure, x || y and transversal z. Explain why and must be supplementary. and -In the figure, x || y and transversal z. Explain why and must be supplementary. must be supplementary.

(Essay)
4.9/5
(38)
Question 5

Explain the following statement. The measure of each interior angle of an equiangular triangle is 60.

(Essay)
4.8/5
(31)
Question 6

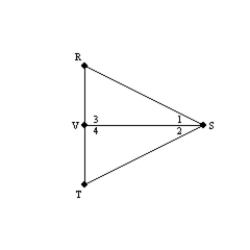-Supply missing statements and missing reasons for the following proof. Given: so that bisects ; also, Prove: S1. so that bisects R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent. -Supply missing statements and missing reasons for the following proof. Given: -Supply missing statements and missing reasons for the following proof. Given: 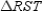so that 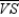bisects 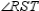; also, Prove: S1. so that bisects R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent. 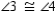so that -Supply missing statements and missing reasons for the following proof. Given: so that bisects ; also, Prove: S1. so that bisects R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent. bisects -Supply missing statements and missing reasons for the following proof. Given: so that bisects ; also, Prove: S1. so that bisects R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent. ; also, -Supply missing statements and missing reasons for the following proof. Given: so that bisects ; also, Prove: S1. so that bisects R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent. Prove: -Supply missing statements and missing reasons for the following proof. Given: so that bisects ; also, Prove: S1. so that bisects R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent. S1. -Supply missing statements and missing reasons for the following proof. Given: so that bisects ; also, Prove: S1. so that bisects R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent. so that -Supply missing statements and missing reasons for the following proof. Given: so that bisects ; also, Prove: S1. so that bisects R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent. bisects -Supply missing statements and missing reasons for the following proof. Given: so that bisects ; also, Prove: S1. 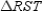so that 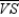bisects 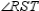R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent. R1. S2. R2. S3. R3. Given S4. R4. If 2 angles of one triangle are congruent to 2 angles of a second triangle, then the third angles of these triangles are also congruent.

(Essay)
4.7/5
(34)
Question 7

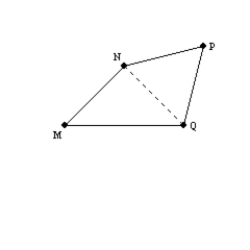-Using the drawing provided, explain the following statement. The sum of the interior angles of a quadrilateral is 360. -Using the drawing provided, explain the following statement. The sum of the interior angles of a quadrilateral is 360.

(Essay)
4.8/5
(38)
Question 8

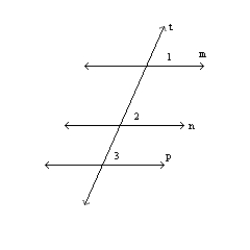-Supply missing statements in the following proof. Given: and Prove: S1. R1. Given S2. R2. If 2 parallel lines are cut by a transversal, corr. angles are congruent. S3. R3. Given S4. R4. Same as #2. S5. R5. Transitive Property of Congruence S6. R6. If 2 lines are cut by a transversal so that corresponding angles are congruent, then these lines are parallel. -Supply missing statements in the following proof. Given: -Supply missing statements in the following proof. Given: 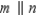and Prove: S1. R1. Given S2. R2. If 2 parallel lines are cut by a transversal, corr. angles are congruent. S3. R3. Given S4. R4. Same as #2. S5. R5. Transitive Property of Congruence S6. R6. If 2 lines are cut by a transversal so that corresponding angles are congruent, then these lines are parallel. and -Supply missing statements in the following proof. Given: and Prove: S1. R1. Given S2. R2. If 2 parallel lines are cut by a transversal, corr. angles are congruent. S3. R3. Given S4. R4. Same as #2. S5. R5. Transitive Property of Congruence S6. R6. If 2 lines are cut by a transversal so that corresponding angles are congruent, then these lines are parallel. Prove: -Supply missing statements in the following proof. Given: and 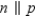Prove: 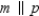S1. R1. Given S2. R2. If 2 parallel lines are cut by a transversal, corr. angles are congruent. S3. R3. Given S4. R4. Same as #2. S5. R5. Transitive Property of Congruence S6. R6. If 2 lines are cut by a transversal so that corresponding angles are congruent, then these lines are parallel. S1. R1. Given S2. R2. If 2 parallel lines are cut by a transversal, corr. angles are congruent. S3. R3. Given S4. R4. Same as #2. S5. R5. Transitive Property of Congruence S6. R6. If 2 lines are cut by a transversal so that corresponding angles are congruent, then these lines are parallel.

(Essay)
4.8/5
(33)
Question 9

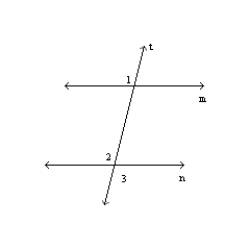-Supply missing reasons for this proof. Given: m || n Prove: S1. m || n R1. S2. R2. S3. R3. S4. R4. -Supply missing reasons for this proof. Given: m || n Prove: -Supply missing reasons for this proof. Given: m || n Prove: S1. m || n R1. S2. R2. S3. R3. S4. R4. S1. m || n R1. S2. -Supply missing reasons for this proof. Given: m || n Prove: S1. m || n R1. S2. R2. S3. R3. S4. R4. R2. S3. -Supply missing reasons for this proof. Given: m || n Prove: S1. m || n R1. S2. R2. S3. R3. S4. R4. R3. S4. -Supply missing reasons for this proof. Given: m || n Prove: S1. m || n R1. S2. R2. S3. R3. S4. R4. R4.

(Essay)
4.9/5
(32)
Question 10

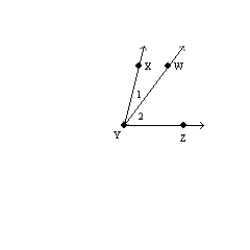-Use an indirect proof to complete the following problem. Given: is not congruent to Prove: does not bisect -Use an indirect proof to complete the following problem. Given: -Use an indirect proof to complete the following problem. Given: 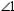is not congruent to Prove: does not bisect is not congruent to -Use an indirect proof to complete the following problem. Given: is not congruent to Prove: does not bisect Prove: -Use an indirect proof to complete the following problem. Given: is not congruent to Prove: does not bisect does not bisect -Use an indirect proof to complete the following problem. Given: is not congruent to Prove: 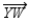does not bisect

(Essay)
4.8/5
(34)
Question 11

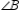-In the triangle shown, is a right angle.Explain why and are complementary. -In the triangle shown, -In the triangle shown, is a right angle.Explain why and are complementary. is a right angle.Explain why -In the triangle shown, is a right angle.Explain why and are complementary. and -In the triangle shown, is a right angle.Explain why and are complementary. are complementary.

(Essay)
4.7/5
(28)
Question 12

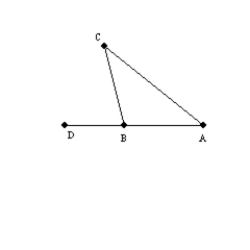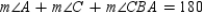-Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. R6. -Supply missing reasons for the following proof. Given: -Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. R6. with D-B-A Prove: -Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. R6. S1. 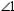-Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. R6. with D-B-A R1. S2. 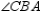-Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. R6. R2. S3. 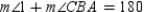-Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. R6. 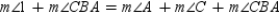and -Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. R6. are supp. R3. S4. -Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. R6. R4. S5. -Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. R6. R5. S6. -Supply missing reasons for the following proof. Given: with D-B-A Prove: S1. with D-B-A R1. S2. R2. S3. and are supp. R3. S4. R4. S5. R5. S6. 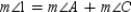R6. R6.

(Essay)
4.7/5
(26)
Question 13
close modal

Filters

  • Essay(0)
  • Multiple Choice(0)
  • Short Answer(0)
  • True False(0)
  • Matching(0)