Exam 10: One-Sample Tests of Hypothesis

To compare the effect of weather on sales of soft drinks, a soda manufacturer sampled two regions Of the country with the following results. Is there a difference in sales between the 2 regions? 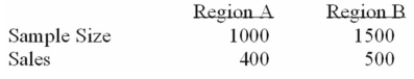i. The alternate hypothesis is pa - pb ≠ 0. ii. The pooled estimate of the population proportion is 0.36. iii. Using the 1% level of significance, the critical value is ±1.96.

The results of a mathematics placement exam at Mercy College for two campuses are as follows: 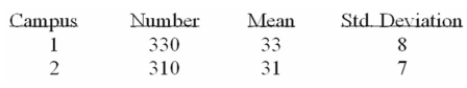What is the p-value if the computed test statistic is 4.1?

To compare the effect of weather on sales of soft drinks, a soda manufacturer sampled two regions Of the country with the following results. Is there a difference in sales between the 2 regions? 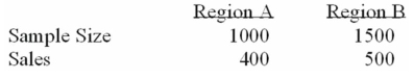i. The alternate hypothesis is pa - pb ≠ 0. ii. The proportion of sales made in Market Area 1 is 0.40. iii. The proportion of sales made in Market Area 2 is 0.33.

A recent study compared the time spent together by single and dual-earner couples. According to The records kept during the study, the mean amount of time spent together watching TV among Single-earner couples was 64 minutes per day, with a standard deviation of 15.5 minutes. For the Dual-earner couples, the mean time was 48.4 and the standard deviation was 18.1. At a 0.01 Significance level, can we conclude that the single-earner couples on average spend more time Watching TV together? There were 20 single-earner and 12 dual-earner couples studied. State the Decision rule, the value of the test statistic, and your decision.

If the decision is to reject the null hypothesis at the 5% level of significance, what are the Acceptable alternate hypothesis and rejection region?

Suppose we test H0: π1 = π2 at the 0.05 level of significance. If the z-test statistic is -1.07, what is Our decision?

The employees at the East Vancouver office of a multinational company are demanding higher Salaries than those offered at the company office located in Oshawa Ontario. Their justification for The pay difference is that the difference between the average price of single-family houses in East Vancouver and that in Oshawa is more than $60,000. Before making a decision, the company Management wants to study the difference in the prices of single-family houses for sale at the two Locations. The results of their search of recent house sales are as follows (in $1000, rounded to the nearest Thousand): 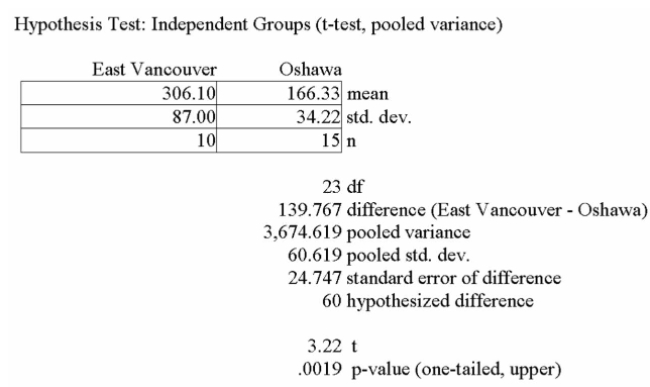What is the decision at the 5% level of significance?

What is the critical value for a one-tailed hypothesis test in which a null hypothesis is tested at the 5% level of significance based on two samples, both sample sizes are 13?

A company is researching the effectiveness of a new website design to decrease the time to access A website. Five website users were randomly selected, and their times (in seconds) to access the Website with the old and new designs were recorded. To compare the times, they computed (new Website design time - old website design time). The results follow: 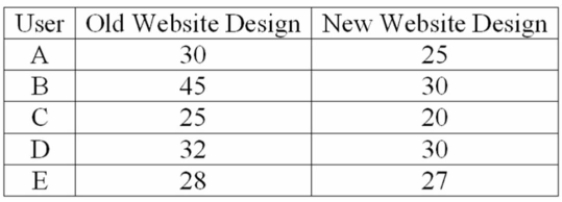For a 0.01 significance level, what is the critical value?

i. If the null hypothesis states that there is no difference between the mean income of males and the Mean income of females, then the test is one-tailed. ii. If we are testing for the difference between two population means, it is assumed that the sample Observations from one population are independent of the sample observations from the other Population. iii. When sample sizes are less than 30, a test for the differences between two population means Has n - 1 degrees of freedom.

A local retail business wishes to determine if there is a difference in preferred indoor temperature Between men and women. A random sample of data is collected, with the following results (note: There are slight differences between Excel and MegaStat output in this test): 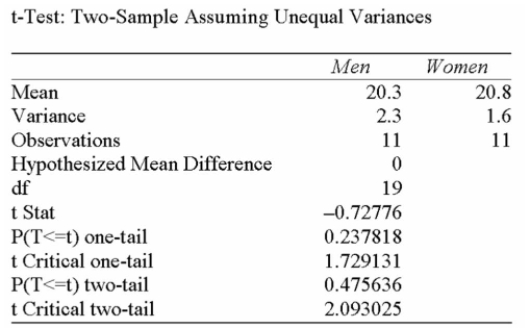Using a 0.05 level of significance, can we conclude that there is indeed a difference in the Temperature that men prefer compared to women? What is the null hypothesis if we assume men to be group 1 and women group 2?

A recent study compared the time spent together by single and dual-earner couples. According to The records kept during the study, the mean amount of time spent together watching TV among Single-earner couples was 60 minutes per day, with a standard deviation of 15.5 minutes. For the Dual-earner couples, the mean time was 48.4 and the standard deviation was 18.1. At a 0.01 Significance level, can we conclude that the single-earner couples on average spend more time Watching TV together? There were 12 single-earner and 12 dual-earner couples studied. State the Decision rule, the value of the test statistic, and your decision.

The employees at the East Vancouver office of a multinational company are demanding higher Salaries than those offered at the company office located in Oshawa Ontario. Their justification for The pay difference is that the difference between the average price of single-family houses in East Vancouver and that in Oshawa is more than $60,000. Before making a decision, the company Management wants to study the difference in the prices of single-family houses for sale at the two Locations. The results of their search of recent house sales are as follows (in $000, rounded to the nearest Thousand): Assuming that the population distributions are approximately normal, can we conclude at the 0.05 Significance level that the difference between the two population means is greater than $60,000? What is the decision at the 5% level of significance?

A local retail business wishes to determine if there is a difference in preferred indoor temperature Between men and women. A random sample of data is collected, with the following results (note: There are slight differences between Excel and MegaStat output in this test): 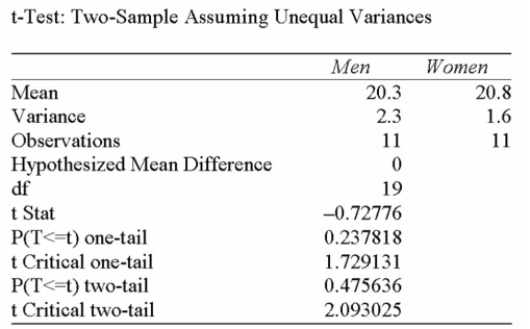If you use the 5% level of significance, what is the critical t value?

Of 150 adults who tried a new peach-flavoured peppermint patty, 81 rated it excellent. Of 200 Children sampled, 123 rated it excellent. Using the 0.10 level of significance, can we conclude that There is a significant difference in the proportion of adults and the proportion of children who rate The new flavour as excellent? State the decision rule, the value of the test statistic, and your Decision.

The employees at the East Vancouver office of a multinational company are demanding higher Salaries than those offered at the company office located in Oshawa Ontario. Their justification for The pay difference is that the difference between the average price of single-family houses in East Vancouver and that in Oshawa is more than $60,000. Before making a decision, the company Management wants to study the difference in the prices of single-family houses for sale at the two Locations. The results of their search of recent house sales are as follows (in $1000, rounded to the nearest Thousand): Assuming that the population distributions are approximately normal, can we conclude at the 0.05 Significance level that the difference between the two population means is greater than $60,000? If we let East Vancouver be population 1 and Oshawa be population 2, what is the null hypothesis?

A local retail business wishes to determine if there is a difference in preferred indoor temperature Between men and women. A random sample of data is collected, with the following results (note: There are slight differences between Excel and MegaStat output in this test): 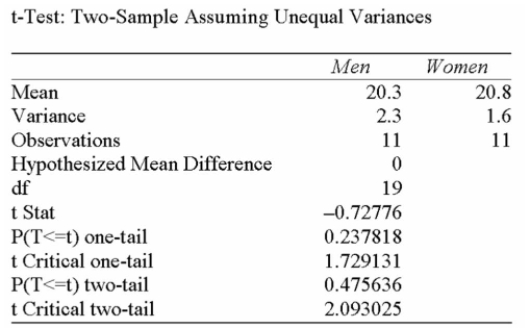What is the degree of freedom?

To compare the effect of weather on sales of soft drinks, a soda manufacturer sampled two regions Of the country with the following results. Is there a difference in sales between the 2 regions? 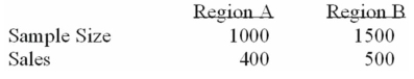i. The null hypothesis is pa - pb > 0. ii. The alternate hypothesis is pa - pb ≠ 0. iii. The pooled estimate of the population proportion is 0.36.

To compare the effect of weather on sales of soft drinks, a soda manufacturer sampled two regions Of the country with the following results. Is there a difference in sales between the 2 regions? 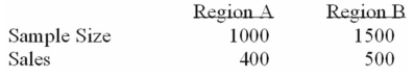i. The alternate hypothesis is pa - pb ≠ 0. ii. Using the 1% level of significance, the critical value is ±2.58. iii. The z-statistic is 3.40.

i. If samples taken from two populations are not independent, then a test of paired differences is Applied. ii. The paired difference test has (n1 + n2 - 2) degrees of freedom. iii. A statistics professor wants to compare grades of two different groups of students taking the Same course in two different sections. This is an example of a paired sample.