Exam 1: Functions and Change
Exam 1: Functions and Change204 Questions
Exam 2: Rate of Change: the Derivative132 Questions
Exam 3: Shortcuts to Differentiation178 Questions
Exam 4: Using the Derivative94 Questions
Exam 5: Accumulated Change: the Definite Integral93 Questions
Exam 6: Antiderivatives and Applications122 Questions
Exam 7: Probability68 Questions
Exam 8: Functions of Several Variables134 Questions
Exam 9: Mathematical Modeling Using Differential Equations121 Questions
Exam 10: Geometric Series65 Questions
Select questions type
The cost of producing q items in a tortilla factory is given by 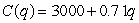 dollars. The revenue from sales of q items is
dollars. The revenue from sales of q items is 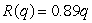 dollars.
A. For what values of q does the tortilla factory make a profit?
B. Write a formula for profit as a function of q.
dollars.
A. For what values of q does the tortilla factory make a profit?
B. Write a formula for profit as a function of q.
(Short Answer)
4.7/5  (40)
(40)
The number of bacteria in milk grows at a rate of 11% per day once the milk has been bottled. When milk is put in the bottles, it has an average bacteria count of 500 million per bottle. Suppose milk cannot be safely consumed if the bacteria count is greater than 3 billion per bottle. Under this model, how many days would the milk be safe to drink once it has been bottled?
(Short Answer)
4.9/5  (31)
(31)
A standard cup of coffee contains about 100 mg of caffeine, and caffeine leaves the body at a rate of about 17% an hour. How many mg of caffeine are left in the body after 6 hours if this rate is hourly? Round to 2 decimal places.
(Short Answer)
4.8/5  (33)
(33)
A cigarette contains about 0.4 mg of nicotine. The half-life of nicotine in the body is about 2 hours. How many hours does it take, after smoking a cigarette, for the level of nicotine in a smoker's body to be reduced to 0.1 mg? Round to 2 decimal places.
(Short Answer)
4.9/5  (42)
(42)
The distance 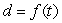 in feet that a golf ball will fall in t seconds if dropped from a very high tower is given by the formula
in feet that a golf ball will fall in t seconds if dropped from a very high tower is given by the formula  . Make and label a table or a graph of values of f(t) giving distances fallen for the time period
. Make and label a table or a graph of values of f(t) giving distances fallen for the time period 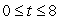 . Using your table or graph, the change in the height of the golf ball between times t = 5 and t = 7 is _____ feet, and the average rate of change in the height of the golf ball between times t = 5 and t = 7 is____ feet per second.
. Using your table or graph, the change in the height of the golf ball between times t = 5 and t = 7 is _____ feet, and the average rate of change in the height of the golf ball between times t = 5 and t = 7 is____ feet per second.
(Short Answer)
4.9/5  (34)
(34)
A teenager has $36 to spend at a carnival on both food and rides. Food costs (on average) $4 per item, and rides cost (on average) $2 each. Let f be the number of food items purchased and r be the number of rides purchased. What is the equation of the teenager's budget constraint?
(Multiple Choice)
4.9/5  (28)
(28)
An exponentially decaying substance was weighed every hour and the results are given below. What is the half-life of the substance? Round to the nearest tenth. 
(Short Answer)
4.8/5  (27)
(27)
A ball is thrown at time t = 0 and its height above ground t seconds after it is thrown is given by 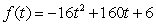 feet. Use a graphing calculator to graph
feet. Use a graphing calculator to graph 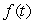 , and use the graph to answer the following.
A. How high does the ball go?
B. How long is it in the air?
, and use the graph to answer the following.
A. How high does the ball go?
B. How long is it in the air?
(Short Answer)
4.9/5  (27)
(27)
A company has cost and revenue functions given in dollars by 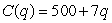 and
and 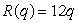 . What is the effect on the break even point of doubling the fixed costs?
. What is the effect on the break even point of doubling the fixed costs?
(Multiple Choice)
4.9/5  (36)
(36)
The graph of 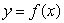 is shown in the following figure. Estimate
is shown in the following figure. Estimate 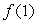 (to the nearest integer).
(to the nearest integer). 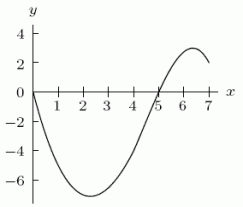
(Short Answer)
4.8/5  (37)
(37)
The size of a bird population on an island can be described by a sinusoidal graph. The number of birds on the island decreased from a maximum of 20,000 in 1943 to a minimum of 12,000 in 1989, and then began increasing again. Find a function that describes this behavior and use it to estimate the number of birds on the island in 2012. Round to the nearest whole number.
(Short Answer)
4.9/5  (32)
(32)
Suppose that 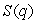 is the price per unit (in dollars) of widgets which will induce producers to supply q thousand widgets to the market, and suppose that
is the price per unit (in dollars) of widgets which will induce producers to supply q thousand widgets to the market, and suppose that 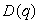 is the price per unit at which consumers will buy q thousand units. If
is the price per unit at which consumers will buy q thousand units. If 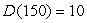 and
and  , what do you predict about the future selling price of widgets (currently at $10)?
, what do you predict about the future selling price of widgets (currently at $10)?
(Multiple Choice)
4.8/5  (25)
(25)
Consider the functions 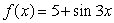 and
and 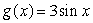 . Which has a larger vertical intercept?
. Which has a larger vertical intercept?
(Multiple Choice)
4.9/5  (44)
(44)
The number of bacteria in milk grows at a rate of 11% per day once the milk has been bottled. When milk is put in the bottles, it has an average bacteria count of 500 million per bottle.
A. Write an equation for 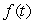 , the number of bacteria t days after the milk was bottled.
B. Graph
, the number of bacteria t days after the milk was bottled.
B. Graph 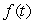 . Label the axes and intercepts.
. Label the axes and intercepts.
(Short Answer)
4.9/5  (26)
(26)
Temperatures in a room oscillate between the low of 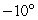 F (at 5am) and the high of
F (at 5am) and the high of 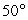 F (reached at 5pm). If
F (reached at 5pm). If  is a formula for the temperature in the room in terms of time from 5am, then A =_____, B =_____, and C =_____.
is a formula for the temperature in the room in terms of time from 5am, then A =_____, B =_____, and C =_____.
(Short Answer)
4.8/5  (32)
(32)
Showing 101 - 120 of 204
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)