Exam 7: Applications of Integration
Exam 1: Preparation for Calculus125 Questions
Exam 2: Limits and Their Properties85 Questions
Exam 3: Differentiation193 Questions
Exam 4: Applications of Differentiation154 Questions
Exam 5: Integration184 Questions
Exam 6: Differential Equations93 Questions
Exam 7: Applications of Integration119 Questions
Exam 8: Integration Techniques and Improper Integrals130 Questions
Exam 9: Infinite Series181 Questions
Exam 10: Conics, Parametric Equations, and Polar Coordinates114 Questions
Exam 11: Vectors and the Geometry of Space130 Questions
Exam 12: Vector-Valued Functions85 Questions
Exam 13: Functions of Several Variables173 Questions
Exam 14: Multiple Integration143 Questions
Exam 15: Vector Anal142 Questions
Select questions type
Use the disk or shell method to find the volume of the solid generated by revolving the region in the first quadrant bounded by the graph of the equation about the given line. 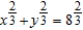 (i) the x-axis; (ii) the y-axis
(i) the x-axis; (ii) the y-axis
(Multiple Choice)
4.9/5  (26)
(26)
Neglecting air resistance and the weight of the propellant, determine the work done in propelling a four-ton satellite to a height of 400 miles above earth. Assume that Earth has a radius of 4000 miles.
(Multiple Choice)
4.9/5  (31)
(31)
A 22-foot chain that weighs 2 pounds per foot hangs from a winch 22 feet above ground level. Find the work done by the winch in winding up the entire chain.
(Multiple Choice)
4.8/5  (30)
(30)
Use the disk or the shell method to find the volume of the solid generated by revolving the region bounded by the graphs of the equations 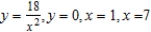 about the x-axis. Round your answer to two decimal places.
about the x-axis. Round your answer to two decimal places.
(Multiple Choice)
4.7/5  (31)
(31)
Use the shell method to find the volume of the solid generated by revolving the plane region bounded by 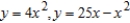 , about the line
, about the line  .
.
(Multiple Choice)
4.9/5  (39)
(39)
Use the disk or shell method to find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the line 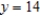 .
. 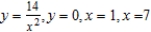
(Multiple Choice)
4.8/5  (35)
(35)
Use the Theorem of Pappus to find the volume of the solid formed by revolving the region bounded by the graphs of 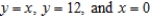 about the x-axis. Round your answer to two decimal places.
about the x-axis. Round your answer to two decimal places.
(Multiple Choice)
4.8/5  (38)
(38)
Find 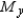 for the lamina of uniform density
for the lamina of uniform density 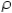 bounded by the graphs of the equations
bounded by the graphs of the equations 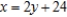 and
and 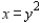 .
.
(Multiple Choice)
4.7/5  (33)
(33)
Find the center of mass of the point masses lying on the x-axis. 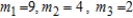

(Multiple Choice)
4.8/5  (28)
(28)
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the given lines. 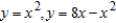
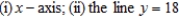
(Multiple Choice)
4.8/5  (43)
(43)
Set up and evaluate the definite integral for the area of the surface formed by revolving the graph of 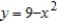 about the y-axis. Round your answer to three decimal places.
about the y-axis. Round your answer to three decimal places. 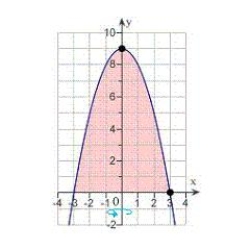
(Multiple Choice)
4.8/5  (32)
(32)
Find the area of the surface generated by revolving the curve about the y-axis.  .
.
(Multiple Choice)
4.8/5  (34)
(34)
Set up and evaluate the integral that gives the volume of the solid formed by revolving the region bounded by  and
and 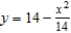 about the
about the  -axis.
-axis.
(Multiple Choice)
4.9/5  (32)
(32)
Find the arc length of the graph of the function 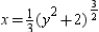 over the interval
over the interval 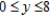 .
.
(Multiple Choice)
4.8/5  (28)
(28)
Find  for the lamina of uniform density
for the lamina of uniform density 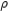 bounded by the graphs of the equations
bounded by the graphs of the equations  .
.
(Multiple Choice)
4.7/5  (30)
(30)
A quantity of gas with an initial volume of 5 cubic feet and a pressure of 1700 pounds per square foot expands to a volume of 9 cubic feet. Find the work done by the gas for the given volume and pressure. Round your answer to two decimal places. Assume the temperature of the gas in this process remain constant.
(Multiple Choice)
4.8/5  (38)
(38)
Find the volume of the solid generated by revolving the region bounded by the graphs of the equations about the line 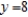 .
. 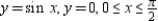
(Multiple Choice)
4.7/5  (33)
(33)
The vertical cross section of an irrigation canal is modeled by 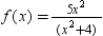 , where x is measured in feet and
, where x is measured in feet and 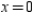 corresponds to the center of the canal. Use the integration capabilities of a graphing utility to approximate the fluid force against a vertical gate used to stop the flow of water if the water is
corresponds to the center of the canal. Use the integration capabilities of a graphing utility to approximate the fluid force against a vertical gate used to stop the flow of water if the water is  feet deep. Round your answer to three decimal places.
feet deep. Round your answer to three decimal places.
(Multiple Choice)
4.7/5  (34)
(34)
Find the fluid force of a square vertical plate submerged in water, where 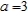 meters and the weight-density of water is 9800 newtons per cubic meter.
meters and the weight-density of water is 9800 newtons per cubic meter. 
(Multiple Choice)
4.9/5  (38)
(38)
Use the shell method to set up and evaluate the integral that gives the volume of the solid generated by revolving the plane region about the y-axis. 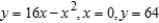
(Multiple Choice)
4.8/5  (27)
(27)
Showing 21 - 40 of 119
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)