Exam 7: Applications of Integration
Exam 1: Preparation for Calculus125 Questions
Exam 2: Limits and Their Properties85 Questions
Exam 3: Differentiation193 Questions
Exam 4: Applications of Differentiation154 Questions
Exam 5: Integration184 Questions
Exam 6: Differential Equations93 Questions
Exam 7: Applications of Integration119 Questions
Exam 8: Integration Techniques and Improper Integrals130 Questions
Exam 9: Infinite Series181 Questions
Exam 10: Conics, Parametric Equations, and Polar Coordinates114 Questions
Exam 11: Vectors and the Geometry of Space130 Questions
Exam 12: Vector-Valued Functions85 Questions
Exam 13: Functions of Several Variables173 Questions
Exam 14: Multiple Integration143 Questions
Exam 15: Vector Anal142 Questions
Select questions type
Set up and evaluate the integral that gives the volume of the solid formed by revolving the region bounded by 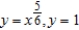 , and
, and 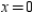 about the
about the 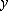 -axis.
-axis.
(Multiple Choice)
4.8/5  (29)
(29)
Use the shell method to find the volume of the solid generated by revolving the plane region about the line 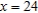 .
. 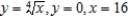
(Multiple Choice)
5.0/5  (30)
(30)
Electrical wires suspended between two towers form a catenary modeled by the equation 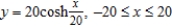 where x and y are measured in meters. The towers are 40 meters apart. Find the length of the suspended cable. Round your answer to three decimal places.
where x and y are measured in meters. The towers are 40 meters apart. Find the length of the suspended cable. Round your answer to three decimal places. 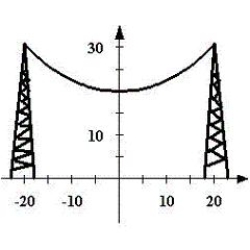
(Multiple Choice)
4.8/5  (26)
(26)
A force of 255 Newtons stretches a spring 20 centimeters. How much work is done in stretching the spring from 10 centimeters to 40 centimeters?
(Multiple Choice)
4.9/5  (26)
(26)
Set up and evaluate the definite integral for the area of the surface formed by revolving the graph of  about the x-axis.
about the x-axis.
(Multiple Choice)
4.7/5  (37)
(37)
The chief financial officer of a company reports that profits for the past fiscal year were $17.8 million. The officer predicts that profits for the next 8 years will grow at a continuous annual rate somewhere between 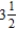 % and 8%. Estimate the cumulative difference in total profit over the 8 years based on the predicted range of growth rates. Round your answer to three decimal places.
% and 8%. Estimate the cumulative difference in total profit over the 8 years based on the predicted range of growth rates. Round your answer to three decimal places.
(Multiple Choice)
4.8/5  (35)
(35)
Find the fluid force on the vertical side of the tank, where the dimensions are given in feet. Assume that the tank is full of water. Note: The density of water is 62.4 lbs per cubic foot. 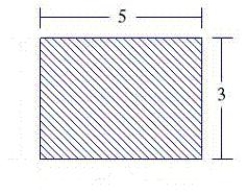
(Multiple Choice)
4.8/5  (42)
(42)
Find the center of mass of the given system of point masses.

(Multiple Choice)
4.8/5  (32)
(32)
Consider a beam of length L = 12 feet with a fulcrum x feet from one end as shown in the figure. Two objects weighing 36 pounds and 108 pounds are placed at opposite ends of the beam. Find x (the distance between the fulcrum and the object weighing 36 pounds) such that the system is equilibrium. 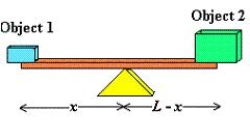
(Multiple Choice)
4.9/5  (33)
(33)
Find the buoyant force of a rectangular solid of the given dimensions submerged in water so that the top side is parallel to the surface of the water. The buoyant force is the difference between the fluid forces on the top and bottom sides of the solid. Round your answer to two decimal places. 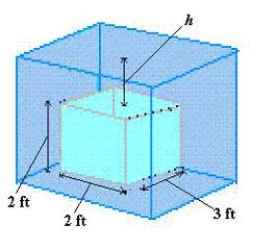
(Multiple Choice)
5.0/5  (34)
(34)
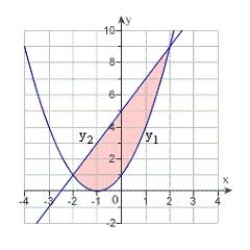
Set up the definite integral that gives the area of the region bounded by the graph of 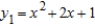 and
and 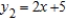 .
. 
(Multiple Choice)
4.9/5  (37)
(37)
Find the area of the surface generated by revolving the curve about the x-axis. 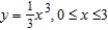 .
.
(Multiple Choice)
4.9/5  (33)
(33)
A cylindrical gasoline tank is placed so that the axis of the cylinder is horizontal. Find the fluid force on a circular end of the tank if the tank is full, assuming that the diameter is 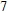 feet and the gasoline weighs
feet and the gasoline weighs  pounds per cubic foot. (Evaluate one integral by a geometric formula and the other by observing that the integrand is an odd function.) Round your answer to two decimal places.
pounds per cubic foot. (Evaluate one integral by a geometric formula and the other by observing that the integrand is an odd function.) Round your answer to two decimal places.
(Multiple Choice)
5.0/5  (34)
(34)
Find 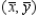 for the lamina of uniform density
for the lamina of uniform density 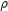 bounded by the graphs of the equations
bounded by the graphs of the equations 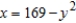 and
and 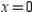 .
.
(Multiple Choice)
4.8/5  (27)
(27)
Find the area of the region bounded by the graphs of the algebraic functions. 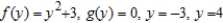
(Multiple Choice)
4.8/5  (38)
(38)
Find the volume of the solid generated by rotating the circle 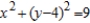 about the x-axis.
about the x-axis.
(Multiple Choice)
4.9/5  (34)
(34)
Find  ,
, 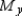 , and
, and 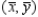 for the lamina of uniform density
for the lamina of uniform density 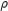 bounded by the graphs of the equations
bounded by the graphs of the equations 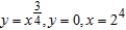 .
.
(Multiple Choice)
4.8/5  (35)
(35)
Concrete sections for the new building have the dimensions (in meters) and shape as shown in the figure (the picture is not necessarily drawn to scale). One cubic meter of concrete weighs 5280 pounds. Find the weight of the section. Round your answer to the nearest pound. 
(Multiple Choice)
4.9/5  (34)
(34)
Find the area of the region bounded by the equations by integrating (i) with respect to x and (ii) with respect to y. 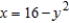
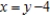
(Multiple Choice)
4.8/5  (30)
(30)
Find the arc length of the graph of the function 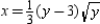 over the interval
over the interval  .
.
(Multiple Choice)
5.0/5  (37)
(37)
Showing 61 - 80 of 119
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)