Exam 3: Exponential and Logarithmic Functions
Exam 1: Functions and Their Graphs118 Questions
Exam 2: Polynomial and Rational Functions120 Questions
Exam 3: Exponential and Logarithmic Functions120 Questions
Exam 4: Trigonometric Functions118 Questions
Exam 5: Analytic Trigonometry120 Questions
Exam 6: Additional Topics in Trigonometry120 Questions
Exam 7: Linear Systems and Matrices120 Questions
Exam 8: Sequences, Series, and Probability119 Questions
Exam 9: Topics in Analytic Geometry120 Questions
Exam 10: Analytic Geometry in Three Dimensions120 Questions
Exam 11: Limits and an Introduction to Calculus40 Questions
Exam 12: Review of Graphs, Equations, and Inequalities99 Questions
Select questions type
Solve the logarithmic equation below algebraically. Round your result to three decimal places.
(Multiple Choice)
4.9/5  (37)
(37)
Condense the expression to the logarithm of a single term.
(Multiple Choice)
4.8/5  (38)
(38)
What is the value of the function at Round to 3 decimal places.
(Multiple Choice)
4.8/5  (36)
(36)
Determine whether the scatter plot below could best be modeled by a linear model, a quadratic model, an exponential model, a logarithmic model, or a logistic model. 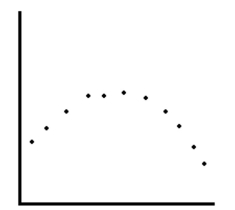
(Multiple Choice)
4.8/5  (37)
(37)
Solve the logarithmic equation below algebraically. Round your result to three decimal places.
(Multiple Choice)
4.9/5  (37)
(37)
Evaluate the function . Round to 3 decimal places. (You may use your calculator.)
(Multiple Choice)
4.9/5  (37)
(37)
Evaluate the logarithm using the change of base formula. Round to 3 decimal places.
(Multiple Choice)
4.9/5  (34)
(34)
Use the properties of logarithms to expand the expression as a sum, difference, and/or constant multiple of logarithms. (Assume all variables are positive.)
(Multiple Choice)
4.9/5  (33)
(33)
Solve the exponential equation below algebraically. Round your result to three decimal places.
(Multiple Choice)
4.8/5  (25)
(25)
Condense the expression below to the logarithm of a single quantity.
(Multiple Choice)
4.7/5  (40)
(40)
Showing 101 - 120 of 120
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)