Exam 6: Eigenvalues and Eigenvectors
Exam 1: Systems of Linear Equations57 Questions
Exam 2: Euclidean Space48 Questions
Exam 3: Matrices76 Questions
Exam 4: Subspaces60 Questions
Exam 5: Determinants48 Questions
Exam 6: Eigenvalues and Eigenvectors75 Questions
Exam 7: Vector Spaces45 Questions
Exam 8: Orthogonality75 Questions
Exam 9: Linear Transformations60 Questions
Exam 10: Inner Product Spaces45 Questions
Exam 11: Additional Topics and Applications75 Questions
Select questions type
Compute the first three iterations of the Power Method without scaling, starting with the given 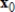 , where
, where  .
.
(Essay)
4.8/5  (26)
(26)
Find the characteristic polynomial, the eigenvalues, and a basis for each eigenspace for the matrix A = 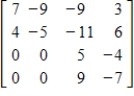 .
.
(Essay)
4.9/5  (39)
(39)
Find the solution for the system that satisfies the condition at t = 0.
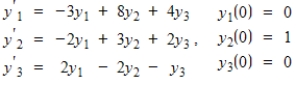
(Essay)
4.8/5  (32)
(32)
If A is a real square matrix with complex eigenvalue  and associated eigenvector
and associated eigenvector  , then
, then 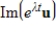 is a real solution to the system
is a real solution to the system 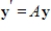 .
.
(True/False)
4.8/5  (39)
(39)
If A is a real matrix and 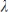 is an eigenvalue of A with
is an eigenvalue of A with  and corresponding eigenvector u, then
and corresponding eigenvector u, then 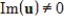 .
.
(True/False)
4.8/5  (34)
(34)
The dominant eigenvalue of the matrix A given below is 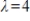 . Compute the first two iterations of the Shifted Power Method with scaling, starting with the given
. Compute the first two iterations of the Shifted Power Method with scaling, starting with the given 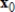 , to determine the eigenvalue farthest from
, to determine the eigenvalue farthest from  , rounding any numerical values to two decimal places.
, rounding any numerical values to two decimal places.

(Essay)
4.8/5  (31)
(31)
Find the characteristic polynomial, the eigenvalues, and a basis for each eigenspace for the matrix A = 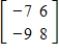 .
.
(Essay)
4.8/5  (36)
(36)
The coefficient matrix for a system of linear differential equations of the form 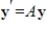 has the given eigenvalues and eigenspace bases. Find the general solution for the system.
has the given eigenvalues and eigenspace bases. Find the general solution for the system.
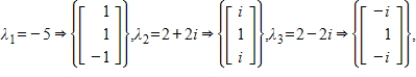
(Essay)
4.9/5  (31)
(31)
If u and v are both eigenvectors of an n ×n matrix A, then u+v is also an eigenvector of the A.
(True/False)
4.8/5  (29)
(29)
Compute the first two iterations of the Inverse Power Method, starting with the given 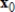 , rounding any numerical values to two decimal places.
, rounding any numerical values to two decimal places.
(Essay)
4.7/5  (30)
(30)
If A is a real matrix, and 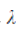 is a complex eigenvalue of A, then
is a complex eigenvalue of A, then 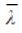 is also an eigenvalue of A.
is also an eigenvalue of A.
(True/False)
4.9/5  (34)
(34)
Use the Power Method with scaling to determine an eigenvalue and associated eigenvector of A, starting with the given 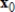 .
.
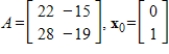
(Essay)
4.9/5  (25)
(25)
Find the eigenvalues and a basis for each eigenspace for the given matrix.

(Essay)
4.8/5  (35)
(35)
Showing 61 - 75 of 75
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)