Exam 1: Systems of Linear Equations
Exam 1: Systems of Linear Equations57 Questions
Exam 2: Euclidean Space48 Questions
Exam 3: Matrices76 Questions
Exam 4: Subspaces60 Questions
Exam 5: Determinants48 Questions
Exam 6: Eigenvalues and Eigenvectors75 Questions
Exam 7: Vector Spaces45 Questions
Exam 8: Orthogonality75 Questions
Exam 9: Linear Transformations60 Questions
Exam 10: Inner Product Spaces45 Questions
Exam 11: Additional Topics and Applications75 Questions
Select questions type
A linear system with infinitely many solutions must have more variables than equations.
(True/False)
4.9/5  (29)
(29)
Determine if the system is diagonally dominant. If not, then if possible rewrite the system so that it is diagonally dominant.
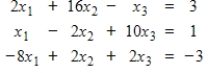
(Short Answer)
4.7/5  (31)
(31)
The values for the first few Gauss-Seidel iterations for a linear system are given. Find the values for the next iteration.

(Essay)
4.8/5  (34)
(34)
If a linear system has infinitely many solutions, then there are more variables than equations.
(True/False)
4.9/5  (31)
(31)
Use partial pivoting with Gaussian elimination to find the solutions to the system.
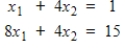
(Essay)
4.7/5  (37)
(37)
The points (-6, 0, -1), (3, 2, 0), and (0, 3, -1) lie on a plane ax + by + cz = 1. Use a system of linear equations to find the equation of this plane.
(Essay)
4.8/5  (33)
(33)
Use a system of linear equations to find the values
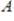 ,
,
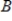 , and
, and
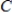 for the partial fraction decomposition
for the partial fraction decomposition
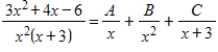
(Essay)
4.8/5  (36)
(36)
Compute the first four Gauss-Seidel iterations for the system in question 11, with the initial value of each variable set equal to
 . Then rewrite the system so that it is diagonally dominant, set the value of each variable to 0, and again compute four Gauss-Seidel iterations.
. Then rewrite the system so that it is diagonally dominant, set the value of each variable to 0, and again compute four Gauss-Seidel iterations.
(Essay)
4.9/5  (35)
(35)
Convert the given system to an augmented matrix, and find all solutions by transforming to reduced echelon form and using back substitution.

(Essay)
4.9/5  (32)
(32)
Reorder the equations to put the following system of three equations with four unknowns in echelon form:
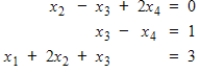
(Essay)
4.8/5  (35)
(35)
Convert the given system to an augmented matrix, and find all solutions by reducing to echelon form and using back substitution.
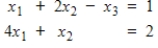
(Essay)
4.7/5  (39)
(39)
The values for the first few Jacobi iterations for a linear system are given. Find the values for the next iteration.
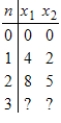
(Essay)
5.0/5  (39)
(39)
Use a system of linear equations to find the values of the coefficients a, b, c if
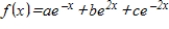 with
with
 ,
,
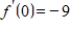 , and
, and
 .
.
(Essay)
4.8/5  (33)
(33)
Use a system of linear equations to find the equation
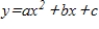 of the parabola which passes through the points (1, 2), (2, 0), and (3, -4).
of the parabola which passes through the points (1, 2), (2, 0), and (3, -4).
(Essay)
4.9/5  (34)
(34)
Suppose a system of
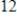 equations has two free variables. How many leading variables are there?
equations has two free variables. How many leading variables are there?
(Short Answer)
4.7/5  (27)
(27)
If a linear system has no free variables, then there exists at most one solution.
(True/False)
4.9/5  (30)
(30)
Compute the first three Jacobi iterations, using
 as the initial value for each variable. Then find the exact solution and compare.
as the initial value for each variable. Then find the exact solution and compare.
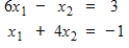
(Essay)
4.9/5  (36)
(36)
Convert the given system to an augmented matrix, and find all solutions by transforming to reduced echelon form and using back substitution.
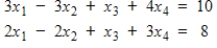
(Essay)
5.0/5  (29)
(29)
Identify the row operation which transforms the matrix on the left to the matrix on the right.

(Essay)
4.9/5  (28)
(28)
Showing 21 - 40 of 57
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)