Exam 10: Managing Customer and Work Flows

Leyland Motors can service an average 5 cars per hour, and the owner of Leyland Motors wants to know the probability of various customer arrival rates. Given the average arrival rate of 4 customers per hour, the owner uses the Poisson distribution to calculate the probabilities of various customer arrivals per hour. -What is the probability that at least 2 of the customers will arrive within 1 hour?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability that at least 4 customers will arrive in 1 hour, when the mean arrival rate is 3 customers per hour, and interarrival times are exponentially distributed?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability that less than 4 customers will arrive in 1 hour, when the mean arrival rate is 3 customers per hour, and interarrival times are exponentially distributed?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability that exactly 4 customers will arrive in 1 hour, when the mean arrival rate is 3 customers per hour, and interarrival times are exponentially distributed?

Leyland Motors can service an average 5 cars per hour, and the owner of Leyland Motors wants to know the probability of various customer arrival rates. Given the average arrival rate of 4 customers per hour, the owner uses the Poisson distribution to calculate the probabilities of various customer arrivals per hour. -What is the probability that there will be more than 5 customers arriving within 1 hour?

Use the following information to determine the optimal overbooking policy for a spa center at downtown Marquette. The center's capacity is 12 guests. The historic number of no-shows for a typical day, along with the probability of occurrence, is shown in the following table. The average profitability per guest is $80, and the cost of lost goodwill per guest due to overbooking is approximately $40. 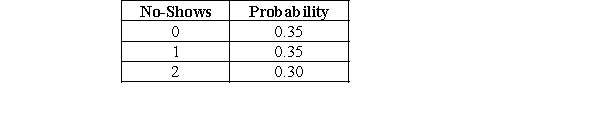-Calculate the total expected profit with 14 reservations (which means 2 overbookings).

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability of having exactly 12 customers arriving within 1 hour?

Use the following information to determine the optimal overbooking policy for a spa center at downtown Marquette. The center's capacity is 12 guests. The historic number of no-shows for a typical day, along with the probability of occurrence, is shown in the following table. The average profitability per guest is $80, and the cost of lost goodwill per guest due to overbooking is approximately $40. -Calculate the total expected profit with 12 reservations (and no overbooking).

Leyland Motors can service an average 5 cars per hour, and the owner of Leyland Motors wants to know the probability of various customer arrival rates. Given the average arrival rate of 4 customers per hour, the owner uses the Poisson distribution to calculate the probabilities of various customer arrivals per hour. -What is the probability that, at most, 3 of the customers will arrive within 1 hour?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability of having exactly 4 customers arriving within 1 hour?

Leyland Motors can service an average 5 cars per hour, and the owner of Leyland Motors wants to know the probability of various customer arrival rates. Given the average arrival rate of 4 customers per hour, the owner uses the Poisson distribution to calculate the probabilities of various customer arrivals per hour. -What is the probability that none of the customers will arrive within 1 hour?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability that less than 2 customers will arrive in 1 hour, when the mean arrival rate is 2 customers per hour, and interarrival times are exponentially distributed?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability that more than 10 customers will arrive in 1 hour, when the mean arrival rate is 15 customers per hour, and interarrival times are exponentially distributed?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability of having none of the customers arriving within 1 hour?

A street noodle vendor (one server) in Singapore can service an average of 20 customers per hour. Given an average arrival rate of 12 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -Calculate the mean number of customers in the system (being served and in the queue).

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability that at least 10 customers will arrive in 1 hour, when the mean arrival rate is 15 customers per hour, and interarrival times are exponentially distributed?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability of having more than 12 customers arriving within 1 hour?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability of having at least 10 customers arriving within 1 hour?

A street noodle vendor in Singapore can service an average of 10 customers per hour. Given an average arrival rate of 8 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -What is the probability of having more than 10 customers arriving within 1 hour?

A street noodle vendor (one server) in Singapore can service an average of 20 customers per hour. Given an average arrival rate of 12 customers per hour, use the Poisson distribution to calculate the probability that the vendor can handle the demand. -Change the arrival rate of from 12 customers per hour to 15 customers per hour. What is the probability that there is more than 1 customer in the system at any given time?