Exam 4: Rational, Power, and Root Functions
Exam 1: Linear Functions, Equations, and Inequalities44 Questions
Exam 2: Analysis of Graphs of Functions84 Questions
Exam 3: Polynomial Functions40 Questions
Exam 4: Rational, Power, and Root Functions48 Questions
Exam 5: Inverse, Exponential, and Logarithmic Functions84 Questions
Exam 6: Systems and Matrices68 Questions
Exam 7: Analytic Geometry and Nonlinear Systems48 Questions
Exam 8: The Unit Circle and Functions of Trigonometry88 Questions
Exam 9: Trigonometric Identities and Equations100 Questions
Exam 10: Applications of Trigonometry and Vectors40 Questions
Exam 11: Further Topics in Algebra48 Questions
Exam 12: Limits, Derivatives, and Definite Integrals100 Questions
Exam 13: Reference: Basic Algebraic Concepts40 Questions
Select questions type
Graph the function in the standard viewing window. Then do each of the following:
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.
Free
(Essay)
4.8/5  (31)
(31)
Correct Answer:
 (a)
(a)
(b)
(c) increases
(d)
(e)
Consider the rational function defined by .
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.
Free
(Essay)
4.8/5  (37)
(37)
Correct Answer:
(a) -2
(b)

(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
Free
(Essay)
4.7/5  (38)
(38)
Correct Answer:
(a)
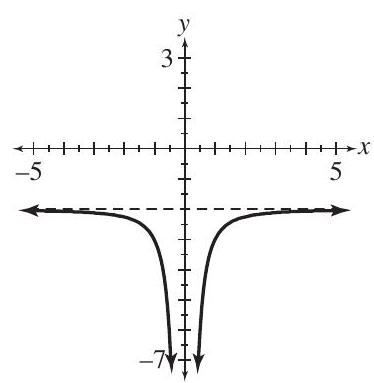
(b) The graph of is reflected across the -axis and shifted 2 units downward.
(c)
Graph the function in the standard viewing window. Then do each of the following:
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.
(Essay)
4.8/5  (33)
(33)
(a) Solve the following rational equation analytically: .
(b) Use the results of part (a) and a graph to find the solution set of .
(Short Answer)
4.8/5  (28)
(28)
Consider the rational function defined by . Determine the answers to (a) - (e) analytically:
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .
(Essay)
4.8/5  (42)
(42)
(a) Solve the equation analytically. Support the solution(s) with a graph.
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .
(Essay)
4.9/5  (38)
(38)
Find the equation of the oblique asymptote of the graph of the rational function defined by . Then graph the function and its asymptote using a graphing calculator to illustrate an accurate comprehensive graph.
(Essay)
4.8/5  (34)
(34)
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(Essay)
4.9/5  (36)
(36)
Find the equation of the oblique asymptote of the graph of the rational function defined by . Then graph the function and its asymptote using a graphing calculator to illustrate an accurate comprehensive graph.
(Essay)
4.7/5  (39)
(39)
Consider the rational function defined by . Determine the answers to (a) - (e) analytically:
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .
(Essay)
4.9/5  (34)
(34)
(a) Solve the equation analytically. Support the solution(s) with a graph.
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .
(Essay)
4.9/5  (35)
(35)
Consider the rational function defined by . Determine the answers to (a) - (e) analytically:
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .
(Essay)
4.8/5  (33)
(33)
A manufacturer needs to construct a box with a lid for a special product. The only stipulations are that the volume of the box should be 2000 cubic centimeters and that the box should have a square base. The cost for producing such a box has been determined to be represented by the function , where is the cost of the box in dollars and is the length of a side of the base in centimeters. Use the graph of to determine the side length that will minimize the cost of the box, and determine what this cost will be.
(Hint: Use the window by .)
(Essay)
4.8/5  (24)
(24)
Find the equation of the oblique asymptote of the graph of the rational function defined by . Then graph the function and its asymptote using a graphing calculator to illustrate an accurate comprehensive graph.
(Essay)
4.8/5  (48)
(48)
A manufacturer needs to construct a box with a lid for a special product. The only stipulations are that the volume of the box should be 3000 cubic centimeters and that the box should have a square base. The cost for producing such a box has been determined to be represented by the function , where is the cost of the box in dollars and is the length of a side of the base in centimeters. Use the graph of to determine the side length that will minimize the cost of the box, and determine what this cost will be.
(Hint: Use the window by .)
(Essay)
4.8/5  (39)
(39)
Consider the rational function defined by .
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.
(Essay)
4.7/5  (41)
(41)
The concession stand at a sporting event can fill at most 12 orders per minute. If people arrive randomly at an average rate of people per minute, then the average wait in minutes before an order is filled is approximated by where .
(a) Evaluate , and . Interpret the results.
(b) Graph using the window by . Identify the vertical asymptote. What happens to as approaches 12 ?
(c) Find when the wait is 4 minutes.
(Essay)
4.9/5  (37)
(37)
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(Essay)
4.9/5  (29)
(29)
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(Essay)
4.9/5  (32)
(32)
Showing 1 - 20 of 48
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)