Exam 9: Conics, Systems of Nonlinear Equations and Inequalities, and Parametric Equations
Exam 1: Functions and Their Graphs137 Questions
Exam 2: Polynomial and Rational Functions141 Questions
Exam 3: Exponential and Logarithmic Functions137 Questions
Exam 4: Trigonometric Functions of Angles261 Questions
Exam 5: Trigonometric Functions of Real Numbers151 Questions
Exam 6: Analytic Trigonometry267 Questions
Exam 7: Vectors, the Complex Plane, and Polar Coordinates225 Questions
Exam 8: Systems of Linear Equations and Inequalities181 Questions
Exam 9: Conics, Systems of Nonlinear Equations and Inequalities, and Parametric Equations230 Questions
Exam 10: Sequences and Series124 Questions
Exam 11: Limits: A Preview to Calculus122 Questions
Exam 12: Review: Equations and Inequalities244 Questions
Select questions type
(a) Identify the type of conic by the discriminant, (b) transform the equation in x and y into an equation in X and Y (without an XY term) by rotating the x- and y-axes by an angle of θ to arrive at the new X- and Y-axes, and (c) graph the resulting equation (showing both sets of axes).
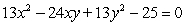
(Short Answer)
4.9/5  (31)
(31)
Find an equation for the parabola with focus (3, 12) and directrix y = 4.
(Multiple Choice)
4.8/5  (33)
(33)
Find the standard form of the equation of an ellipse with foci (0, -2) and (0, 2) and vertices (0, -5) and (0, 5).
(Multiple Choice)
4.9/5  (33)
(33)
Recall that the flight of a projectile can be modeled with the parametric equations:
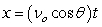 ,
, 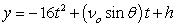 where t is in seconds, vo is the initial velocity, is the angle with the horizontal, and x and y are in feet.
A baseball is hit at an initial speed of 100 mph and angle of 32° at a height of 3 feet above the ground. If home plate is 465 feet from the back fence, which is 19 feet tall, will the baseball clear the back fence for a home run?
where t is in seconds, vo is the initial velocity, is the angle with the horizontal, and x and y are in feet.
A baseball is hit at an initial speed of 100 mph and angle of 32° at a height of 3 feet above the ground. If home plate is 465 feet from the back fence, which is 19 feet tall, will the baseball clear the back fence for a home run?
(Multiple Choice)
4.8/5  (44)
(44)
Find the points of intersection of the two parabolas.
Parabola I: vertex (1, -10); focus: (1, -9.142857)
 Parabola II: vertex (1, 11); focus: (1, 10.142857)
Parabola II: vertex (1, 11); focus: (1, 10.142857)

(Short Answer)
4.9/5  (35)
(35)
Find the polar equation that represents the conic described (assume the focus is at the origin).
Hyperbola with eccentricity e =
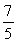 and directrix
and directrix
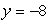
(Short Answer)
4.7/5  (37)
(37)
Identify the conic (parabola, ellipse, or hyperbola) that is represented by the equation.
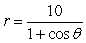
(Short Answer)
4.8/5  (33)
(33)
Find the standard form of the equation of a hyperbola with center (0, 0), transverse x-axis, and asymptotes
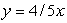 And
And
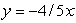
(Multiple Choice)
4.9/5  (38)
(38)
Recall that the flight of a projectile can be modeled with the parametric equations:
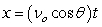 ,
, 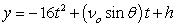 where t is in seconds, vo is the initial velocity, is the angle with the horizontal, and x and y are in feet.
A missile is fired from a ship at an angle of 39°, an initial height of 20 feet above the water's surface, and at a speed of 3890 feet per second. Will it be able to hit a target that is 2.4 miles away?
where t is in seconds, vo is the initial velocity, is the angle with the horizontal, and x and y are in feet.
A missile is fired from a ship at an angle of 39°, an initial height of 20 feet above the water's surface, and at a speed of 3890 feet per second. Will it be able to hit a target that is 2.4 miles away?
(Multiple Choice)
4.9/5  (27)
(27)
Find the standard form of the equation of a hyperbola with center (0, 0), transverse y-axis, and asymptotes y = -7x and y = 7x.
(Multiple Choice)
4.8/5  (44)
(44)
Find the standard form of the equation of an ellipse with foci (-3, 0) and (3, 0) and vertices (-4, 0) and (4, 0).
(Multiple Choice)
4.9/5  (40)
(40)
Solve the system of equations by applying the substitution method.
Y = x2 - 3
Y = 3x - 3
(Multiple Choice)
4.8/5  (36)
(36)
Recall that the flight of a projectile can be modeled with the parametric equations:
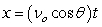 ,
, 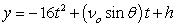 where t is in seconds, νo is the initial velocity, θ is the angle with the horizontal, and x and y are in feet.
A projectile is launched at a speed of 200 ft/sec at an angle of 30° with the horizontal. Plot the path of the projectile on a graph.
where t is in seconds, νo is the initial velocity, θ is the angle with the horizontal, and x and y are in feet.
A projectile is launched at a speed of 200 ft/sec at an angle of 30° with the horizontal. Plot the path of the projectile on a graph.
(Short Answer)
4.7/5  (30)
(30)
(a) Identify the type of conic by the discriminant, (b) transform the equation in x and y into an equation in X and Y (without an XY term) by rotating the x- and y-axes by an angle of θ to arrive at the new X- and Y-axes, and (c) graph the resulting equation (showing both sets of axes).

(Short Answer)
4.9/5  (34)
(34)
Showing 181 - 200 of 230
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)