Exam 4: Sensitivity Analysis and the Simplex Method
Exam 1: Introduction to Modeling and Decision Analysis51 Questions
Exam 2: Introduction to Optimization and Linear Programming62 Questions
Exam 3: Modeling and Solving Lp Problems in a Spreadsheet89 Questions
Exam 4: Sensitivity Analysis and the Simplex Method72 Questions
Exam 5: Network Modeling73 Questions
Exam 6: Integer Linear Programming73 Questions
Exam 7: Goal Programming and Multiple Objective Optimization62 Questions
Exam 8: Nonlinear Programming and Evolutionary Optimization69 Questions
Exam 9: Regression Analysis77 Questions
Exam 10: Discriminant Analysis60 Questions
Exam 11: Time Series Forecasting116 Questions
Exam 12: Introduction to Simulation Using Risk Solver Platform65 Questions
Exam 13: Queuing Theory80 Questions
Exam 14: Decision Analysis101 Questions
Exam 15: Project Management Online63 Questions
Select questions type
What are the objective function coefficients for X1 and X2 based on the following Risk Solver Platform (RSP) sensitivity output?
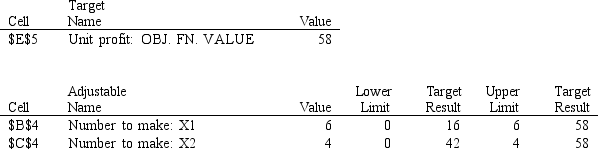
(Essay)
5.0/5  (32)
(32)
Consider the following linear programming model and Risk Solver Platform (RSP) sensitivity output. What is the optimal objective function value if the RHS of the first constraint increases to 18?
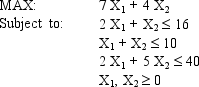
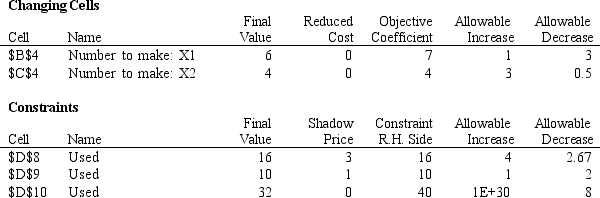
(Essay)
4.8/5  (30)
(30)
Is the optimal solution to this problem unique, or is there an alternate optimal solution? Explain your reasoning. 
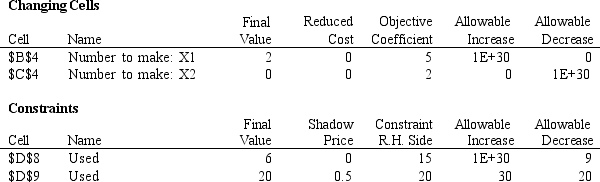
(Essay)
4.9/5  (40)
(40)
If the shadow price for a resource is 0 and 150 units of the resource are added what happens to the objective function value?
(Multiple Choice)
4.9/5  (37)
(37)
Use slack variables to rewrite this problem so that all its constraints are equality constraints.
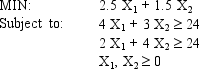
(Essay)
4.7/5  (26)
(26)
Solve this problem graphically. What is the optimal solution and what constraints are binding at the optimal solution?
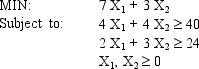
(Essay)
4.7/5  (38)
(38)
Solve this problem graphically. What is the optimal solution and what constraints are binding at the optimal solution?
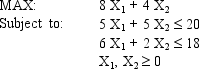
(Essay)
4.7/5  (36)
(36)
If the shadow price for a resource is 0 and 150 units of the resource are added what happens to the optimal solution?
(Multiple Choice)
4.9/5  (37)
(37)
When a solution is degenerate the shadow prices and their ranges
(Multiple Choice)
4.9/5  (34)
(34)
Why might a decision maker prefer a solution in the interior of the feasible region of a linear programming problem?
(Multiple Choice)
4.8/5  (32)
(32)
The optimization technique that locates solutions in the interior of the feasible region is known as _____?
(Multiple Choice)
4.9/5  (41)
(41)
Which of the constraints are binding at the optimal solution for the following problem and Risk Solver Platform (RSP) sensitivity output?
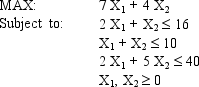
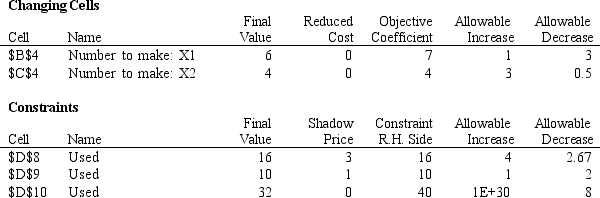
(Essay)
4.9/5  (32)
(32)
What is the smallest value of the objective function coefficient X1 can assume without changing the optimal solution?

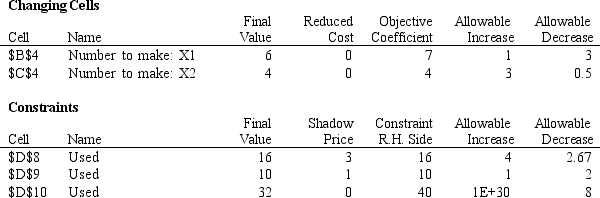
(Essay)
4.7/5  (36)
(36)
A change in the right hand side of a binding constraint may change all of the following except
(Multiple Choice)
4.8/5  (44)
(44)
The following questions are based on the problem below and accompanying Risk Solver Platform (RSP) sensitivity report.
Carlton construction is supplying building materials for a new mall construction project in Kansas. Their contract calls for a total of 250,000 tons of material to be delivered over a three-week period. Carlton's supply depot has access to three modes of transportation: a trucking fleet, railway delivery, and air cargo transport. Their contract calls for 120,000 tons delivered by the end of week one, 80% of the total delivered by the end of week two, and the entire amount delivered by the end of week three. Contracts in place with the transportation companies call for at least 45% of the total delivered be delivered by trucking, at least 40% of the total delivered be delivered by railway, and up to 15% of the total delivered be delivered by air cargo. Unfortunately, competing demands limit the availability of each mode of transportation each of the three weeks to the following levels (all in thousands of tons):
 The following is the LP model for this logistics problem.
The following is the LP model for this logistics problem.
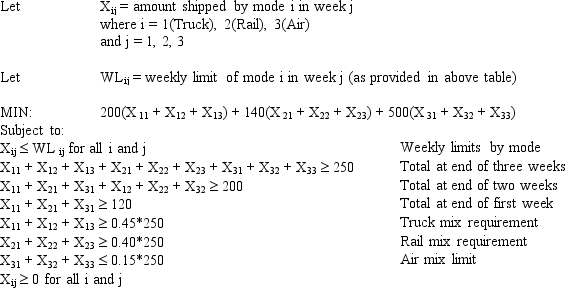
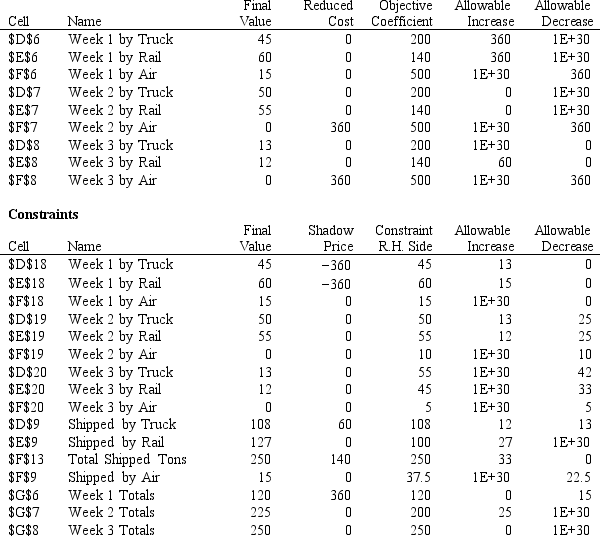 -Refer to Exhibit 4.1. Should the company negotiate for additional air delivery capacity?
-Refer to Exhibit 4.1. Should the company negotiate for additional air delivery capacity?
(Essay)
4.8/5  (32)
(32)
Constraint 3 is a non-binding constraint in the final solution to a maximization problem. Complete the following entry for the Risk Solver Platform (RSP) sensitivity report. Cell labels are included to ease of reference. 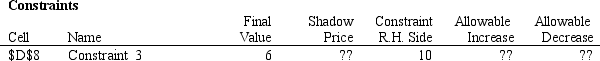
(Essay)
4.8/5  (32)
(32)
Showing 21 - 40 of 72
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)