Exam 10: Differential Equations
Exam 1: The Derivative189 Questions
Exam 2: Applications of the Derivative93 Questions
Exam 3: Techniques of Differentiation69 Questions
Exam 4: Logarithm Functions135 Questions
Exam 5: Applications of the Exponential and Natural Logarithm Functions73 Questions
Exam 6: The Definite Integral135 Questions
Exam 7: Functions of Several Variables119 Questions
Exam 8: The Trigonometric Functions128 Questions
Exam 9: Techniques of Integration178 Questions
Exam 10: Differential Equations126 Questions
Exam 11: Taylor Polynomials and Infinite Series132 Questions
Exam 12: Probability and Calculus92 Questions
Select questions type
Let t represent the number of hours that a packing machine is operated and y(t) represent the probability that the machine breaks down at least once during the t hours of operation. It has been observed that the rate of increase of the probability of a breakdown is proportional to the probability of not having a breakdown. Find a differential equation describing this situation.
Free
(Multiple Choice)
4.9/5  (32)
(32)
Correct Answer:
D
The following is a polygonal path obtained from Euler's method with n = 4 to approximate a solution f(t) of a differential equation. Indicate whether the following statements are true or false: 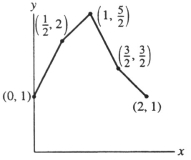 - ≈ 1
- ≈ 1
Free
(True/False)
4.9/5  (30)
(30)
Correct Answer:
True
Below is a sketch of f(x) = (x - 1) . 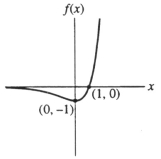 On a ty-coordinate system, sketch the solutions to the differential equation corresponding to the initial conditions , , and . Does the following graph represent this situation?
On a ty-coordinate system, sketch the solutions to the differential equation corresponding to the initial conditions , , and . Does the following graph represent this situation? 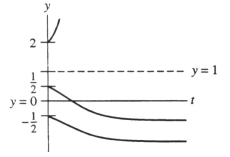
Free
(True/False)
4.8/5  (38)
(38)
Correct Answer:
True
One or more initial conditions are given for the differential equation. Use the qualitative theory of autonomous differential equations to sketch the graphs of the corresponding solution. Include a yz-graph as well as a ty-graph. Do these graphs represent the situation? 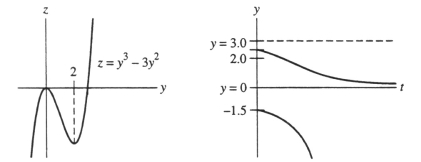
(True/False)
4.7/5  (34)
(34)
Solve the differential equation with the given initial condition.
-
(Multiple Choice)
4.7/5  (41)
(41)
Use Euler's method with n = 5 to approximate f(1) if y = f(t) satisfies the differential equation Compare this answer with the exact value of f(1). Is the following the correct answer?
Euler's method: f(1) ≈ 2.488; Actual value: f(t) = e ≈ 2.718
(True/False)
4.8/5  (31)
(31)
Solve the initial value problem using an integrating factor.
- + y = 3; y(0) = 0.
(Multiple Choice)
4.8/5  (38)
(38)
Write a differential equation that expresses the following description of a rate: When ice cream is removed from the freezer, it warms up at a rate proportional to the difference between the temperature of the ice cream and the room temperature of 76°. (Use y for the temperature of the ice cream, t for the time, and k for an unknown constant.)
(Multiple Choice)
4.8/5  (38)
(38)
Consider the differential equation = g(y) where g(y) is the function whose graph is shown below: 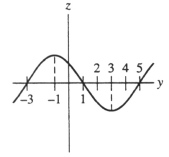 Indicate whether the following statements are true or false.
-If the initial value of y(0) is greater than 6, then the corresponding solution will be an increasing function.
Indicate whether the following statements are true or false.
-If the initial value of y(0) is greater than 6, then the corresponding solution will be an increasing function.
(True/False)
5.0/5  (38)
(38)
The birth rate in a certain city is 2% per year and the death rate is 2.5% per year. Also, there is a net movement of population into the city at the rate of 4000 people per year. Let N = f(t) be the city's population at time t. Write the differential equation satisfied by f(t). Does this equation accurately represent this situation:
(True/False)
4.8/5  (32)
(32)
Use Euler's method with n = 3 to approximate the solution f(t) to = 9t + , . Estimate f(1).
Enter just a reduced fraction of form .
(Short Answer)
4.8/5  (36)
(36)
Which of the following functions solves the differential equation: ?
(Multiple Choice)
4.8/5  (31)
(31)
The following is a polygonal path obtained from Euler's method with n = 4 to approximate a solution f(t) of a differential equation. Indicate whether the following statements are true or false:  -f(1) ≈
-f(1) ≈
(True/False)
4.8/5  (36)
(36)
Given the differential equation with the given initial condition: is this the solution
(True/False)
5.0/5  (38)
(38)
A man opens a savings account that earns interest at an annual rate of 6% compounded continuously. He plans to make continuous withdrawals at a rate of $300 per year. What will happen if his initial deposit is $5000? [Hint: Let f(x) be the savings account balance at time t, and determine the differential equation satisfied by f(t).]
(Multiple Choice)
4.8/5  (43)
(43)
Consider the differential equation = g(y) where g(y) is the function whose graph is shown below:  Indicate whether the following statements are true or false.
-For what y value(s) does a solution of = - 3y + 2 have inflection points?
Indicate whether the following statements are true or false.
-For what y value(s) does a solution of = - 3y + 2 have inflection points?
(Multiple Choice)
4.8/5  (32)
(32)
An investment earns 25% interest per year. Every year $10,000 is withdrawn in order to pay dividends to the investors. Set up a differential equation satisfied by f(t), the amount of money invested at time t. Does this equation accurately describe this situation:
(True/False)
4.9/5  (34)
(34)
The following is a polygonal path obtained from Euler's method with n = 4 to approximate a solution f(t) of a differential equation. Indicate whether the following statements are true or false:  - = 1
- = 1
(True/False)
4.9/5  (33)
(33)
Showing 1 - 20 of 126
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)