Exam 1: The Derivative
Exam 1: The Derivative189 Questions
Exam 2: Applications of the Derivative93 Questions
Exam 3: Techniques of Differentiation69 Questions
Exam 4: Logarithm Functions135 Questions
Exam 5: Applications of the Exponential and Natural Logarithm Functions73 Questions
Exam 6: The Definite Integral135 Questions
Exam 7: Functions of Several Variables119 Questions
Exam 8: The Trigonometric Functions128 Questions
Exam 9: Techniques of Integration178 Questions
Exam 10: Differential Equations126 Questions
Exam 11: Taylor Polynomials and Infinite Series132 Questions
Exam 12: Probability and Calculus92 Questions
Select questions type
Find the equation of the tangent line to the curve y = + 4 + 4 at (1, 9).
Enter your answer in standard slope-intercept form.
Free
(Short Answer)
4.9/5  (33)
(33)
Correct Answer:
y = 11x - 2
Write the word or phrase that best completes each statement or answers the question.
-f(x) = Enter your answer as just: a . No parentheses.
Free
(Short Answer)
4.8/5  (34)
(34)
Correct Answer:
Find the slope of the tangent line to the curve y = 2 + 4 at x = -1.
Enter just an integer.
Free
(Short Answer)
4.8/5  (36)
(36)
Correct Answer:
36
Compute the third derivative of the following function: f(z) = at z = .
Enter just a reduced fraction of form .
(Short Answer)
4.9/5  (29)
(29)
Let f(x) = . Compute (0) using limits.
Enter a reduced fraction or an integer.
(Short Answer)
4.7/5  (37)
(37)
Determine whether or not the following limit exists. If, so compute the limit.
(Multiple Choice)
4.9/5  (36)
(36)
Determine: if y = .
Enter your answer as just an integer or a reduced fraction.
(Short Answer)
4.9/5  (34)
(34)
Find the equation of the following line: Perpendicular to 3y - x = 1; y-intercept is .
Enter your answer in slope-intercept form.
(Short Answer)
4.8/5  (38)
(38)
A point P is moving along the x-axis. At any time t, the location of P on the x-axis is described by . Determine the point's instantaneous velocity when t = 5.
Enter just an integer.
(Short Answer)
4.8/5  (32)
(32)
The graph below shows the total sales (in thousands of dollars) in a supermarket during a typical 24-hour period. 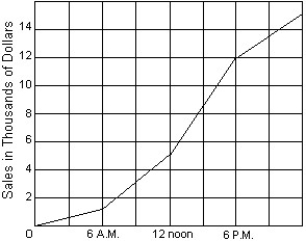 (i) Estimate the rate of sales during the period from 6 A.M. to 12 noon.
(ii) Which 6-hour interval sees the highest rate of sales and what is this rate?
(i) Estimate the rate of sales during the period from 6 A.M. to 12 noon.
(ii) Which 6-hour interval sees the highest rate of sales and what is this rate?
(Multiple Choice)
5.0/5  (42)
(42)
Find the slope of the graph of y = ( - 7 at x = 3.
Enter just an integer.
(Short Answer)
4.9/5  (35)
(35)
Find the second derivative of U = - at u = .
Enter just a real number.
(Short Answer)
4.8/5  (39)
(39)
Calculate the following limit(s) if they exist.
- ( - 2x + 5).
Enter just an integer or "does not exist".
(Short Answer)
4.7/5  (30)
(30)
Find the derivative of f(x) = .
Enter your answer exactly in the form: a
(Short Answer)
4.9/5  (37)
(37)
Write the word or phrase that best completes each statement or answers the question.
-y = Enter your answer exactly in the form: a where a, b are either fractions or integers. No parentheses. No labels.
(Short Answer)
5.0/5  (36)
(36)
Find the first derivative of z = 4t + (3 - at t = .
Enter just a fraction of form in lowest terms.
(Short Answer)
4.8/5  (26)
(26)
Find the equation of the following line: Perpendicular to - 8x + y - 3 = 0; (4, 3) on line.
(Multiple Choice)
4.9/5  (38)
(38)
A manufacturer's profit from producing x units of a product is given by What is the marginal profit when the production level is at 50 units? Enter your answer as a real number to two decimal places, no units.
(Short Answer)
4.8/5  (29)
(29)
Showing 1 - 20 of 189
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)