Exam 8: Calculus of Several Variables
Exam 1: Preliminaries205 Questions
Exam 2: Functions, Limits and the Derivative269 Questions
Exam 3: Differentiation330 Questions
Exam 4: Applications of the Derivative182 Questions
Exam 5: Exponential and Logarithmic Functions278 Questions
Exam 6: Integration314 Questions
Exam 7: Additional Topics in Integration250 Questions
Exam 8: Calculus of Several Variables206 Questions
Select questions type
Find the volume of the solid bounded above by the surface  and below by the plane region
and below by the plane region  .
. 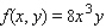 and
and 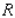 is the region bounded by the graphs of
is the region bounded by the graphs of 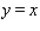 and
and 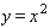 .
.
(Essay)
4.9/5  (43)
(43)
In a survey it was determined that the demand equation for VCRs is given by  The demand equation for blank VCR tapes is given by
The demand equation for blank VCR tapes is given by 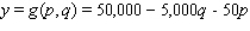 where
where 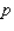 and
and 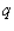 denote the unit prices, respectively, and
denote the unit prices, respectively, and 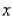 and
and  denote the number of VCRs and the number of blank VCR tapes demanded each week. Determine whether these two products are substitute, complementary, or neither.
denote the number of VCRs and the number of blank VCR tapes demanded each week. Determine whether these two products are substitute, complementary, or neither.
(Essay)
5.0/5  (37)
(37)
Evaluate the double integral 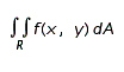 for the function
for the function 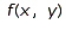 and the region
and the region  .
. 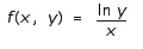 and
and  is the rectangle defined by
is the rectangle defined by 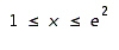 and
and 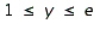 .
.
(Multiple Choice)
4.9/5  (37)
(37)
Use a double integral to find the volume of the solid shown in the figure. 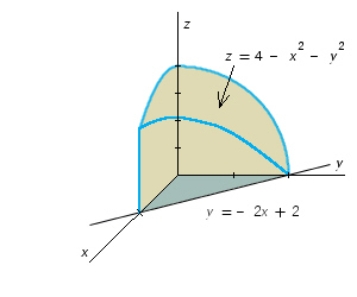
(Multiple Choice)
4.8/5  (34)
(34)
The Ross-Simons Company has a monthly advertising budget of $40,000. Their marketing department estimates that if they spend x dollars on newspaper advertising and y dollars on television advertising, then the monthly sales will be given by 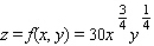 dollars. Determine how much money Ross-Simons should spend on newspaper ads and on television ads each month to maximize its monthly sales.
dollars. Determine how much money Ross-Simons should spend on newspaper ads and on television ads each month to maximize its monthly sales.
(Essay)
4.8/5  (33)
(33)
The Company requires that its corned beef hash containers have a capacity of 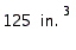 , be right circular cylinders, and be made of a tin alloy. Find the radius and height of the least expensive container that can be made if the metal for the side and bottom costs
, be right circular cylinders, and be made of a tin alloy. Find the radius and height of the least expensive container that can be made if the metal for the side and bottom costs  and the metal for the pull-off lid costs
and the metal for the pull-off lid costs  .
.
(Essay)
4.7/5  (41)
(41)
An auxiliary electric power station will serve three communities, A, B, and C, whose relative locations are shown in the accompanying figure. Determine where the power station should be located if the sum of the squares of the distances from each community to the site is minimized. Please round the answer to the nearest hundredth. 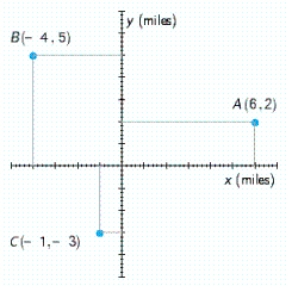
(Essay)
4.8/5  (31)
(31)
Find the volume of the solid bounded above by the surface 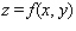 and below by the plane region
and below by the plane region 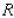 .
.  and
and 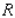 is the region bounded by the graphs of
is the region bounded by the graphs of 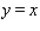 and
and 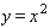 .
.
(Multiple Choice)
4.9/5  (39)
(39)
Find the second-order partial derivatives of the given function. Show that the mixed partial derivatives 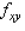 and
and 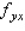 are equal.
are equal. 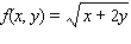
(Multiple Choice)
4.8/5  (37)
(37)
If  is a function of x and y, then there are functions
is a function of x and y, then there are functions 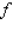 and
and 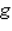 of one variable such a that
of one variable such a that
(Multiple Choice)
4.8/5  (41)
(41)
The total daily profit (in dollars) realized by Weston Publishing in publishing and selling its dictionaries is given by the profit function 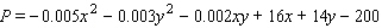 where x stands for the number of deluxe editions and y denotes the number of standard editions sold daily. Weston's management decides that publication of these dictionaries should be restricted to a total of exactly 400 copies/day. How many deluxe copies and how many standard copies should be published each day to maximize Weston's daily profit?
Find the number of deluxe copies.
Find the number of standard copies.
where x stands for the number of deluxe editions and y denotes the number of standard editions sold daily. Weston's management decides that publication of these dictionaries should be restricted to a total of exactly 400 copies/day. How many deluxe copies and how many standard copies should be published each day to maximize Weston's daily profit?
Find the number of deluxe copies.
Find the number of standard copies.
(Essay)
4.9/5  (35)
(35)
Find the critical point(s) of the function. Then use the second derivative test to classify the nature of each point, if possible. Finally, determine the relative extrema of the function. 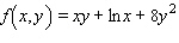 Find the critical point(s) of the function.
Find the point(s) of maximum.
Find the point(s) of minimum.
Find the relative extrema of the function.
Find the critical point(s) of the function.
Find the point(s) of maximum.
Find the point(s) of minimum.
Find the relative extrema of the function.
(Essay)
4.8/5  (29)
(29)
The total weekly revenue (in dollars) of Country Workshop associated with manufacturing and selling their rolltop desks is given by the function 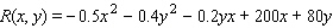 where x denotes the number of finished units and y denotes the number of unfinished units manufactured and sold each week. Compute
where x denotes the number of finished units and y denotes the number of unfinished units manufactured and sold each week. Compute 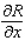 and
and  when x = 200 and y = 180. Interpret your results.
when x = 200 and y = 180. Interpret your results.
(Multiple Choice)
4.8/5  (40)
(40)
Body mass index (BMI) is used to identify, evaluate, and treat overweight and obese adults. The BMI value for an adult of weight 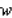 (in kilograms) and height
(in kilograms) and height 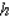 (in meters) is defined to be
(in meters) is defined to be  According to federal guidlines, an adult is overweight iff he or she has a BMI value greater than 25 but less than 30 and is "obese" if the value greater than or equal to 30. What is the BMI of adult who weights in at 80 kg and stands 1.7 m tall?
Round your answers to two decimal places if necessary.
According to federal guidlines, an adult is overweight iff he or she has a BMI value greater than 25 but less than 30 and is "obese" if the value greater than or equal to 30. What is the BMI of adult who weights in at 80 kg and stands 1.7 m tall?
Round your answers to two decimal places if necessary.
(Multiple Choice)
4.8/5  (35)
(35)
With computer security always a hot-button issue, demand is growing for technology that authenticates and authorizes computer users. The following table gives the authentication software sales (in billions of dollars), including projections, from 1999 through 2004.  (Here, x = 0 represents 1999.)
Find an equation of the least-squares line for these data. Please round the coefficients in your equation to three decimal places. Use the result to estimate the sales for 2006, assuming the projection is accurate.
(Here, x = 0 represents 1999.)
Find an equation of the least-squares line for these data. Please round the coefficients in your equation to three decimal places. Use the result to estimate the sales for 2006, assuming the projection is accurate.
(Multiple Choice)
4.8/5  (28)
(28)
Showing 161 - 180 of 206
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)