Exam 12: Linear Regression and Correlation

i. If the coefficient of correlation is 0.70, what is the coefficient of determination? ii. If the value of r is -0.88, what does this indicate about the dependent variable as the independent variable increases? iii. If the correlation between sales and advertising is -0.7, what percent of the variation in sales can be attributed to advertising?

A regression analysis yields the following information: Y' = 2.24 + 1.49 X S_{y x} = 1.66; $\Sigma$ x = 32; $\Sigma$ x² = 134; n = 10 Estimate the value of Y' when X = 4.

i. Trying to predict weekly sales with a standard error of estimate of $1,955, we would conclude that 68 percent of the predictions would not be off more than $1,955, 95 percent would not be off by more $3,910, and 99.7 percent would not be off by more than $5,865. ii. Approximately 68% of the values lie within one standard error of the regression line. iii. For a set of observations, there is no difference in the width of a confidence interval and the width of a predictor interval.

i. The smaller the sample, the smaller the possible error as measured by the standard error of estimate. ii. Approximately 68% of the values lie within two standard errors of the regression line. iii. For a set of observations, there is no difference in the width of a confidence interval and the width of a predictor interval.

The partial Mega Stat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports. Refer to the printout above. Predict the number of wins for a team with PAYROLL = 25(million) (nearest whole number)

i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis. ii. In order to visualize the form of the regression equation, we can draw a scatter diagram. iii. A regression equation may be determined using a mathematical method called the.

i. If the value of r is -0.96, what does this indicate about the dependent variable as the independent variable increases? ii. What is the value of the correlation coefficient if there is perfect correlation? iii. If the dependent variable is measured in dollars, in what units is the standard error of estimate measured?

If testing the hypothesis: H₀: $\rho$ = 0, the computed t - statistic is:

The partial Mega Stat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports. 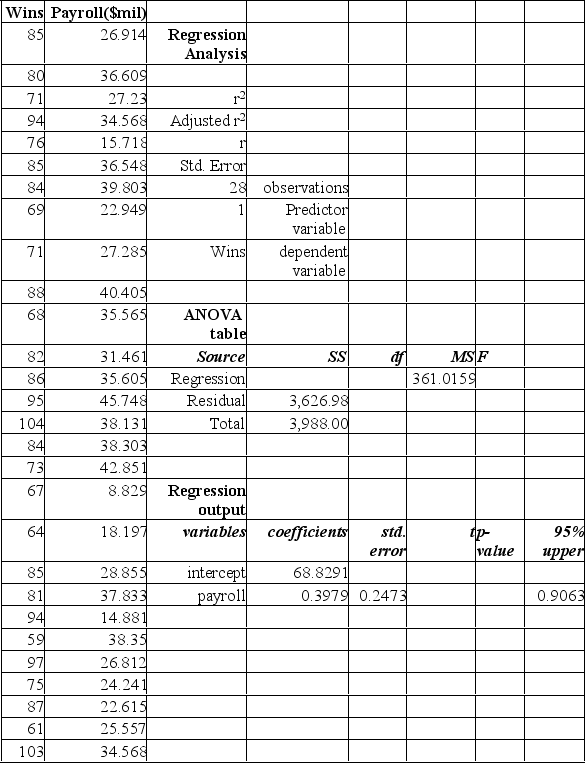Refer to the printout above. The Sum of Squares Regression is:

If the correlation between two variables is close to one, the association is

i. The basic question in testing the significance of rho is to see if there is zero correlation in the population from which the sample was selected. ii. A z test is used to test the significance of the coefficient of correlation. iii. Perfect correlation means that the scatter diagram will appear as a straight line.

i. If we are studying the relationship between high school performance and college performance, and want to predict college performance, high school performance is the dependent variable. ii. A financial advisor is interested in predicting bond yield based on bond term, i.e., one year, two years, etc. The dependent variable is bond term. iii. The variable used to predict the value of another is called the dependent variable.

When comparing the 95% confidence and prediction intervals for a given regression analysis,

Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Excel results are summarized below: 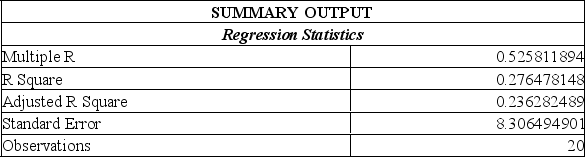From this printout you determine:

The relationship between interest rates as a percent (X) and housing starts (Y) is given by the linear equation Y' = 4094 - 269X. What will be the number of housing starts if the interest rate rose to 16%?

Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Excel results are summarized below: 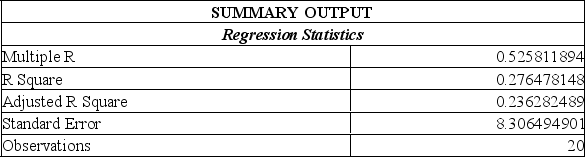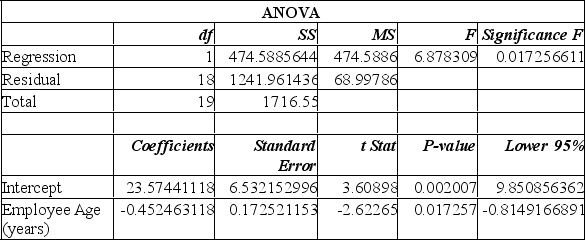From this printout you determine:

i. If we are studying the relationship between high school performance and college performance, and want to predict college performance, high school performance is the independent variable. ii. An economist is interested in predicting the unemployment rate based on gross domestic product. Since the economist is interested in predicting unemployment, the independent variable is gross domestic product. iii. The variable used to predict the value of another is called the dependent variable.

i. The purpose of correlation analysis is to find how strong the relationship is between two variables. ii. A coefficient of correlation of -0.96 indicates a very weak negative correlation. iii. The standard error of estimate measures the accuracy of our prediction.

The partial Mega Stat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports. 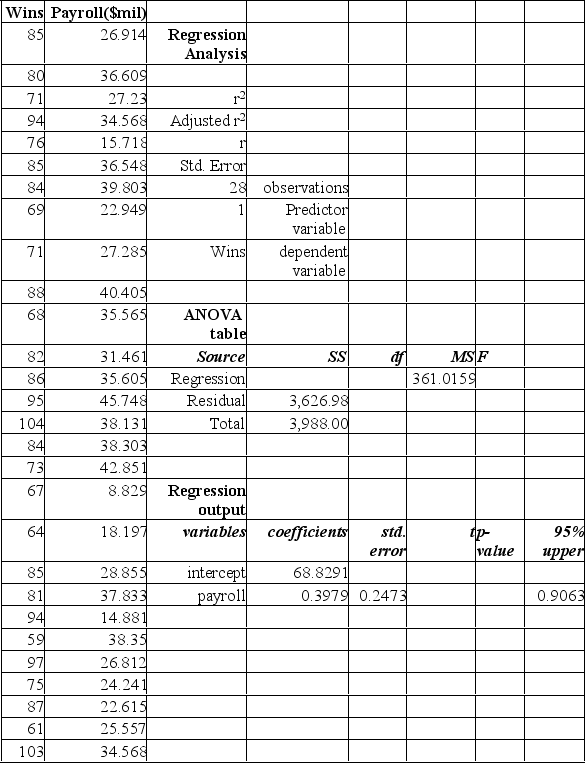Refer to the printout above. Predict the number of wins for a team with PAYROLL = 25(million) (nearest whole number)

Data is collected from 20 sales people in order to verify that the more contacts made with potential clients, the greater the sales volume. The Excel printout is shown below. 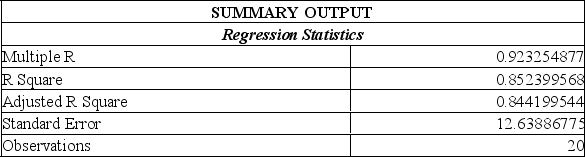From this printout you determine: