Exam 12: Linear Regression and Correlation
Exam 1: What Is Statistics79 Questions
Exam 2: Describing Data: Frequency Tables, Frequency Distributions, and Graphic Presentation87 Questions
Exam 3: Describing Data: Numerical Measures191 Questions
Exam 4: A Survey of Probability Concepts130 Questions
Exam 5: Discrete Probability Distributions121 Questions
Exam 6: Continuous Probability Distributions143 Questions
Exam 7: Sampling Methods and the Central Limit Theorem78 Questions
Exam 8: Estimation and Confidence Intervals134 Questions
Exam 9: One-Sample Tests of Hypothesis139 Questions
Exam 10: Two-Sample Tests of Hypothesis103 Questions
Exam 11: Analysis of Variance97 Questions
Exam 12: Linear Regression and Correlation166 Questions
Exam 13: Multiple Regression and Correlation Analysis128 Questions
Exam 14: Chi-Square Applications126 Questions
Exam 15: Index Numbers93 Questions
Exam 16: Time Series and Forecasting90 Questions
Exam 17: An Introduction to Decision Theory54 Questions
Select questions type
A sales manager for an advertising agency believes there is a relationship between the number of contacts and the amount of the sales. To verify this belief, the following data was collected: 
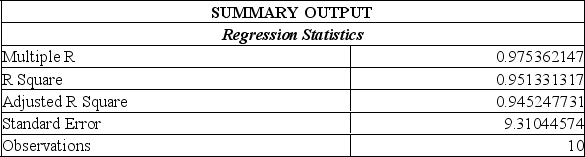
 What is the value of the coefficient of correlation?
What is the value of the coefficient of correlation?
(Multiple Choice)
4.7/5  (37)
(37)
Which value of r indicates a stronger correlation than 0.25?
(Multiple Choice)
4.8/5  (37)
(37)
What is the chart called when the paired data (the dependent and independent variables) are plotted?
(Multiple Choice)
4.8/5  (34)
(34)
The partial Mega Stat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports. 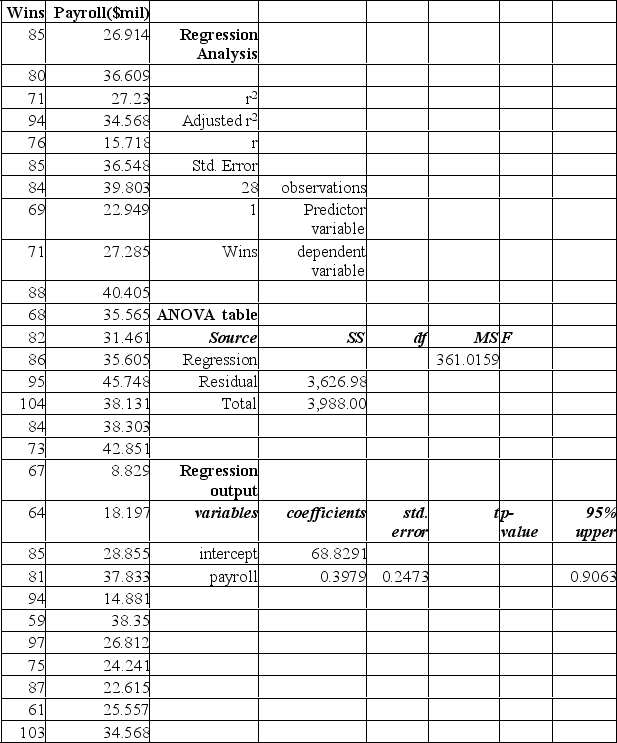 Refer to the printout above. How many degrees of freedom for Residual (Error)?
Refer to the printout above. How many degrees of freedom for Residual (Error)?
(Multiple Choice)
4.9/5  (33)
(33)
i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis. ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
iii. The equation for a straight line going through the plots on a scatter diagram is called a regression equation. It is alternately called an estimating equation and a predicting equation.
(Multiple Choice)
4.7/5  (26)
(26)
The partial Mega Stat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports.  Refer to the printout above. The critical value of t, at the 5% level of significance, for testing the coefficient of correlation is:
Refer to the printout above. The critical value of t, at the 5% level of significance, for testing the coefficient of correlation is:
(Multiple Choice)
4.9/5  (41)
(41)
i. The basic question in testing the significance of rho is to see if there is zero correlation in the population from which the sample was selected. ii. A t test is used to test the significance of the coefficient of correlation.
iii. When testing the strength of the relationship between two variables, the alternate hypothesis is: H0: 0.
(Multiple Choice)
4.9/5  (36)
(36)
i. The basic question in testing the significance of rho is to see if there is zero correlation in the population from which the sample was selected. ii. A t test is used to test the significance of the coefficient of correlation.
iii. Suppose a sample of 15 homes recently sold in your area is obtained. The correlation between the area of the home, in square feet, and the selling price is 0.40. We want to test the hypothesis that the correlation in the population is zero versus the alternate that it is greater than zero. You determine that the rejection region should fall in the lower tail if this is a one-tailed test and we use a 0.01 significance level.
(Multiple Choice)
4.7/5  (37)
(37)
If r = 0.65, what does the coefficient of determination equal?
(Multiple Choice)
4.7/5  (40)
(40)
Data is collected from 20 sales people in order to verify that the more contacts made with potential clients, the greater the sales volume. The Excel printout is shown below. 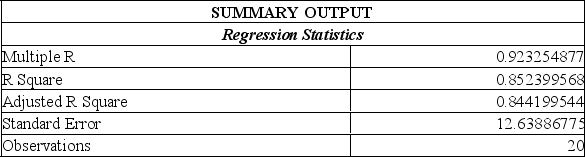
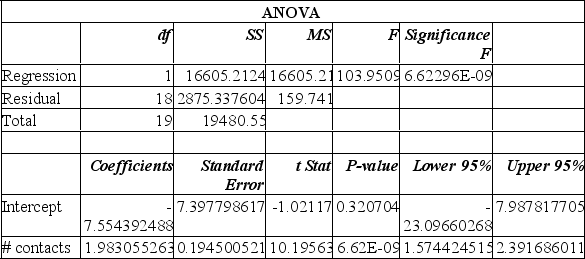 The y-intercept in this instance suggests:
The y-intercept in this instance suggests:
(Multiple Choice)
4.7/5  (29)
(29)
The following table shows the number of workdays absent based on the length of employment in years.  i. The least squares equation for the data: Y' = 7.107 - 0.6852X.
ii. The dependent variable (Y) is the number of work days absent.
iii. The slope of the linear equation is -0.6852.
i. The least squares equation for the data: Y' = 7.107 - 0.6852X.
ii. The dependent variable (Y) is the number of work days absent.
iii. The slope of the linear equation is -0.6852.
(Multiple Choice)
4.8/5  (35)
(35)
i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis. ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
iii. When a regression line has a zero slope, indicating a lack of a relationship, the line is horizontal to the x-axis.
(Multiple Choice)
4.8/5  (41)
(41)
Using a 95% significance level, what is the critical value for the F-statistic? 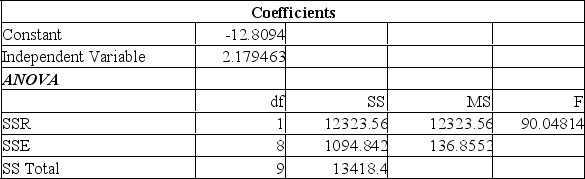
(Multiple Choice)
4.9/5  (33)
(33)
i. A correlation coefficient of -1 or +1 indicates perfect correlation. ii. The strength of the correlation between two variables depends on the sign of the coefficient of correlation.
iii. If the coefficient of correlation is -0.90, the coefficient of determination is -0.81.
(Multiple Choice)
4.9/5  (35)
(35)
A sales manager for an advertising agency believes there is a relationship between the number of contacts and the amount of the sales. To verify this belief, the following data was collected: 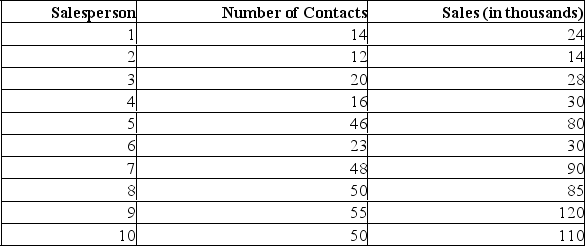

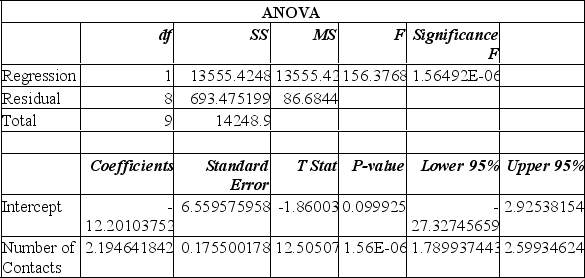 What is the regression equation?
What is the regression equation?
(Multiple Choice)
4.9/5  (35)
(35)
Given the following five points: (-2,0), (-1,0), (0,1), (1,1), and (2,3). If the regression equation is Y' = 2 - 0.4X, what is the value of Y' when X = -3?
(Multiple Choice)
4.8/5  (32)
(32)
The correlation between two variables is -0.63 for a sample of 20 observations. What is the computed value of the test statistic?
(Multiple Choice)
4.8/5  (33)
(33)
The partial Mega Stat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports. 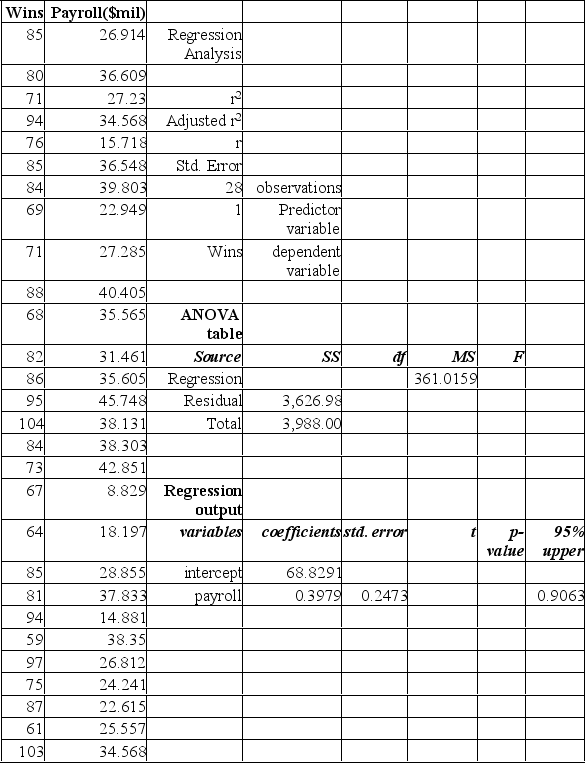 Refer to the printout above. The regression equation is:
Refer to the printout above. The regression equation is:
(Multiple Choice)
4.8/5  (32)
(32)
Showing 81 - 100 of 166
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)