Exam 12: Linear Regression and Correlation
Exam 1: What Is Statistics79 Questions
Exam 2: Describing Data: Frequency Tables, Frequency Distributions, and Graphic Presentation87 Questions
Exam 3: Describing Data: Numerical Measures191 Questions
Exam 4: A Survey of Probability Concepts130 Questions
Exam 5: Discrete Probability Distributions121 Questions
Exam 6: Continuous Probability Distributions143 Questions
Exam 7: Sampling Methods and the Central Limit Theorem78 Questions
Exam 8: Estimation and Confidence Intervals134 Questions
Exam 9: One-Sample Tests of Hypothesis139 Questions
Exam 10: Two-Sample Tests of Hypothesis103 Questions
Exam 11: Analysis of Variance97 Questions
Exam 12: Linear Regression and Correlation166 Questions
Exam 13: Multiple Regression and Correlation Analysis128 Questions
Exam 14: Chi-Square Applications126 Questions
Exam 15: Index Numbers93 Questions
Exam 16: Time Series and Forecasting90 Questions
Exam 17: An Introduction to Decision Theory54 Questions
Select questions type
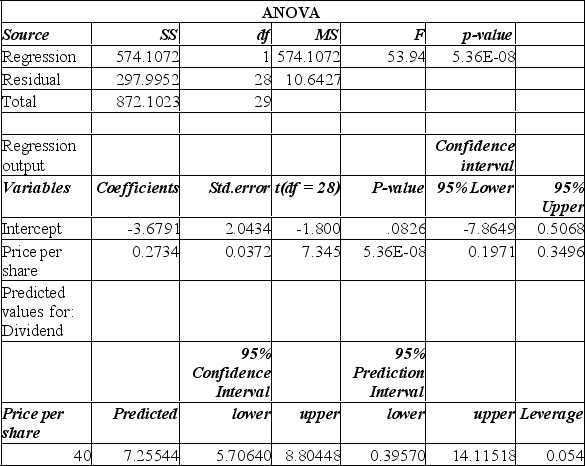
We have collected price per share and dividend information from a sample of 30 companies. Using the Mega Stat printout, determine the regression equation that predicts the dividend from the stock's selling price. 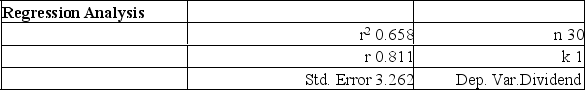
(Multiple Choice)
4.9/5  (32)
(32)
i. The least squares technique minimizes the sum of the squares of the vertical distances between the actual Y values and the predicted values of Y. ii. When a regression line has a zero slope, indicating a lack of a relationship, the line is vertical to the x-axis.
iii. In regression analysis, the predicted value of Y' rarely agrees exactly with the actual Y value, i.e., we expect some prediction error.
(Multiple Choice)
4.9/5  (26)
(26)
i. Trying to predict weekly sales with a standard error of estimate of $1,955, we would conclude that 68 percent of the predictions would not be off more than $1,955, 95 percent would not be off by more $3,910, and 99.7 percent would not be off by more than $5,865. ii. The smaller the sample, the smaller the possible error as measured by the standard error of estimate.
iii. Approximately 95% of the values lie within two standard errors of the regression line.
(Multiple Choice)
4.9/5  (34)
(34)
i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis. ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
iii. The least squares technique minimizes the sum of the squares of the vertical distances between the actual Y values and the predicted values of Y.
(Multiple Choice)
4.8/5  (28)
(28)
Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Partial excel results are summarized below from two different samples: 
 Given this information alone, would you decide to continue with the regression analysis for sample #1 or #2 or both?
Given this information alone, would you decide to continue with the regression analysis for sample #1 or #2 or both?
(Multiple Choice)
4.9/5  (24)
(24)
A sales manager for an advertising agency believes there is a relationship between the number of contacts and the amount of the sales. To verify this belief, the following data was collected: 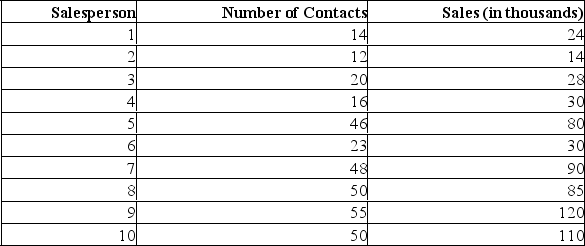

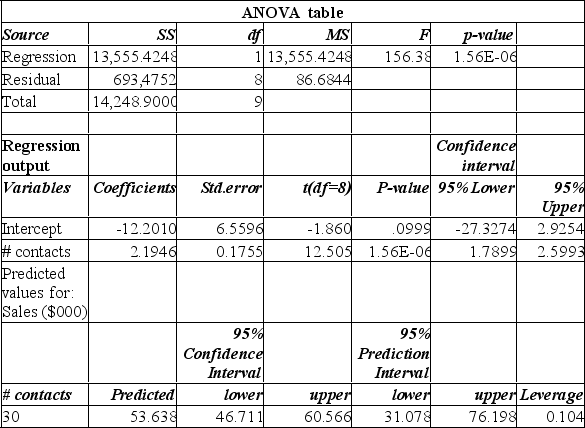 The 95% confidence interval for 30 calls is
The 95% confidence interval for 30 calls is
(Multiple Choice)
4.9/5  (41)
(41)
The difference between formulas for constructing a confidence interval and a prediction interval is
(Multiple Choice)
5.0/5  (41)
(41)
What is the range of values for the coefficient of determination?
(Multiple Choice)
4.7/5  (37)
(37)
i. The purpose of correlation analysis is to find how strong the relationship is between two variables. ii. A coefficient of correlation close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
iii. The strength of the correlation between two variables depends on the sign of the coefficient of correlation.
(Multiple Choice)
4.8/5  (31)
(31)
We have collected price per share and dividend information from a sample of 30 companies. 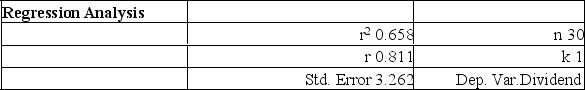
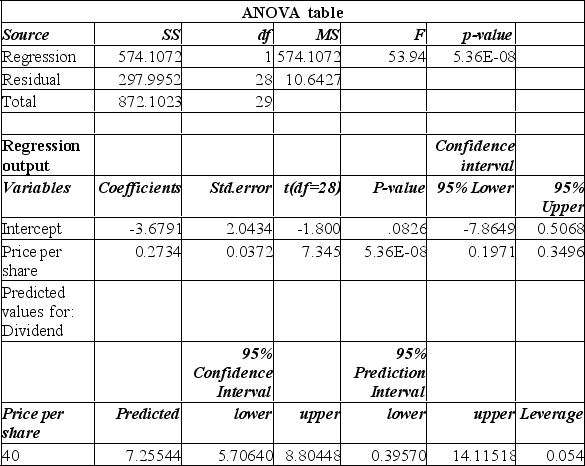 Determine the 95% confidence interval for Dividends when the stock price is $40 per share:
Determine the 95% confidence interval for Dividends when the stock price is $40 per share:
(Multiple Choice)
4.8/5  (34)
(34)
Given the following five points: (-2,0), (-1,0), (0,1), (1,1), and (2,3). What is the critical value necessary to determine a confidence interval for a 95% level of confidence?
(Multiple Choice)
4.8/5  (36)
(36)
i. The coefficient of correlation is a measure of the strength of relationship between two variables. ii. The coefficient of determination can only be positive.
iii. The standard error of estimate measures the accuracy of our prediction.
(Multiple Choice)
4.8/5  (38)
(38)
i. A scatter diagram is a chart that portrays the relationship between two variables. ii. If a scatter diagram shows very little scatter about a straight line drawn through the plots, it indicates a rather weak relationship.
iii. A scatter diagram may be put together using excel or Mega Stat.
(Multiple Choice)
4.8/5  (34)
(34)
i. A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak. ii. The coefficient of determination is the proportion of the total variation in the dependent variable Y that is explained or accounted for by its relationship with the independent variable X.
iii. If the coefficient of correlation is -0.90, the coefficient of determination is -0.81.
(Multiple Choice)
4.8/5  (34)
(34)
i. A correlation coefficient of -1 or +1 indicates perfect correlation. ii. A coefficient of correlation close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
iii. Coefficients of -0.91 and +0.91 have equal strength.
(Multiple Choice)
4.8/5  (39)
(39)
What is the difference between a confidence interval and a prediction interval for the dependent variable in correlation analysis?
(Multiple Choice)
5.0/5  (32)
(32)
The relationship between interest rates as a percent (X) and housing starts (Y) is given by the linear equation Y' = 4094 - 269X. At what interest rate will there be no permits for housing starts?
(Multiple Choice)
4.7/5  (40)
(40)
A sales manager for an advertising agency believes there is a relationship between the number of contacts and the amount of the sales. To verify this belief, the following data was collected: 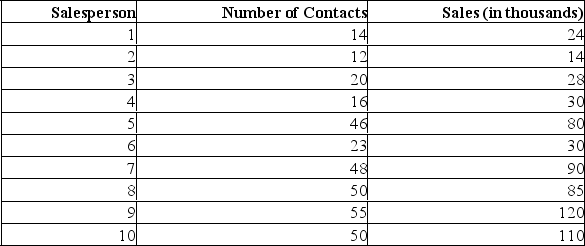
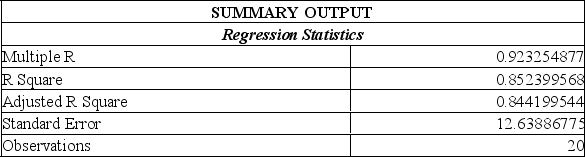
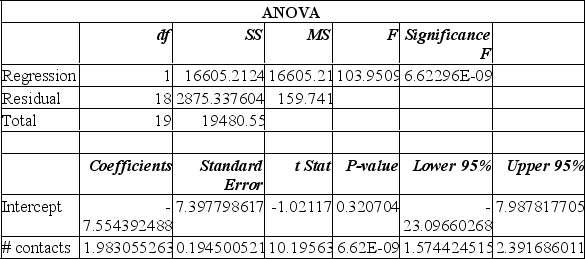 What is the independent variable?
What is the independent variable?
(Multiple Choice)
4.7/5  (35)
(35)
The following table shows the number of workdays absent based on the length of employment in years.  i. The Y intercept of the linear equation is 7.7407.
ii. The dependent variable (Y) is the number of years employed.
iii. The slope of the linear equation is -0.6852.
i. The Y intercept of the linear equation is 7.7407.
ii. The dependent variable (Y) is the number of years employed.
iii. The slope of the linear equation is -0.6852.
(Multiple Choice)
4.8/5  (32)
(32)
Showing 101 - 120 of 166
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)