Exam 15: B:nonparametric Statistics
Hamburger Ratings Narrative
The restaurant critic at a newspaper claims that the hamburgers that one gets at the hamburger chain restaurants are all equally bad, and that people who claim to like one hamburger over others are victims of advertising. In fact, he claims that if there were no differences in appearance, then all hamburgers would be rated equally. To test the critic's assertion, ten teenagers are asked to taste hamburgers from three different fast-food chains. Each hamburger is dressed in the same way (mustard, relish, tomato, and pickle) and with the same type of bun. The teenagers taste each hamburger and rate it on a 9-point scale with 1 = bad and 9 = excellent. The data are listed below.
Hamburger Ratings  -Refer to Hamburger Ratings Narrative. Can we infer at the 1% significance level that the critic is wrong? Justify your response.
-Refer to Hamburger Ratings Narrative. Can we infer at the 1% significance level that the critic is wrong? Justify your response.
 The locations of all three populations are the same.
The locations of all three populations are the same. 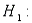 At least two population locations differ.
At least two population locations differ.
Rejection region:  Test statistic:
Test statistic: 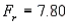 Conclusion: Don't reject the null hypothesis. No, we can't infer at the 1% significance level that the critic is wrong.
Conclusion: Don't reject the null hypothesis. No, we can't infer at the 1% significance level that the critic is wrong.
To investigate the effect of sleep on basal metabolism, seven students who averaged seven or more hours of sleep a night (Group A), and five students who averaged less than seven hours of sleep a night (Group B), were examined and their basal metabolism recorded as shown below.  Since it was not clear whether the assumptions for a t test were valid, the researcher decided to employ nonparametric methods. Use the Wilcoxon rank sum test to determine whether the metabolism measurements for Group A are significantly higher than those of Group B. Use
Since it was not clear whether the assumptions for a t test were valid, the researcher decided to employ nonparametric methods. Use the Wilcoxon rank sum test to determine whether the metabolism measurements for Group A are significantly higher than those of Group B. Use 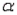 = 0.05.
= 0.05.
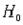 : The distributions of metabolism measurements for the two groups are identical.
: The distributions of metabolism measurements for the two groups are identical.  : The distribution of metabolism measurements for Group B is shifted to the left of Group A. (i.e., the metabolism measurements are lower for Group B).
: The distribution of metabolism measurements for Group B is shifted to the left of Group A. (i.e., the metabolism measurements are lower for Group B).
Let Group B be population 1 since it has the smaller sample size. We first need to rank the observations from small to large as shown below. 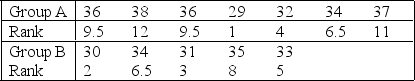 The test statistic is
The test statistic is 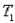 = 2 + 6.5 + 3 + 8 + 5 = 24.5. For left-tailed test with
= 2 + 6.5 + 3 + 8 + 5 = 24.5. For left-tailed test with  = 0.05,
= 0.05,  = 5, and
= 5, and 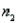 = 7, the critical value for the Wilcoxon rank sum test is 21. Reject
= 7, the critical value for the Wilcoxon rank sum test is 21. Reject 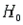 if
if 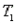
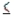
21. Since 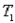 > 21, do not reject
> 21, do not reject  , and therefore cannot conclude the distribution of metabolism measurements for Group B is lower than those for Group A.
, and therefore cannot conclude the distribution of metabolism measurements for Group B is lower than those for Group A.
Use the Wilcoxon rank sum test on the data below to determine at the 10% significance level whether the two population locations differ.
Sample 1: 32 22 19 29 20 34 25 9 28 17
Sample 2: 29 20 18 27 19 23 19 12 22 10
 The two population locations are the same.
The two population locations are the same. 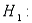 The two population locations are different.
The two population locations are different.
Rejection region: 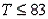 Test statistic:
Test statistic: 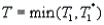 = 90.5
= 90.5
Conclusion: Don't reject the null hypothesis. The two population locations are the same.
Advertisement Narrative
In a Kruskal-Wallis test to determine whether differences exist among three different advertisements, the following statistics were obtained:  ,
, 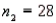 ,
, 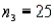 ,
, 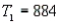 ,
, 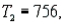 and
and  .
-Refer to Advertisement Narrative. Explain how to use the p-value for testing the hypotheses.
.
-Refer to Advertisement Narrative. Explain how to use the p-value for testing the hypotheses.
Frozen TV Dinner Narrative
The general manager of a frozen TV dinner maker must decide which one of four new dinners to introduce to the market. He decides to perform an experiment to help make a decision. Each dinner is sampled by ten people who then rate the product on a 7-point scale, where 1 = poor, and 7 = excellent. The results are shown below.
Taste Ratings 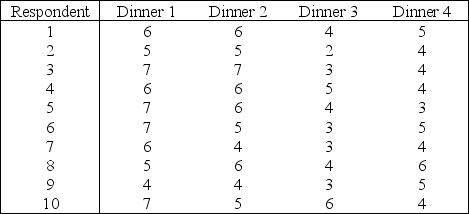 -Refer to Frozen TV Dinner Narrative. Which statistical technique can the general manager use to help him make a decision?
-Refer to Frozen TV Dinner Narrative. Which statistical technique can the general manager use to help him make a decision?
Children and Antibiotics Narrative
In a study of the palatability of antibiotics in children, a medical team used a voluntary sample of healthy children to assess their reactions to the taste of four antibiotics. The children's response was measured on a 10 centimetre (cm) visual analogue scale incorporating the use of faces, from sad (low score) to happy (high score). The minimum score was 0 and the maximum was 10. For the accompanying data, each of five children was asked to taste each of four antibiotics and rate them using the visual (faces) analogue scale from 0 to 10 cm.
Antibiotic 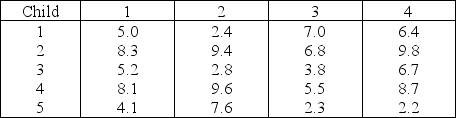 -Refer to Children and Antibiotics Narrative. Use the appropriate nonparametric test to test for differences in the distributions of responses to the tastes of the four antibiotics.
-Refer to Children and Antibiotics Narrative. Use the appropriate nonparametric test to test for differences in the distributions of responses to the tastes of the four antibiotics.
Standardized College Entrance Exam Narrative
Two psychometricians (educators who are experts in the field of psychological test design) were asked to rank six designs for a new standardized college entrance exam.
Design  This uses Spearman's rank correlation coefficient to see if there is a positive relationship between the educators' rankings.
-Refer to Standardized College Entrance Exam Narrative. What is the observed significance level for this test?
This uses Spearman's rank correlation coefficient to see if there is a positive relationship between the educators' rankings.
-Refer to Standardized College Entrance Exam Narrative. What is the observed significance level for this test?
Attitude Test Narrative
Twenty students are given an attitude test before and after viewing a motion picture designed to change their attitudes favourably toward a new curriculum. A high score indicates a favourable attitude and a low score indicates an unfavourable attitude, with the scores ranging from 1 to 30. This will use the sign test on the data given below to see if we can conclude the motion picture was successful in improving attitudes. 
 -Refer to Attitude Test Narrative. Find the p-value for the Wilcoxon signed-rank test.
-Refer to Attitude Test Narrative. Find the p-value for the Wilcoxon signed-rank test.
Attitude Test Narrative
Twenty students are given an attitude test before and after viewing a motion picture designed to change their attitudes favourably toward a new curriculum. A high score indicates a favourable attitude and a low score indicates an unfavourable attitude, with the scores ranging from 1 to 30. This will use the sign test on the data given below to see if we can conclude the motion picture was successful in improving attitudes. 
 -Refer to Attitude Test Narrative. At what level of significance could we reject
-Refer to Attitude Test Narrative. At what level of significance could we reject  ?
?
A paired-difference experiment was conducted to compare two populations. The data are shown in the table. Use a sign test to determine whether the population distributions are different.
Pairs  a. State the null and alternative hypotheses for the test.
b. Determine an appropriate rejection region with
a. State the null and alternative hypotheses for the test.
b. Determine an appropriate rejection region with 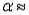 0.01.
c. Calculate the observed value of the test statistic.
d. Do the data present sufficient evidence to indicate that populations 1 and 2 are different?
0.01.
c. Calculate the observed value of the test statistic.
d. Do the data present sufficient evidence to indicate that populations 1 and 2 are different?
Given the statistics 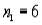 ,
,  ,
, 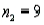 ,
,  , use the Wilcoxon rank sum test to determine at the 5% significance whether the location of population 1 is to the right of the location of population 2.
, use the Wilcoxon rank sum test to determine at the 5% significance whether the location of population 1 is to the right of the location of population 2.
Reaction Times Narrative
The reaction times to three stimuli were recorded for each of eight subjects. The data, recorded in seconds, are shown below. This uses Friedman's Fr test to determine if there is a difference among the population distributions of reaction times. 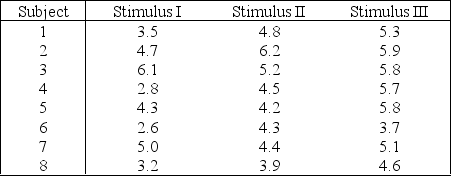 -Refer to Reaction Times Narrative. Find the rejection region for
-Refer to Reaction Times Narrative. Find the rejection region for  = 0.05.
= 0.05.
Standardized College Entrance Exam Narrative
Two psychometricians (educators who are experts in the field of psychological test design) were asked to rank six designs for a new standardized college entrance exam.
Design  This uses Spearman's rank correlation coefficient to see if there is a positive relationship between the educators' rankings.
-Refer to Standardized College Entrance Exam Narrative. Describe why the test statistic
This uses Spearman's rank correlation coefficient to see if there is a positive relationship between the educators' rankings.
-Refer to Standardized College Entrance Exam Narrative. Describe why the test statistic 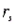 is called the rank correlation coefficient. What is the value of
is called the rank correlation coefficient. What is the value of 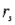 in this ?
in this ?
A car dealer was interested in comparing two brands of tires to see if they yielded different wear length (in thousands of km). The dealer selected eight cars at random and used each of the brands of tires on each car. The wear length was recorded as follows: 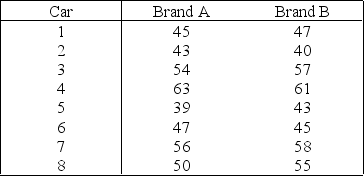 Use the sign test to see if the distribution of wear length is the same for both brands of tires. Use
Use the sign test to see if the distribution of wear length is the same for both brands of tires. Use 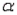 = 0.05.
= 0.05.
Children and Antibiotics Narrative
In a study of the palatability of antibiotics in children, a medical team used a voluntary sample of healthy children to assess their reactions to the taste of four antibiotics. The children's response was measured on a 10 centimetre (cm) visual analogue scale incorporating the use of faces, from sad (low score) to happy (high score). The minimum score was 0 and the maximum was 10. For the accompanying data, each of five children was asked to taste each of four antibiotics and rate them using the visual (faces) analogue scale from 0 to 10 cm.
Antibiotic  -Refer to Children and Antibiotics Narrative. Comment on the results of the analysis of variance compared with the nonparametric test.
-Refer to Children and Antibiotics Narrative. Comment on the results of the analysis of variance compared with the nonparametric test.
The following data were generated from a blocked experiment. Conduct a Friedman test at the 5% significance level to determine if at least two population locations differ.
Treatment 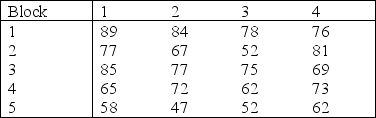
Given the statistics 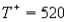 ,
, 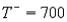 , and n = 50 from a matched pairs experiment, perform the Wilcoxon signed-rank test to determine whether we can infer at the 5% significance level that the two population locations differ.
, and n = 50 from a matched pairs experiment, perform the Wilcoxon signed-rank test to determine whether we can infer at the 5% significance level that the two population locations differ.
Standardized College Entrance Exam Narrative
Two psychometricians (educators who are experts in the field of psychological test design) were asked to rank six designs for a new standardized college entrance exam.
Design  This uses Spearman's rank correlation coefficient to see if there is a positive relationship between the educators' rankings.
-Refer to Standardized College Entrance Exam Narrative. State the null and alternative hypotheses.
This uses Spearman's rank correlation coefficient to see if there is a positive relationship between the educators' rankings.
-Refer to Standardized College Entrance Exam Narrative. State the null and alternative hypotheses.
Attitude Test Narrative
Twenty students are given an attitude test before and after viewing a motion picture designed to change their attitudes favourably toward a new curriculum. A high score indicates a favourable attitude and a low score indicates an unfavourable attitude, with the scores ranging from 1 to 30. This will use the sign test on the data given below to see if we can conclude the motion picture was successful in improving attitudes. 
 -Refer to Attitude Test Narrative. Using the answers to the previous two questions, can we conclude that the motion picture was successful in changing attitudes?
-Refer to Attitude Test Narrative. Using the answers to the previous two questions, can we conclude that the motion picture was successful in changing attitudes?
Customers' Ages Narrative
The marketing manager of a pizza chain is in the process of examining some of the demographic characteristics of her customers. In particular, she would like to investigate the belief that the ages of the customers of pizza parlours, hamburger emporiums, and fast-food chicken restaurants are different. As an experiment, the ages of eight customers of each of the restaurants are recorded and listed below. From previous analysis we know that the ages are not normally distributed.
Customers' Ages 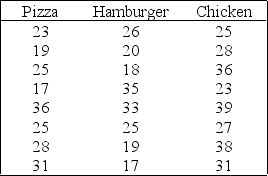 -Refer to Customers' Ages Narrative. Using the appropriate statistical table, what statement can be made about the p-value for this test?
-Refer to Customers' Ages Narrative. Using the appropriate statistical table, what statement can be made about the p-value for this test?
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)