Exam 15: Chi-Squared Tests
Exam 1: What Is Statistics43 Questions
Exam 2: Graphical Descriptive Techniques I93 Questions
Exam 3: Graphical Descriptive Techniques II183 Questions
Exam 4: Numerical Descriptive Techniques274 Questions
Exam 5: Data Collection and Sampling82 Questions
Exam 6: Probability234 Questions
Exam 7: Random Variables and Discrete Probability Distributions274 Questions
Exam 8: Continuous Probability Distributions215 Questions
Exam 9: Sampling Distributions153 Questions
Exam 10: Introduction to Estimation152 Questions
Exam 11: Introduction to Hypothesis Testing182 Questions
Exam 12: Inference About a Population147 Questions
Exam 13: Inference About Comparing Two Populations170 Questions
Exam 14: Analysis of Variance153 Questions
Exam 15: Chi-Squared Tests175 Questions
Exam 16: Simple Linear Regression and Correlation298 Questions
Exam 17: Multiple Regression157 Questions
Select questions type
You find the expected value for each cell in a chi-squared test for a contingency table by multiplying the ____________________ total by the ____________________ total and dividing by the sample size, n.
Free
(Short Answer)
4.8/5  (35)
(35)
Correct Answer:
row; column
column; row
Student Absenteeism
Consider a multinomial experiment involving n = 200 students of a large high school.The attendance department recorded the number of students who were absent during the weekdays.The null hypothesis to be tested is: H0: p1 = .10, p2 = .25, p3 = .30, p4 = .20, p5 = .15.
-{Student Absenteeism Narrative} Test the hypothesis at the 5% level of significance with the following frequencies: (n = 50)

Free
(Essay)
4.9/5  (29)
(29)
Correct Answer:
Rejection region: 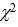 > 11ef175b_ad29_b853_8934_438bbc7f59db_TB7453_11 .05,4 = 9.488
> 11ef175b_ad29_b853_8934_438bbc7f59db_TB7453_11 .05,4 = 9.488
Test statistic: 11ef175b_ad29_b853_8934_438bbc7f59db_TB7453_11= 1.147
Conclusion: Cannot reject the null hypothesis.There is not enough evidence to conclude that absenteeism patterns differ throughout the week.
The expected values of a contingency table are calculated based on the assumption that H0 is ____________________.
Free
(Short Answer)
4.8/5  (30)
(30)
Correct Answer:
true
accepted
A multinomial experiment with two categories is identical to a binomial experiment.
(True/False)
4.8/5  (31)
(31)
The only way the chi-squared test statistic can be zero is if the observed frequencies are all exactly the same as the expected frequencies.
(True/False)
4.8/5  (39)
(39)
To address whether two variables are related in a contingency table, the alternative hypothesis, H1, is:
(Multiple Choice)
4.8/5  (35)
(35)
A Deli proposes to serve 4 main Sandwiches.For planning purposes, the manager expects that the proportions of each that will be selected by her customers will be:
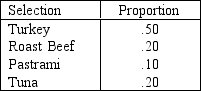 Of a random sample of 100 customers, 44 selected chicken, 24 selected roast beef, 13 selected Pastrami, and 10 selected tuna.Should the manager revise her estimates? Use = .01.
Of a random sample of 100 customers, 44 selected chicken, 24 selected roast beef, 13 selected Pastrami, and 10 selected tuna.Should the manager revise her estimates? Use = .01.
(Essay)
4.7/5  (33)
(33)
A chi-squared test of a contingency table with 6 degrees of freedom results in a test statistic 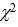 = 13.58.Using the 11ef175e_802b_4a04_8934_59fb895fb22d_TB7453_11 tables, the most accurate statement that can be made about the p-value for this test is that:
= 13.58.Using the 11ef175e_802b_4a04_8934_59fb895fb22d_TB7453_11 tables, the most accurate statement that can be made about the p-value for this test is that:
(Multiple Choice)
4.8/5  (34)
(34)
The sampling distribution of the test statistic for a goodness-of-fit test with k categories is a:
(Multiple Choice)
4.8/5  (32)
(32)
A test for whether one proportion is higher than the other can be performed using the chi-squared distribution.
(True/False)
4.8/5  (39)
(39)
Consider a multinomial experiment involving 160 trials 4 categories (cells).The observed frequencies resulting from the experiment are shown in the accompanying table.
 Use the 10% significance level to test the hypotheses: H0: p1 = p2 = p3 = p4 = .25 vs.H1: At least one proportion differs from their specified values.
Use the 10% significance level to test the hypotheses: H0: p1 = p2 = p3 = p4 = .25 vs.H1: At least one proportion differs from their specified values.
(Essay)
4.8/5  (36)
(36)
If you want to compare two populations that each have more than two categories, you can use a chi-squared test of a(n) ____________________.
(Short Answer)
4.9/5  (33)
(33)
Before a chi-squared test of a contingency table can be carried out, the expected values of all cells should be greater than or equal to ____________________.
(Short Answer)
4.9/5  (35)
(35)
Mathematical statisticians have established that if we square the value of z, the test statistic for the test of one proportion p, we produce the 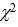 statistic.That is, z2 = 11ef175f_648a_6c86_8934_c9eca761ba5b_TB7453_11.
statistic.That is, z2 = 11ef175f_648a_6c86_8934_c9eca761ba5b_TB7453_11.
(True/False)
4.8/5  (41)
(41)
A test statistic that lies in the far right tail of the chi-squared distribution indicates you will ____________________ H0.
(Short Answer)
4.9/5  (33)
(33)
Suppose that two shipping companies, A and B, each decide to estimate the annual percentage of shipments on which a $100 or greater claim for loss or damage was filed by sampling their records, and they report the data shown below.  The owner of Company B is hoping to use these data to show that her company is superior to Company A with regard to the percentage of claims filed.Which test would be used to properly analyze the data in this experiment?
The owner of Company B is hoping to use these data to show that her company is superior to Company A with regard to the percentage of claims filed.Which test would be used to properly analyze the data in this experiment?
(Multiple Choice)
4.9/5  (30)
(30)
To determine the critical values in the chi-squared distribution table, you need to know the:
(Multiple Choice)
4.8/5  (35)
(35)
The number of degrees of freedom associated with the chi-squared test statistic for normality is the number of ____________________ minus 1 minus the number of ____________________ estimated.
(Short Answer)
4.8/5  (41)
(41)
If we want to perform a two-tail test for differences between two populations of nominal data with exactly two categories, we can employ either the z-test of p1 - p2, or the chi-squared test of a contingency table.
(True/False)
4.8/5  (33)
(33)
Showing 1 - 20 of 175
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)