Exam 14: Analysis of Variance

Keyboard Configuration and Size The data shown below were taken from a 2 1 x 3 factorial experiment to examine the effects of factor A (keyboard configuration, 3 levels) and factor B (keyboard size, 2 levels) on typing speed.Each cell consists of the times needed for each of 4 randomly assigned keyboardists to type a standard document under each set of conditions (in minutes). 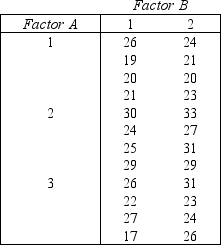-{Keyboard Configuration and Size Narrative} Test at the 5% significance level to determine if time differences exist among the different keyboard configurations.

Fisher's least significant difference method (LSD) substitutes the pooled variance estimator from the equal variances t-test with the MSE from ANOVA.

When the F-test is used for ANOVA, the rejection region is always in the right tail.

The F-test statistic in a one-way ANOVA is equal to:

Consider the following partial ANOVA table: 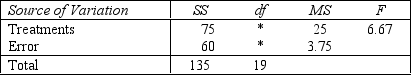The numerator and denominator degrees of freedom for the F-test (identified by asterisks) are

Consider the following ANOVA table: The number of treatments is

The F-test of a randomized block design of the analysis of variance has the same requirements as the independent samples design; that is, the random variable must be ____________________ distributed and the population ____________________ must be equal.

When the effect of a level for one factor depends on which level of another factor is present, the most appropriate ANOVA design to use in this situation is the:

In the two-factor ANOVA where a is the number of factor A levels, b is the number of factor B levels, r is the number of replicates, and n is the total number of observations, the number of degrees of freedom for error is:

One-way ANOVA is applied to independent samples taken from four normally distributed populations with equal variances.If the null hypothesis is rejected, then we can infer that

Health Inspectors' Ages In order to examine the differences in ages of health inspectors among five counties, a Health Department statistician took random samples of six inspectors' ages in each county.The data are listed below.An F-test using ANOVA showed that average age differs for at least two counties. 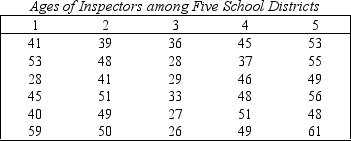-{Health Inspectors' Ages Narrative} Did Tukey's method and Fisher's LSD method in the previous two questions yield the same results? Will this always be the case?

The randomized block design with exactly two treatments is equivalent to a two-tail:

In one-way analysis of variance, between-treatments variation is measured by the:

Sub Sandwich Customers The marketing manager of a Sub Shop chain is in the process of examining some of the demographic characteristics of her customers.In particular, she would like to investigate the belief that the ages of the customers of Sub Shops, hamburger emporiums, and fast-food chicken restaurants are different.The ages of eight randomly selected customers of each of the restaurants are recorded and listed below.From previous analyses we know that the ages are normally distributed with equal variances for each group. 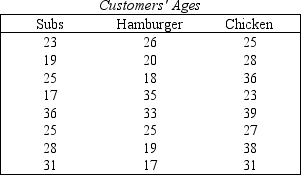-{Sub Sandwich Customers Narrative} Set up the ANOVA Table.Use $\alpha$ = 0.05 to determine the critical value.

The F-statistic in a one-way ANOVA represents the variation within the treatments divided by the variation between the treatments.

In a two-factor ANOVA, where a is the number of factor A levels and b is the number of factor B levels, the number of degrees of freedom for the interaction term is

In a completely randomized design for ANOVA, the numerator and denominator degrees of freedom are 4 and 25, respectively.The total number of observations must equal:

The within-treatments variation provides a measure of the amount of variation in the response variables that is caused by the treatments.

Health Inspectors' Ages In order to examine the differences in ages of health inspectors among five counties, a Health Department statistician took random samples of six inspectors' ages in each county.The data are listed below.An F-test using ANOVA showed that average age differs for at least two counties. -{Health Inspectors' Ages Narrative} Use Tukey's multiple comparison method to determine which means differ.

GMAT Scores A recent college graduate is in the process of deciding which one of three graduate schools he should apply to.He decides to judge the quality of the schools on the basis of the Graduate Management Admission Test (GMAT) scores of those who are accepted into the school.A random sample of six students in each school produced the following GMAT scores.Assume that the data are normally distributed with equal variances for each school. 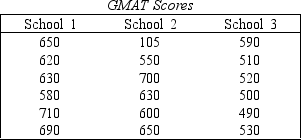-{GMAT Scores Narrative} Can he infer at the 10% significance level that the GMAT scores differ among the three schools?