Exam 12: Introduction to Simulation Using Risk Solver Platform

A simulation model was replicated 100 times yielding a mean of 82.59 with variance of 17.66. Of the 100 replications, 11 replications yielded an outcome over a value of 100. The 95% confidence interval of the mean is the interval (81.77, 83.41). Of the 100 simulation outcomes, 65 outcomes failed to fall within this interval. What is wrong with the confidence interval?

Exhibit 12.4. The following questions use the information below. The manager of a Washington, DC sightseeing tour company is concerned about overbooking for one of his bus tours. The bus has 15 seats but sometimes there are empty seats. His records show that about 20% of ticket holders do not show up for their tour. Tickets cost $10 and are non-refundable. If the manager overbooks the tour and more than 15 passengers show up, some of them will be bumped to a later tour. This bumping costs the company $25 in various expenses to keep the customer happy until the next tour. The manager wants to see what happens to profits if 18 reservations are accepted. 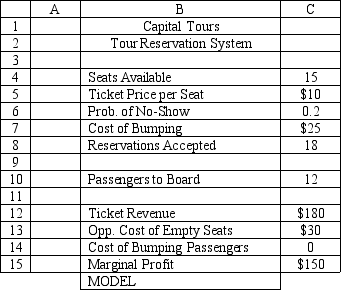-Using the information in Exhibit 12.4, what formula should go in cell C15 of the worksheet to determine the Marginal Profit?

What function should be used for generating random numbers from a normal distribution with mean  and standard deviation ?

Exhibit 12.3 The following questions use the information below. An auto parts store wants to simulate its inventory system for engine oil. The company has collected data on the shipping time for oil and the daily demand for cases of oil. A case of oil generates a $10 profit. Customers can buy oil at any auto parts store so there are no backorders (the company loses the sale and profit). The company orders 30 cases whenever the inventory position falls below the reorder point of 15 cases. Orders are placed at the beginning of the day and delivered at the beginning of the day so the oil is available on the arrival day. An average service level of 99% is desired. The following spreadsheets have been developed for this problem. The company has simulated 2 weeks of operation for their inventory system. The current level of on-hand inventory is 25 units and no orders are pending. 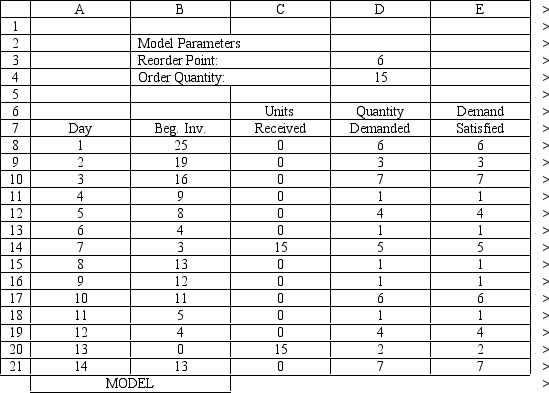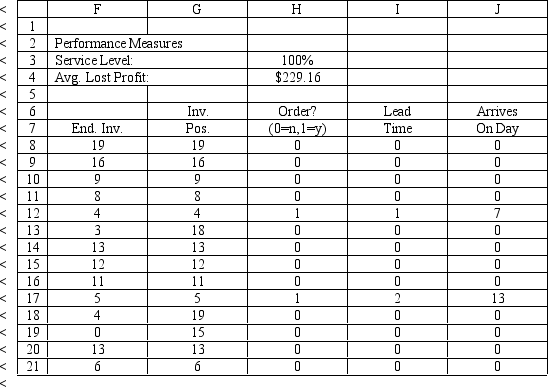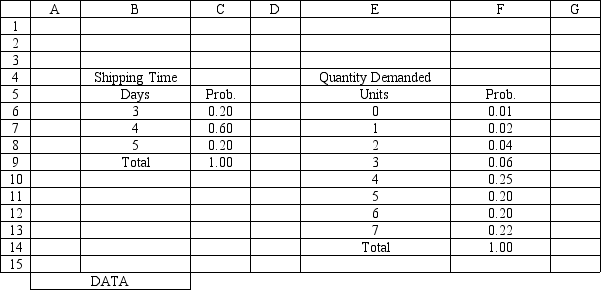-Using the information in Exhibit 12.3, what formula should go in cell C9 and copied to C10:C21 of the MODEL sheet to compute units received?

Exhibit 12.2 The following questions use the information below. The owner of Fix-a-dent Auto Repair wants to study the growth of his business using simulation. He is interested in simulating the number of damaged cars and the amount of damage to the cars each month. He currently repairs 100 cars per month and feels the change in number of cars can vary uniformly between a decrease of as much as 3% and an increase of up to 5% (average change of 1%). The dollar value of the damage to the cars is a normally distributed random variable with a mean of $3,000 and a standard deviation of $500. The average repair bill has been increasing steadily over the years and the owner expects the mean repair bill will increase by 1% per month. A spreadsheet model to simulate the problem has been run 300 times. A part of the simulation statistics output from Risk Solver Platform (RSP)and a spreadsheet for computing confidence intervals follows. Simulation Statistics Name Income/Revenue Description Output Cell D21 Minimum= 3339249.82 Maximum= 5086714.77 Mean= 4119518.91 Std Deviation= 291116.83 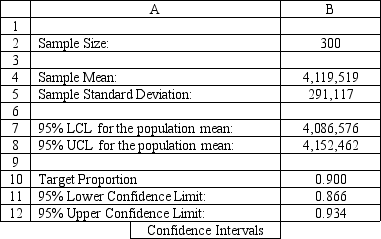-Using the information in Exhibit 12.2, what is the worst case scenario for the Fix-a-dent company based on this output?

Exhibit 12.4. The following questions use the information below. The manager of a Washington, DC sightseeing tour company is concerned about overbooking for one of his bus tours. The bus has 15 seats but sometimes there are empty seats. His records show that about 20% of ticket holders do not show up for their tour. Tickets cost $10 and are non-refundable. If the manager overbooks the tour and more than 15 passengers show up, some of them will be bumped to a later tour. This bumping costs the company $25 in various expenses to keep the customer happy until the next tour. The manager wants to see what happens to profits if 18 reservations are accepted. -Using the information in Exhibit 12.4, what formula should go in cell C14 of the worksheet to determine the Cost of Bumping Passengers?

Exhibit 12.5 The following questions use the information below. The owner of Sal's Italian Restaurant wants to study the growth of his business using simulation. He is interested in simulating the number of customers and the amount ordered by customers each month. He currently serves 1000 customers per month and feels this can vary uniformly between a decrease of as much as 5% and an increase of up to 9%. The bill for each customer is a normally distributed random variable with a mean of $20 and a standard deviation of $5. The average order has been increasing steadily over the years and the owner expects the mean order will increase by 2% per month. You have created the following spreadsheet to simulate the problem. 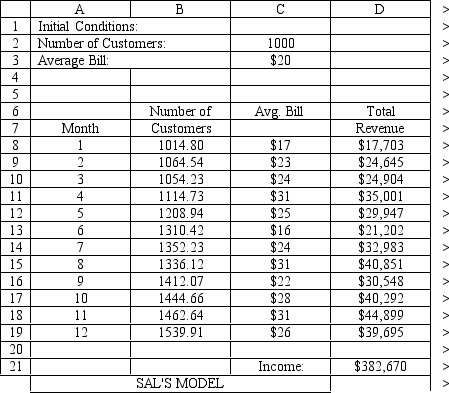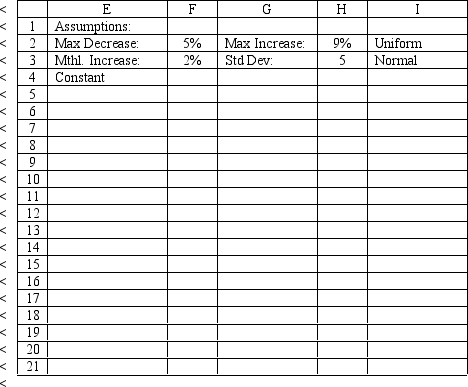-Sal, from Exhibit 12.5, has produced the following spreadsheet to compute confidence intervals on his income. What formula should go in cell B12 to compute the upper limit on a 95% confidence interval for the population proportion below 90%? A B 1 2 Sample Size: 300 3 4 Sample Mean: 4,119,519 5 Sample Standard Deviation: 291,117 6 7 95\% LCL far the papulation mean: 4,086,576 8 95\% UCL far the population mean: 4,152,462 9 10 Target Prapartion 0.900 11 95\% Lower Confidence Linnit: 0.866 12 95\% Upper Confidence I.init: 0.934

If chance or uncertainty is present in a system then there is an element of ____ in the decision-making problem.

Project 12.2  Friendly Bank Services Friendly Bank has various branches throughout the city. Ronnie Fare is a branch manager. Ronnie has always been very customer focused. Her branch is relatively small; three counter tellers and two drive-through tellers. Her drive-through support appears more than adequate. Her concern is with the in-branch service. She is concerned with the level of service being provided and whether or not each of her three tellers are of equal proficiency. When a customer comes in for service, they wait in a single line and move to the first available teller. Once serviced, the customer leaves the bank. Six weeks ago, Ronnie enlisted a small company to conduct time studies of the bank processes. The data was collected over a four-week period. This extended collection period helped overcome bias in the data collection due to observation activities. The final set of data just arrived in the mail and Ronnie is ready to conduct some desk-top analysis of the data to answer her own questions about her branch. The data pertinent to her concerns are: Customer inter-arrivals: Uniformly distributed between 1 and 3 minutes. Average of 250 arrivals per day. In-bank teller performance (service time): Teller 1: Normally distributed, mean 5 minutes, standard deviation 1.2 minutes Teller 2: Normally distributed, mean 3 minutes, standard deviation 1.1 minutes Teller 3: Normally distributed, mean 4.2 minutes, standard deviation 1.5 minute: (The minimum service time observed was 30 seconds despite the distribution fit) At first glance, it appears there is no difference between the tellers. However, Ronnie wants to simulate the bank process to provide estimates on the following pieces of information: Customer Information: Average wait time per customer. Average time in bank per customer. Percentage of time the customer does not wait Percentage of time the customer waits less than a minute How often, if at all the entire work day experiences no waiting lines Teller Performance Information: Average utilization per day. Average number of customers seen per day. Build a simulation model to help answer Ronnie Fare's questions. Use 250 arrivals per day. Provide appropriate statistical information in your answer.

A simulation model was replicated 100 times yielding a mean of 82.59 with variance of 17.66. Of the 100 replications, 11 replications yielded an outcome over a value of 100. What is the 90% confidence interval on the mean?

Exhibit 12.4. The following questions use the information below. The manager of a Washington, DC sightseeing tour company is concerned about overbooking for one of his bus tours. The bus has 15 seats but sometimes there are empty seats. His records show that about 20% of ticket holders do not show up for their tour. Tickets cost $10 and are non-refundable. If the manager overbooks the tour and more than 15 passengers show up, some of them will be bumped to a later tour. This bumping costs the company $25 in various expenses to keep the customer happy until the next tour. The manager wants to see what happens to profits if 18 reservations are accepted. -Using the information in Exhibit 12.4, what Risk Solver Platform (RSP) function should be used in cell C10 to determine the number of Passengers to Board?

A variable whose value cannot be predicted or set with certainty is a

A simulation model was replicated 100 times yielding a mean of 82.59 with variance of 17.66. Of the 100 replications, 11 replications yielded an outcome over a value of 100. What is the 95% confidence interval on the proportion of simulations whose outcomes exceeded a value of 100?

Why would a manager be interested in analyzing risk?

Which of the following do not help determine the magnitude of risk in a decision-making problem?

Exhibit 12.5 The following questions use the information below. The owner of Sal's Italian Restaurant wants to study the growth of his business using simulation. He is interested in simulating the number of customers and the amount ordered by customers each month. He currently serves 1000 customers per month and feels this can vary uniformly between a decrease of as much as 5% and an increase of up to 9%. The bill for each customer is a normally distributed random variable with a mean of $20 and a standard deviation of $5. The average order has been increasing steadily over the years and the owner expects the mean order will increase by 2% per month. You have created the following spreadsheet to simulate the problem. -Using the information in Exhibit 12.5 and the Risk Solver Platform (RSP), what formulas should go in cells B8:D21 of the spreadsheet?

Inventory position is defined as

What function should be used for generating random numbers between a and b from a symmetric triangular distribution when the most likely value is (a + b)/2?

How should one determine which RNGs to employ in a spreadsheet simulation model?

What function should be used for generating random numbers between 1 and 12 from a continuous uniform distribution?