Exam 15: Multiple Regression
Exam 1: Data and Statistics84 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Displays67 Questions
Exam 3: Descriptive Statistics: Numerical Measures127 Questions
Exam 4: Introduction to Probability99 Questions
Exam 5: Discrete Probability Distributions86 Questions
Exam 6: Continuous Probability Distributions120 Questions
Exam 7: Sampling and Sampling Distributions117 Questions
Exam 8: Interval Estimation144 Questions
Exam 9: Hypothesis Tests129 Questions
Exam 10: Inference About Means and Proportions With Two Populations85 Questions
Exam 11: Inferences About Population Variances85 Questions
Exam 12: Comparing Multiple Proportions, Tests of Independence and Goodness of Fit59 Questions
Exam 13: Experimental Design and Analysis of Variance80 Questions
Exam 14: Simple Linear Regression131 Questions
Exam 15: Multiple Regression103 Questions
Select questions type
In a multiple regression model involving 44 observations, the following estimated regression equation was obtained.  = 50 + 13x1 + 40x2 + 68x3
For this model, SSR = 600 and SSE = 400.The computed F statistic for testing the significance of the above model is
= 50 + 13x1 + 40x2 + 68x3
For this model, SSR = 600 and SSE = 400.The computed F statistic for testing the significance of the above model is
(Multiple Choice)
4.8/5  (29)
(29)
The following estimated regression equation was developed relating yearly income (y in $1000s) of 30 individuals with their age (x1) and their gender (x2) (0 if male and 1 if female).  = 30 + .7x1 + 3x2
Also provided are SST = 1200 and SSE = 384.The multiple coefficient of determination is
= 30 + .7x1 + 3x2
Also provided are SST = 1200 and SSE = 384.The multiple coefficient of determination is
(Multiple Choice)
4.8/5  (34)
(34)
The following estimated regression equation was developed relating yearly income (y in $1000s) of 30 individuals with their age (x1) and their gender (x2) (0 if male and 1 if female). 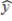 = 30 + .7x1 + 3x2
Also provided are SST = 1200 and SSE = 384.The yearly income (in $) expected of a 24-year-old male individual is
= 30 + .7x1 + 3x2
Also provided are SST = 1200 and SSE = 384.The yearly income (in $) expected of a 24-year-old male individual is
(Multiple Choice)
4.9/5  (37)
(37)
Below you are given a partial computer output from a multiple regression analysis based on a sample of 16 observations. 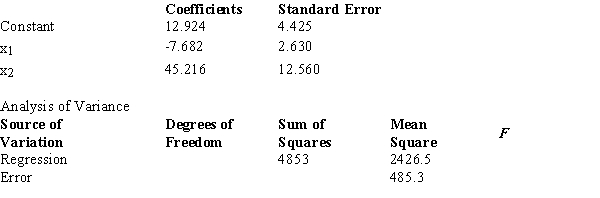 Carry out the test of significance for the parameter β1 at the 1% level.The null hypothesis should
Carry out the test of significance for the parameter β1 at the 1% level.The null hypothesis should
(Multiple Choice)
4.7/5  (33)
(33)
A regression analysis involved 5 independent variables and 99 observations.The critical value of t for testing the significance of each of the independent variable's coefficients will have _____ degrees of freedom.
(Multiple Choice)
4.8/5  (43)
(43)
In a multiple regression analysis, SSR = 1000 and SSE = 200.The multiple coefficient of determination is
(Multiple Choice)
4.8/5  (36)
(36)
Below you are given a partial computer output from a multiple regression analysis based on a sample of 16 observations. 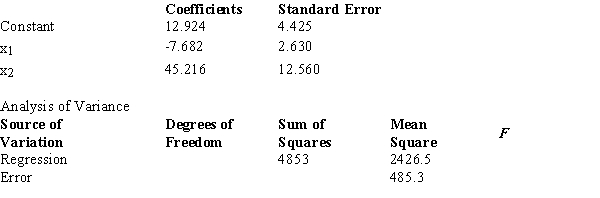 The sum of squares due to error (SSE) equals
The sum of squares due to error (SSE) equals
(Multiple Choice)
4.9/5  (36)
(36)
A regression analysis involved 17 independent variables and 697 observations.The critical value of t for testing the significance of each of the independent variable's coefficients will have
(Multiple Choice)
4.7/5  (31)
(31)
In order to test for the significance of a regression model involving 3 independent variables and 47 observations, the numerator and denominator degrees of freedom (respectively) for the critical value of F are
(Multiple Choice)
4.8/5  (38)
(38)
In order to test for the significance of a regression model involving 10 independent variables and 100 observations, the numerator and denominator degrees of freedom (respectively) for the critical value of F are
(Multiple Choice)
4.7/5  (40)
(40)
A regression model involving 4 independent variables and a sample of 15 observations resulted in the following sum of squares. SSR = 165
SSE = 60
The multiple coefficient of determination is
(Multiple Choice)
4.9/5  (22)
(22)
Determine if the model is significant based upon the data given at α = .01. 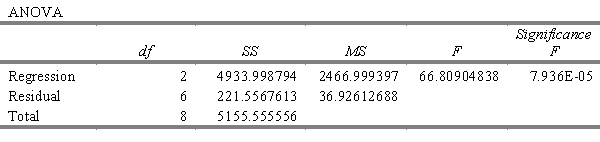
(Essay)
4.9/5  (37)
(37)
A regression analysis involved 2 independent variables and 27 observations.The critical value of t for testing the significance of each of the independent variable's coefficients will have _____ degrees of freedom.
(Multiple Choice)
4.9/5  (34)
(34)
A regression model between sales (y in $1000), unit price (x1 in dollars), and television advertisement (x2 in dollars) resulted in the following function:  = 7 - 4x1 + 5x2
For this model, SSR = 3500, SSE = 1500, and the sample size is 20.The adjusted multiple coefficient of determination for this problem is
= 7 - 4x1 + 5x2
For this model, SSR = 3500, SSE = 1500, and the sample size is 20.The adjusted multiple coefficient of determination for this problem is
(Multiple Choice)
4.8/5  (33)
(33)
Below you are given a partial computer output from a multiple regression analysis based on a sample of 16 observations. 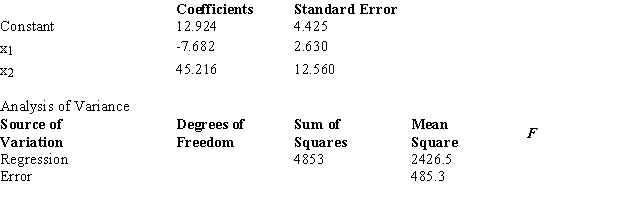 The t value obtained from the table which is used to test an individual parameter at the 1% level is
The t value obtained from the table which is used to test an individual parameter at the 1% level is
(Multiple Choice)
4.9/5  (30)
(30)
Below you are given a partial computer output from a multiple regression analysis based on a sample of 16 observations. 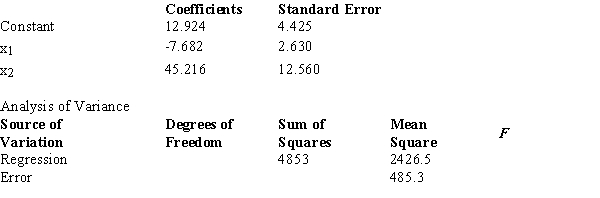 The degrees of freedom for the sum of squares explained by the regression (SSR) are
The degrees of freedom for the sum of squares explained by the regression (SSR) are
(Multiple Choice)
4.8/5  (33)
(33)
In a multiple regression model involving 44 observations, the following estimated regression equation was obtained: 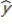 = 30 + 18x1 + 43x2 + 87x3
For this model, SSR = 800 and SST = 1400.The multiple correlation coefficient for the above model is
= 30 + 18x1 + 43x2 + 87x3
For this model, SSR = 800 and SST = 1400.The multiple correlation coefficient for the above model is
(Multiple Choice)
4.9/5  (29)
(29)
Showing 41 - 60 of 103
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)