Exam 11: Inferences About Population Variances
Exam 1: Data and Statistics84 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Displays67 Questions
Exam 3: Descriptive Statistics: Numerical Measures118 Questions
Exam 4: Introduction to Probability94 Questions
Exam 5: Discrete Probability Distributions84 Questions
Exam 6: Continuous Probability Distributions121 Questions
Exam 7: Sampling and Sampling Distributions116 Questions
Exam 8: Interval Estimation90 Questions
Exam 9: Hypothesis Tests95 Questions
Exam 10: Inference About Means and Proportions With Two Populations63 Questions
Exam 11: Inferences About Population Variances66 Questions
Exam 12: Comparing Multiple Proportions, Tests of Independence and Goodness of Fit59 Questions
Exam 13: Experimental Design and Analysis of Variance76 Questions
Exam 14: Simple Linear Regression132 Questions
Exam 15: Multiple Regression103 Questions
Exam 16: Regression Analysis: Model Building41 Questions
Exam 17: Time Series Analysis and Forecasting51 Questions
Exam 18: Nonparametric Methods58 Questions
Exam 19: Decision Analysis48 Questions
Exam 20: Index Numbers39 Questions
Exam 21: Statistical Methods for Quality Control60 Questions
Exam 22: Sample Survey48 Questions
Select questions type
The symbol used for the variance of the population is
Free
(Multiple Choice)
5.0/5  (29)
(29)
Correct Answer:
B
A sample of 28 elements is selected to estimate a 95% confidence interval for the variance of the population. The chi-square values to be used for this interval estimation are
Free
(Multiple Choice)
4.9/5  (34)
(34)
Correct Answer:
B
A sample of 31 observations yielded a sample variance of 16. If we want to test H0: σ2 = 16, the test statistic is
Free
(Multiple Choice)
4.8/5  (29)
(29)
Correct Answer:
C
Based on the sample evidence below, we want to test the hypothesis that population A has a larger variance than population B.  The test statistic for this problem equals
The test statistic for this problem equals
(Multiple Choice)
4.9/5  (35)
(35)
Consider the following hypothesis problem.  The null hypothesis is to be tested at the 5% level of significance. The critical value(s) from the chi-square distribution table is(are)
The null hypothesis is to be tested at the 5% level of significance. The critical value(s) from the chi-square distribution table is(are)
(Multiple Choice)
4.9/5  (34)
(34)
A sample of 60 items from population 1 has a sample variance of 12 while a sample of 50 items from population 2 has a sample variance of 15. If we want to test whether the variances of the two populations are equal, the test statistic will have a value of
(Multiple Choice)
4.7/5  (32)
(32)
Consider the following hypothesis problem. 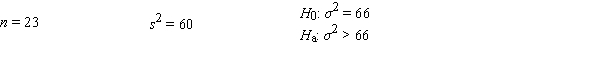 The test statistic has a value of
The test statistic has a value of
(Multiple Choice)
4.9/5  (36)
(36)
Consider the following hypothesis problem.  At the 5% level of significance, the null hypothesis
At the 5% level of significance, the null hypothesis
(Multiple Choice)
5.0/5  (37)
(37)
Consider the following hypothesis problem.  At the 5% level of significance, the null hypothesis
At the 5% level of significance, the null hypothesis
(Multiple Choice)
4.8/5  (47)
(47)
The sampling distribution of the ratio of independent sample variances extracted from two normal populations with equal variances is the
(Multiple Choice)
4.9/5  (32)
(32)
We are interested in testing to see if the variance of a population is less than 7. The correct null hypothesis is
(Multiple Choice)
4.9/5  (35)
(35)
The sampling distribution of the quantity (n - 1)s2/σ2 is the
(Multiple Choice)
4.9/5  (33)
(33)
Consider the following hypothesis problem. 
The p-value is
The p-value is
(Multiple Choice)
4.8/5  (38)
(38)
The bottler of a certain soft drink claims their equipment to be accurate and that the variance of all filled bottles is at most .05. The null hypothesis in a test to confirm the claim would be written as
(Multiple Choice)
4.9/5  (33)
(33)
Consider the following hypothesis problem. 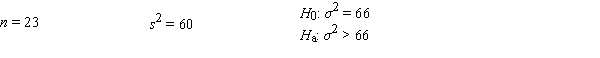
If the test is to be performed at the 5% level, the critical value(s) from the chi-square distribution table is(are)
If the test is to be performed at the 5% level, the critical value(s) from the chi-square distribution table is(are)
(Multiple Choice)
4.8/5  (42)
(42)
The standard deviation of the daily temperatures in Honolulu last year was 4 degrees Fahrenheit. A random sample of 25 days resulted in a standard deviation of 5.8 degrees Fahrenheit. Has there been a significant increase in the variance of the temperatures? Use α = .05.
(Essay)
4.8/5  (28)
(28)
The contents of a sample of 26 cans of apple juice showed a standard deviation of .06 ounces. We are interested in testing whether the variance of the population is significantly more than .003. The null hypothesis is
(Multiple Choice)
4.9/5  (29)
(29)
Showing 1 - 20 of 66
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)