Exam 12: Comparing Multiple Proportions, Tests of Independence and Goodness of Fit

In the past, 35% of the students at ABC University were in the Business College, 35% of the students were in the Liberal Arts College, and 30% of the students were in the Education College. To see whether or not the proportions have changed, a sample of 300 students from the university was taken. Ninety of the sample students are in the Business College, 120 are in the Liberal Arts College, and 90 are in the Education College. The calculated value for the test statistic equals

A group of 2000 individuals from 3 different cities were asked whether they owned a foreign or a domestic car. The following table shows the results of the survey. ​ At α = .01 using the p-value approach, test to determine if the type of car purchased is independent of the city in which the purchasers live.

During the first few weeks of the new television season, the evening news audience proportions were recorded as ABC - 31%, CBS - 34%, and NBC - 35%. A sample of 600 homes taken recently yielded the following viewing audience data. ​ We want to determine whether or not there has been a significant change in the proportion of viewing audience of the three networks. a. State the null and alternative hypotheses to be tested. b. Compute the expected frequencies. c. Compute the test statistic. d. The null hypothesis is to be tested at the .05 level of significance. Determine the critical value for this test. What do you conclude? e. Determine the p-value and perform the test.

Last school year, the student body of a local university consisted of 30% freshmen, 24% sophomores, 26% juniors, and 20% seniors. A sample of 400 students taken from this year's student body showed the following number of students in each classification. ​ We are interested in determining whether or not there has been a significant change in the classifications between the last school year and this school year. The expected frequency of seniors is

You want to test whether or not the following sample of 30 observations follows a normal distribution. The mean of the sample equals 11.83 and the standard deviation equals 4.53. ​ At the 5% level of significance, the conclusion of the test is that the

The number of emergency calls per day at a hospital over a period of 120 days is shown below. ​ Use α = .05 and the p-value approach to see if the above data have a Poisson distribution.

The degrees of freedom for a table with 7 rows and 4 columns is

In the past, 35% of the students at ABC University were in the Business College, 35% of the students were in the Liberal Arts College, and 30% of the students were in the Education College. To see whether or not the proportions have changed, a sample of 300 students from the university was taken. Ninety of the sample students are in the Business College, 120 are in the Liberal Arts College, and 90 are in the Education College. This problem is an example of a

A group of 500 individuals were asked to cast their votes regarding a particular issue of the Equal Rights Amendment. The following table shows the results of the votes: ​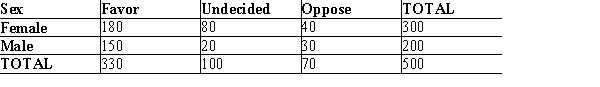 At α = .05 using the p-value approach, test to determine if the votes cast were independent of the sex of the individuals.

The table below gives beverage preferences for random samples of teens and adults. ​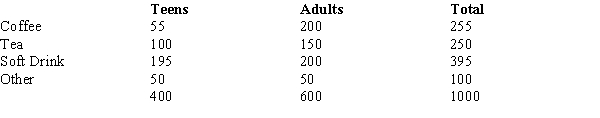 We are asked to test for independence between age (i.e., adult and teen) and drink preferences. The test statistic for this test of independence is

The owner of a car wash wants to see if the arrival rate of cars follows a Poisson distribution. In order to test the assumption of a Poisson distribution, a random sample of 150 ten-minute intervals was taken. You are given the following observed frequencies: ​ At the .05 level of significance, the conclusion of the test is that the

A statistical test conducted to determine whether to reject or not reject a hypothesized probability distribution for a population is known as a _____ test.

The number of categorical outcomes per trial for a multinomial probability distribution is​

If there are three or more populations, then it is

Marascuilo procedure is used to test for a significant difference between pairs of population

Before the rush began for Christmas shopping, a department store had noted that the percentage of its customers who use the store's credit card, the percentage of those who use a major credit card, and the percentage of those who pay cash are the same. During the Christmas rush, in a sample of 210 shoppers, 66 used the store's credit card; 63 used a major credit card; and 81 paid cash. With α = .05, test to see if the methods of payment have changed during the Christmas rush.

In the past, 35% of the students at ABC University were in the Business College, 35% of the students were in the Liberal Arts College, and 30% of the students were in the Education College. To see whether or not the proportions have changed, a sample of 300 students from the university was taken. Ninety of the sample students are in the Business College, 120 are in the Liberal Arts College, and 90 are in the Education College. The expected frequency for the Business College is

A population where each of its element is assigned to one and only one of several classes or categories is a _____ population.

When individuals in a sample of 150 were asked whether or not they supported capital punishment, the following information was obtained. ​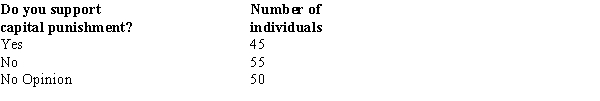 We are interested in determining whether or not the opinions of the individuals (as to Yes, No, and No Opinion) are uniformly distributed. The conclusion of the test at the 5% level of significance is that the

The owner of a car wash wants to see if the arrival rate of cars follows a Poisson distribution. In order to test the assumption of a Poisson distribution, a random sample of 150 ten-minute intervals was taken. You are given the following observed frequencies: ​ Calculate mean and use Poisson probabilities. The expected frequency of exactly 3 cars arriving in a 10-minute interval is