Exam 6: Continuous Probability Distributions
Exam 1: Data and Statistics84 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Displays67 Questions
Exam 3: Descriptive Statistics: Numerical Measures118 Questions
Exam 4: Introduction to Probability94 Questions
Exam 5: Discrete Probability Distributions84 Questions
Exam 6: Continuous Probability Distributions121 Questions
Exam 7: Sampling and Sampling Distributions116 Questions
Exam 8: Interval Estimation90 Questions
Exam 9: Hypothesis Tests95 Questions
Exam 10: Inference About Means and Proportions With Two Populations63 Questions
Exam 11: Inferences About Population Variances66 Questions
Exam 12: Comparing Multiple Proportions, Tests of Independence and Goodness of Fit59 Questions
Exam 13: Experimental Design and Analysis of Variance76 Questions
Exam 14: Simple Linear Regression132 Questions
Exam 15: Multiple Regression103 Questions
Exam 16: Regression Analysis: Model Building41 Questions
Exam 17: Time Series Analysis and Forecasting51 Questions
Exam 18: Nonparametric Methods58 Questions
Exam 19: Decision Analysis48 Questions
Exam 20: Index Numbers39 Questions
Exam 21: Statistical Methods for Quality Control60 Questions
Exam 22: Sample Survey48 Questions
Select questions type
For a continuous random variable x, the probability density function f(x) represents the
(Multiple Choice)
5.0/5  (31)
(31)
The travel time for a college student traveling between her home and her college is uniformly distributed between 40 and 90 minutes. The probability that her trip will take exactly 50 minutes is
(Multiple Choice)
4.9/5  (35)
(35)
Given that z is a standard normal random variable, what is the value of z if the area to the right of z is 0.5?
(Multiple Choice)
4.9/5  (34)
(34)
Given that z is a standard normal random variable, what is the value of z if the area between -z and z is 0.9812?
(Multiple Choice)
4.7/5  (35)
(35)
Whenever the probability is proportional to the length of the interval in which the random variable can assume a value, the random variable is _____ distributed.
(Multiple Choice)
4.9/5  (32)
(32)
For any continuous random variable, the probability that the random variable takes on exactly a specific value is
(Multiple Choice)
4.8/5  (37)
(37)
What is the mean of x, given the function below? f(x) = (1/10) e-x/10 x  0
0
(Multiple Choice)
4.8/5  (38)
(38)
The travel time for a college student traveling between her home and her college is uniformly distributed between 40 and 90 minutes. The probability that her trip will take longer than 80 minutes is
(Multiple Choice)
4.9/5  (37)
(37)
Given that z is a standard normal random variable, what is the probability that -2.51 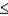 z
z 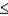 -1.53?
-1.53?
(Multiple Choice)
4.8/5  (34)
(34)
The life expectancy of a particular brand of tire is normally distributed with a mean of 40,000 and a standard deviation of 5,000 miles. What is the probability that a randomly selected tire will have a life of exactly 42,500 miles?
(Multiple Choice)
4.9/5  (30)
(30)
z is a standard normal random variable. The P(-1.96  z
z 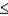 1.4) equals
1.4) equals
(Multiple Choice)
4.9/5  (25)
(25)
A uniform probability distribution is a continuous probability distribution where the probability that the random variable assumes a value in any interval of equal length is
(Multiple Choice)
4.8/5  (40)
(40)
The weight of football players is normally distributed with a mean of 200 pounds and a standard deviation of 25 pounds. The probability of a player weighing less than 240 pounds is
(Multiple Choice)
4.8/5  (27)
(27)
For a standard normal distribution, the probability of obtaining a z value of less than 1.65 is
(Multiple Choice)
4.9/5  (32)
(32)
The time it takes to travel from home to the office is normally distributed with μ = 25 minutes and σ = 5 minutes. What is the probability the trip takes between 20 and 30 minutes?
(Multiple Choice)
4.9/5  (39)
(39)
The time it takes to travel from home to the office is normally distributed with μ = 25 minutes and σ = 5 minutes. What is the probability the trip takes more than 32 minutes?
(Multiple Choice)
4.9/5  (26)
(26)
Showing 61 - 80 of 121
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)