Exam 6: Higher-Degree Polynomial and Rational Functions
Exam 1: Functions, Graphs, and Models; Linear Functions306 Questions
Exam 2: Linear Models, Equations, and Inequalities212 Questions
Exam 3: Quadratic, Piecewise-Defined, and Power Functions261 Questions
Exam 4: Additional Topics With Functions299 Questions
Exam 5: Exponential and Logarithmic Functions304 Questions
Exam 6: Higher-Degree Polynomial and Rational Functions262 Questions
Exam 7: Systems of Equations and Matrices196 Questions
Exam 8: Special Topics in Algebra265 Questions
Select questions type
Suppose c(x) x is the cost of manufacturing x items. Find a production level that will minimize the average cost of making x items.
(Multiple Choice)
4.9/5  (35)
(35)
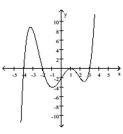
Use the graph of the polynomial function f(x) to solve f(
-
(Multiple Choice)
4.9/5  (26)
(26)
Find the cubic or quartic function that models the data in the table.
- x 3 4 6 8 y 10 15 21 33 start text left parenthesisCubicright parenthesis end text
(Multiple Choice)
4.8/5  (33)
(33)
The Cool Company determines that the supply function for its basic air conditioning unit is and that its demand function is , where is the price. Determine the price for which the supply equals the demand.
(Multiple Choice)
4.8/5  (38)
(38)
State the degree and leading coefficient of the polynomial function.
-
(Multiple Choice)
4.9/5  (35)
(35)
Determine whether the given constant is a solution to the given polynomial equation.
-
(Multiple Choice)
4.8/5  (31)
(31)
Give the equations of any vertical asymptotes for the graphs of the rational functions.
-
(Multiple Choice)
4.8/5  (23)
(23)
Use the graph of f(x) to solve the inequality.
-The profit made when units are sold, , is given by . Determine the number of units to be sold in order for (a profit is made).
(Multiple Choice)
4.8/5  (35)
(35)
Use the graph of the polynomial function f(x) to solve f(
-
(Multiple Choice)
4.9/5  (31)
(31)
The table shows the number of dollars spent in Country X (in millions) on environmental protection programs during the years 2000(x=1) through 2010(x=11) . Find the cubic model that is the best fit for this data. Round coefficients to three decimal places.
Year Spending (millions of dollars) 2000 5.5 2001 5.8 2002 6.1 2003 6.9 2004 7.1 2005 7.0 2006 6.5 2007 7.3 2008 7.6 2009 7.8 2010 7.9
(Multiple Choice)
4.7/5  (37)
(37)
Use factoring and the root method to solve the polynomial equation.
-
(Multiple Choice)
4.8/5  (41)
(41)
Showing 41 - 60 of 262
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)