Exam 7: The Circular Functions and Their Graphs
Exam 1: Review of Basic Concepts639 Questions
Exam 2: Equations and Inequalities496 Questions
Exam 3: Graphs and Functions522 Questions
Exam 4: Polynomial and Rational Functions508 Questions
Exam 5: Inverse, Exponential, and Logarithmic Functions472 Questions
Exam 6: Trigonometric Functions297 Questions
Exam 7: The Circular Functions and Their Graphs286 Questions
Exam 8: Trigonometric Identities and Equations492 Questions
Exam 9: Applications of Trigonometry447 Questions
Exam 10: Systems and Matrices507 Questions
Exam 11: Analytic Geometry217 Questions
Exam 12: Further Topics in Algebra348 Questions
Select questions type
Convert the radian measure to degrees. Give answer using decimal degrees to the nearest hundredth. Us
-
Free
(Multiple Choice)
4.9/5  (31)
(31)
Correct Answer:
D
Find the exact circular function value.
-
Free
(Multiple Choice)
4.9/5  (39)
(39)
Correct Answer:
C
Find the area of a sector of a circle having radius r and central angle . If necessary, express the answer to the nearest
tenth.
-
(Multiple Choice)
4.9/5  (37)
(37)
Find the area of a sector of a circle having radius r and central angle . If necessary, express the answer to the nearest
tenth.
-A pendulum swings through an angle of 16° each second. If the pendulum is 12 cm in length and 62) the complete swing from right to left lasts 2 seconds, what area is covered by each complete swing?
Round to the nearest hundredth.
(Multiple Choice)
4.8/5  (44)
(44)
Determine the equation of the graph.
-Determine the length of a pendulum that has a period of 2 seconds.
(Multiple Choice)
4.9/5  (43)
(43)
Assume that the cities lie on the same north-south line and that the radius of the earth is 6400 km.
-Find the latitude of Spokane, WA if Spokane and Jordan Valley, OR, , are apart.
(Multiple Choice)
5.0/5  (26)
(26)
Assume that the cities lie on the same north-south line and that the radius of the earth is 6400 km.
-The minute hand of a clock is 7 inches long. What distance does its tip move in 24 minutes? Give an exact answer.
(Multiple Choice)
4.8/5  (38)
(38)
Convert the radian measure to degrees. Give answer using decimal degrees to the nearest hundredth. Us
-2
(Multiple Choice)
5.0/5  (35)
(35)
The figure shows an angle in standard position with its terminal side intersecting the unit circle. Evaluate the indicated
circular function value of
-Find
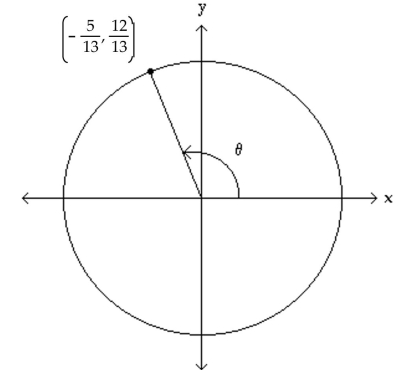
(Multiple Choice)
4.9/5  (32)
(32)
The function graphed is of the form y etermine the equation of the graph.
-The minimum length of a highway sag curve can be computed by
where is the downhill grade in degrees is the uphill grade in degrees is the safe stopping distance for a given speed limit, is the height of the headlights, and is the alignment of the headlights in degrees. Compute L for a 55-mph speed limit, where , , and . Round your answer to the nearest foot.
(Multiple Choice)
4.8/5  (32)
(32)
Use a table or a calculator to evaluate the function. Round to four decimal places.
-
(Multiple Choice)
4.7/5  (37)
(37)
Convert the degree measure to radians, correct to four decimal places.
-
(Multiple Choice)
4.8/5  (34)
(34)
Assume that the cities lie on the same north-south line and that the radius of the earth is 6400 km.
-Suppose the tip of the minute hand of a clock is 3 inches from the center of the clock. Determine the distance traveled by the tip of the minute hand in hours. Give an exact answer.
(Multiple Choice)
4.9/5  (37)
(37)
The function graphed is of the form y Determine the equation of the graph.
-The voltage in an electrical circuit is given by , where is time measured in seconds. Find the frequency of the function (that is, find the number of cycles or periods completed in one second).
(Multiple Choice)
4.8/5  (34)
(34)
Solve the problem
-Let angle POQ be designated . Angles and VRQ are right angles. If , find the length of PQ accurate to four decimal places.
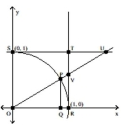
(Multiple Choice)
5.0/5  (36)
(36)
Showing 1 - 20 of 286
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)