Exam 8: Polar Coordinates; Vectors
Exam 1: Functions and Their Graphs297 Questions
Exam 2: Linear and Quadratic Functions302 Questions
Exam 3: Polynomial and Rational Functions354 Questions
Exam 4: Exponential and Logarithmic Functions517 Questions
Exam 5: Trigonometric Functions354 Questions
Exam 6: Analytic Trigonometry342 Questions
Exam 7: Applications of Trigonometric Functions105 Questions
Exam 8: Polar Coordinates; Vectors253 Questions
Exam 9: Analytic Geometry200 Questions
Exam 10: Systems of Equations and Inequalities235 Questions
Exam 11: Sequences; Induction; the Binomial Theorem238 Questions
Exam 12: Counting and Probability115 Questions
Exam 13: A Preview of Calculus: the Limit, Derivative, and Integral of a Function145 Questions
Exam 14: Foundations: a Prelude to Functions234 Questions
Exam 15: Graphing Utilities29 Questions
Select questions type
Write the vector v in the form ai + bj, given its magnitude v and the angle it makes with the positive x-axis.
-
(Multiple Choice)
4.8/5  (30)
(30)
Use the vectors in the figure below to graph the following vector. 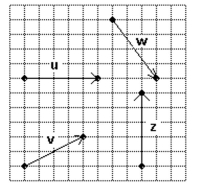 -
-

(Multiple Choice)
4.8/5  (28)
(28)
Find the position vector for the vector having initial point P and terminal point Q.
- and
(Multiple Choice)
4.9/5  (38)
(38)
Write the vector v in the form ai + bj, given its magnitude v and the angle it makes with the positive x-axis.
-
(Multiple Choice)
4.8/5  (33)
(33)
Choose the one alternative that best completes the statement or answers the question.
Find the direction angles of the vector. Round to the nearest degree, if necessary.
-
(Multiple Choice)
5.0/5  (40)
(40)
Use the given vectors to find the indicated expression.
-
Find .
(Multiple Choice)
4.9/5  (24)
(24)
Find the angle between v and w. Round to one decimal place, if necessary.
- and
(Multiple Choice)
4.8/5  (34)
(34)
Test the equation for symmetry with respect to the given axis, line, or pole.
- the line
(Multiple Choice)
4.9/5  (34)
(34)
Solve the problem. Leave your answer in polar form.
- z=2+2i w=-i
Find zw.
(Multiple Choice)
4.8/5  (29)
(29)
Decompose v into two vectors v1 and v2, where v1 is parallel to w and v2 is orthogonal to w.
-
(Multiple Choice)
4.8/5  (33)
(33)
Use the given vectors to find the indicated expression.
-
Find .
(Multiple Choice)
4.9/5  (32)
(32)
Write the vector v in the form ai + bj, given its magnitude v and the angle it makes with the positive x-axis.
-
(Multiple Choice)
4.8/5  (37)
(37)
Find all the complex roots. Leave your answers in polar form with the argument in degrees.
-The complex fifth roots of
(Multiple Choice)
4.9/5  (32)
(32)
Write the vector v in the form ai + bj, given its magnitude v and the angle it makes with the positive x-axis.
-
(Multiple Choice)
4.8/5  (45)
(45)
Showing 121 - 140 of 253
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)