Exam 13: A Preview of Calculus: the Limit, Derivative, and Integral of a Function
Exam 1: Functions and Their Graphs297 Questions
Exam 2: Linear and Quadratic Functions302 Questions
Exam 3: Polynomial and Rational Functions354 Questions
Exam 4: Exponential and Logarithmic Functions517 Questions
Exam 5: Trigonometric Functions354 Questions
Exam 6: Analytic Trigonometry342 Questions
Exam 7: Applications of Trigonometric Functions105 Questions
Exam 8: Polar Coordinates; Vectors253 Questions
Exam 9: Analytic Geometry200 Questions
Exam 10: Systems of Equations and Inequalities235 Questions
Exam 11: Sequences; Induction; the Binomial Theorem238 Questions
Exam 12: Counting and Probability115 Questions
Exam 13: A Preview of Calculus: the Limit, Derivative, and Integral of a Function145 Questions
Exam 14: Foundations: a Prelude to Functions234 Questions
Exam 15: Graphing Utilities29 Questions
Select questions type
Choose the one alternative that best completes the statement or answers the question.
Find the slope of the tangent line to the graph at the given point.
-
(Multiple Choice)
4.9/5  (26)
(26)
Use the graph shown to determine if the limit exists. If it does, find its value.
-
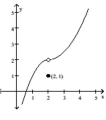
(Multiple Choice)
4.9/5  (31)
(31)
Find the limit as x approaches c of the average rate of change of the function from c to x.
-
(Multiple Choice)
4.9/5  (38)
(38)
Use the graph of y = g(x) to answer the question. 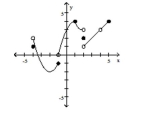 -What is the range of ?
-What is the range of ?
(Multiple Choice)
4.8/5  (35)
(35)
Choose the one alternative that best completes the statement or answers the question.
Approximate the area under the curve and above the x-axis using n rectangles. Let the height of each rectangle be given
by the value of the function at the right side of the rectangle.
-
(Multiple Choice)
5.0/5  (38)
(38)
Find the numbers at which f is continuous. At which numbers is f discontinuous?
-
(Multiple Choice)
4.8/5  (33)
(33)
Choose the one alternative that best completes the statement or answers the question.
Find the slope of the tangent line to the graph at the given point.
-
(Multiple Choice)
4.7/5  (33)
(33)
Find the numbers at which f is continuous. At which numbers is f discontinuous?
-
(Multiple Choice)
4.9/5  (37)
(37)
Showing 101 - 120 of 145
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)