Exam 5: Inverse, Exponential, and Logarithmic Functions
Exam 1: Review of Basic Concepts637 Questions
Exam 2: Equations and Inequalities498 Questions
Exam 3: Graphs and Functions531 Questions
Exam 4: Polynomials and Rational Functions517 Questions
Exam 5: Inverse, Exponential, and Logarithmic Functions472 Questions
Exam 6: Systems and Matrices505 Questions
Exam 7: Arithmetic Sequence: Common Difference and First n Terms570 Questions
Select questions type
The number of books in a small library increases according to the functio , where t is measured in years. How many books will the library have after 7 years?
(Multiple Choice)
4.7/5  (37)
(37)
models salinity of ocean water to depths of 1000 meters at a certain latitude. x is the depth in meters and f(x) is in grams of salt per kilogram of seawater. Approximate the depth (to the
Nearest tenth of a meter) where the salinity equals 35.
(Multiple Choice)
4.7/5  (42)
(42)
Use the formula , where the loudness of a sound in decibels is determined by , the number of watt/ produced by the soundwave, and . A certain noise produces watt of power. What is the decibel level of this noise? (Round to the nearest unit.)
(Multiple Choice)
4.8/5  (35)
(35)
Find the value. Give an approximation to four decimal places.
-
(Multiple Choice)
4.8/5  (38)
(38)
Find the domain and range of the inverse of the given function.
-
(Multiple Choice)
5.0/5  (37)
(37)
Round your answer to the nearest tenth, when appropriate. Use the formula , as
needed.
-Find the if .
(Multiple Choice)
4.9/5  (37)
(37)
Use a graphing calculator to estimate the solution set of the equation. Round to the nearest hundredth.
-
(Multiple Choice)
4.8/5  (36)
(36)
If the function is one-to-one, find its inverse. If not, write "not one-to-one."
-
(Multiple Choice)
4.8/5  (36)
(36)
Graph the exponential function using transformations where appropriate.
-
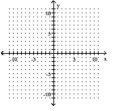
(Multiple Choice)
4.8/5  (31)
(31)
The graph of a function f is given. Use the graph to find the indicated value.
-
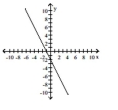
(Multiple Choice)
4.9/5  (29)
(29)
Solve the equation and express the solution in exact form.
-
(Multiple Choice)
4.7/5  (31)
(31)
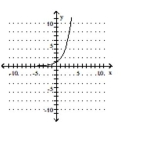
Use the graph of f to sketch a graph of the inverse of f using a dashed curve.
-
(Multiple Choice)
4.8/5  (38)
(38)
Find the function value. If the result is irrational, round your answer to the nearest thousandth.
-
(Multiple Choice)
5.0/5  (36)
(36)
Use the change of base rule to find the logarithm to four decimal places.
-log9 0.60
(Multiple Choice)
4.8/5  (35)
(35)
Showing 21 - 40 of 472
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)