Question 1

Determine whether f is continuous at c.
-$f ( x ) = \frac { x + 6 } { ( x - 2 ) ( x - 3 ) } ; c = - 6$

Accepted Answer

A)  not continuous 
B)  continuous 
A)  not continuous 
B)  continuous

Question 2

Determine whether f is continuous at c.
-$f ( x ) = \frac { 8 } { x ^ { 2 } + 3 x } ; \quad c = - 8$

Accepted Answer

A)  continuous 
B)  not continuous 
A)  continuous 
B)  not continuous

Question 3

Use the graph of y = g(x) to answer the question.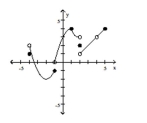  
-What is the domain of g? A) $\{ x \mid - 4 \leq x < 5 \}$

Accepted Answer

B)  $\{ x \mid - 4 \leq x < 4$ or $4 < x \leq 5 \}$ 
C)  $\{ x \mid - 4 < x < 5 \}$ 
D)  $\{ x \mid - 4 < x < - 1$ or $- 1 < x < 2$ or $2 < x \leq 5 \}$ 
B)  $\{ x \mid - 4 \leq x < 4$ or $4 < x \leq 5 \}$ 
C)  $\{ x \mid - 4 < x < 5 \}$ 
D)  $\{ x \mid - 4 < x < - 1$ or $- 1 < x < 2$ or $2 < x \leq 5 \}$

Question 4

Use the TABLE feature of a graphing utility to find the limit.
-$\lim _ { x \rightarrow 0 } \frac { x ^ { 2 } } { \cos x }$

Accepted Answer

A)  -1 
B)  0 
C)  1 
D)  does not exist 
A)  -1 
B)  0 
C)  1 
D)  does not exist

Question 5

Find the limit algebraically.
-$$\lim _ { x \rightarrow9 } \left( 6 - 3 x ^ { 2 } \right)$$

Accepted Answer

A)  -48 
B)  -237 
C)  249 
D)  -21 
A)  -48 
B)  -237 
C)  249 
D)  -21

Question 6

Find the limit algebraically.
-$$\lim _ { x \rightarrow 1 } \frac { 2 x - 7 } { 4 x + 5 }$$

Accepted Answer

A)  - 59 
B)  - 75 
C)  - 12 
D)  does not exist 
A)  - 59 
B)  - 75 
C)  - 12 
D)  does not exist

Question 7

Find the limit algebraically.
-$$\lim _ { x \rightarrow 2 } \frac { x ^ { 2 } - 4 } { x + 2 }$$

Accepted Answer

A)  Does not exist 
B)  -2 
C)  1 
D)  -4 
A)  Does not exist 
B)  -2 
C)  1 
D)  -4

Question 8

Find the limit as x approaches c of the average rate of change of the function from c to x.
-c = 9; $$f ( x ) = \frac { x } { 3 } + 10$$

Accepted Answer

A)  3 
B)  Does not exist 
C)  13 
D)  $$\frac { 1 } { 3 }$$ 
A)  3 
B)  Does not exist 
C)  13 
D)  $$\frac { 1 } { 3 }$$

Question 9

Find the limit as x approaches c of the average rate of change of the function from c to x.
-c = 4; $$f ( x ) = \frac { 3 } { x }$$ A) $- \frac { 3 } { 16 }$

Accepted Answer

B)  $- 12$ 
C)  $\frac { 3 } { 4 }$ 
D)  Does not exist 
B)  $- 12$ 
C)  $\frac { 3 } { 4 }$ 
D)  Does not exist

Question 10

Find the derivative of the function at the given value of x.
-$$f ( x ) = x ^ { 3 } + 4 x ; x = - 2$$

Accepted Answer

A)  -2 
B)  4 
C)  16 
D)  12 
A)  -2 
B)  4 
C)  16 
D)  12

Question 11

Find the one-sided limit.
-$\lim _ { x \rightarrow 2 ^ { + } } \left( x ^ { 2 } - 3 x - 3 \right)$

Accepted Answer

A)  13 
B)  -5 
C)  1 
D)  7 
A)  13 
B)  -5 
C)  1 
D)  7

Question 12

Find the limit algebraically.
-$$\lim _ { x \rightarrow 1 } \frac { x ^ { 3 } + 5 x ^ { 2 } + 3 x - 9 } { x - 1 }$$

Accepted Answer

A)  -16 
B)  0 
C)  16 
D)  does not exist 
A)  -16 
B)  0 
C)  16 
D)  does not exist

Question 13

Find the one-sided limit.
-$$\lim _ { x \rightarrow3  ^ { - } } \frac { x ^ { 2 } - 9 } { x - 3 }$$

Accepted Answer

A)  3 
B)  -6 
C)  6 
D)  -3 
A)  3 
B)  -6 
C)  6 
D)  -3

Question 14

Solve the problem.
-(a) What area does the integral $\int _ { 0 } ^ { \pi / 2 } \sin x \cos x \mathrm { dx }$ represent?
(b) Use a graphing utility to approximate the area to three decimal places.

Accepted Answer

(a) The ar

Question 15

Solve the problem.
-A foul tip of a baseball is hit straight upward from a height of 4 feet with an initial velocity of 112 feet per second. The function $$s ( t ) = - 16 t ^ { 2 } + 112 t + 4$$ 4 describes the ball's height above the ground, s(t), in feet, t seconds
After it was hit. The ball reaches its maximum height above the ground when the instantaneous speed reaches
Zero. After how many seconds does the ball reach its maximum height? A) 112

Accepted Answer

B)  $\frac { 3 } { 32 }$ 
C)  $\frac { 7 } { 2 }$ 
D)  7 
B)  $\frac { 3 } { 32 }$ 
C)  $\frac { 7 } { 2 }$ 
D)  7

Question 16

Approximate the area under the curve and above the x-axis using n rectangles. Let the height of each rectangle be given
by the value of the function at the right side of the rectangle.
-$$f ( x ) = 3 x ^ { 2 } - 2 \text { from } x = 1 \text { to } x = 5 ; n = 4$$

Accepted Answer

A)  140 
B)  154 
C)  144 
D)  150 
A)  140 
B)  154 
C)  144 
D)  150

Question 17

Find the numbers at which f is continuous. At which numbers is f discontinuous?
-$$f ( x ) = \frac { x + 5 } { x ^ { 2 } - 11 x + 24 }$$

Accepted Answer

A)  continuous for all real numbers except $x = - 8 , x = - 3$ 
B)  continuous for all real numbers except $x = 8 , x = 3$ 
C)  continuous for all real numbers except $x = - 5 , x = 8$, and $x = 3$ 
D)  continuous for all real numbers except $x = 5 , x = - 8$, and $x = - 3$ 
A)  continuous for all real numbers except $x = - 8 , x = - 3$ 
B)  continuous for all real numbers except $x = 8 , x = 3$ 
C)  continuous for all real numbers except $x = - 5 , x = 8$, and $x = 3$ 
D)  continuous for all real numbers except $x = 5 , x = - 8$, and $x = - 3$

Question 18

Find the limit algebraically.
-$$\lim _ { x \rightarrow 1 } \left( 3 x ^ { 2 } - 19 \right) ^ { 2 }$$

Accepted Answer

A)  100 
B)  -352 
C)  256 
D)  144 
A)  100 
B)  -352 
C)  256 
D)  144

Question 19

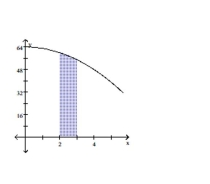
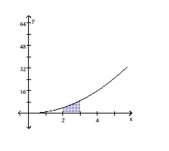
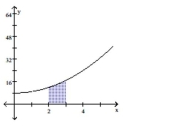
Solve the problem.
-Provide a graph that illustrates the area represented by the integral. $$\int _ { 2 } ^ { 3 } x ^ { 2 } d x$$
 
 A)

Accepted Answer

B) 
  
C) 
  
D) 
  
B) 
  
C) 
  
D)

Question 20

Determine whether f is continuous at c.
-$f ( x ) = \left\{ \begin{aligned}
- 2 x + 3 , & x  1
\end{aligned} \right.$

Accepted Answer

A)  not continuous 
B)  continuous 
A)  not continuous 
B)  continuous

Determine whether f is continuous at c. - $f ( x ) = \frac { x + 6 } { ( x - 2 ) ( x - 3 ) } ; c = - 6$

Determine whether f is continuous at c. - $f ( x ) = \frac { 8 } { x ^ { 2 } + 3 x } ; \quad c = - 8$

Use the TABLE feature of a graphing utility to find the limit. - $\lim _ { x \rightarrow 0 } \frac { x ^ { 2 } } { \cos x }$

Find the limit algebraically. - $\lim _ { x \rightarrow9 } \left( 6 - 3 x ^ { 2 } \right)$

Find the limit algebraically. - $\lim _ { x \rightarrow 1 } \frac { 2 x - 7 } { 4 x + 5 }$

Find the limit algebraically. - $\lim _ { x \rightarrow 2 } \frac { x ^ { 2 } - 4 } { x + 2 }$

Find the limit as x approaches c of the average rate of change of the function from c to x. -c = 9; $f ( x ) = \frac { x } { 3 } + 10$

Find the limit as x approaches c of the average rate of change of the function from c to x. -c = 4; $f ( x ) = \frac { 3 } { x }$ A) $- \frac { 3 } { 16 }$ B) $- 12$ C) $\frac { 3 } { 4 }$ D) Does not exist

Find the derivative of the function at the given value of x. - $f ( x ) = x ^ { 3 } + 4 x ; x = - 2$

Find the one-sided limit. - $\lim _ { x \rightarrow 2 ^ { + } } \left( x ^ { 2 } - 3 x - 3 \right)$

Find the limit algebraically. - $\lim _ { x \rightarrow 1 } \frac { x ^ { 3 } + 5 x ^ { 2 } + 3 x - 9 } { x - 1 }$

Find the one-sided limit. - $\lim _ { x \rightarrow3 ^ { - } } \frac { x ^ { 2 } - 9 } { x - 3 }$

Solve the problem. -(a) What area does the integral $\int _ { 0 } ^ { \pi / 2 } \sin x \cos x \mathrm { dx }$ represent? (b) Use a graphing utility to approximate the area to three decimal places.

Approximate the area under the curve and above the x-axis using n rectangles. Let the height of each rectangle be given by the value of the function at the right side of the rectangle. - $f ( x ) = 3 x ^ { 2 } - 2 \text { from } x = 1 \text { to } x = 5 ; n = 4$

Find the numbers at which f is continuous. At which numbers is f discontinuous? - $f ( x ) = \frac { x + 5 } { x ^ { 2 } - 11 x + 24 }$

Find the limit algebraically. - $\lim _ { x \rightarrow 1 } \left( 3 x ^ { 2 } - 19 \right) ^ { 2 }$

Determine whether f is continuous at c. - $f ( x ) = \left\{ \begin{aligned}- 2 x + 3 , & x < 1 \\1 , & x = 1 ; \quad c = 1 \\4 x - 1 , & x > 1\end{aligned} \right.$

Functions and Their Graphs

Linear and Quadratic Functions

Polynomial and Rational Functions

Exponential and Logarithmic Functions

Trigonometric Functions

Analytic Trigonometry

Applications of Trigonometric Functions

Polar Coordinates; Vectors

Analytic Geometry

Systems of Equations and Inequalities

Sequences; Induction; the Binomial Theorem

Counting and Probability

Review

Filters

Exam 13: A Preview of Calculus: the Limit, Derivative, and Integral of a Function

Determine whether f is continuous at c. - f(x)=x+6(x−2)(x−3);c=−6f ( x ) = \frac { x + 6 } { ( x - 2 ) ( x - 3 ) } ; c = - 6f(x)=(x−2)(x−3)x+6​;c=−6

Determine whether f is continuous at c. - f(x)=8x2+3x;c=−8f ( x ) = \frac { 8 } { x ^ { 2 } + 3 x } ; \quad c = - 8f(x)=x2+3x8​;c=−8

Use the TABLE feature of a graphing utility to find the limit. - lim⁡x→0x2cos⁡x\lim _ { x \rightarrow 0 } \frac { x ^ { 2 } } { \cos x }limx→0​cosxx2​

Find the limit algebraically. - lim⁡x→9(6−3x2)\lim _ { x \rightarrow9 } \left( 6 - 3 x ^ { 2 } \right)limx→9​(6−3x2)

Find the limit algebraically. - lim⁡x→12x−74x+5\lim _ { x \rightarrow 1 } \frac { 2 x - 7 } { 4 x + 5 }limx→1​4x+52x−7​

Find the limit algebraically. - lim⁡x→2x2−4x+2\lim _ { x \rightarrow 2 } \frac { x ^ { 2 } - 4 } { x + 2 }limx→2​x+2x2−4​

Find the limit as x approaches c of the average rate of change of the function from c to x. -c = 9; f(x)=x3+10f ( x ) = \frac { x } { 3 } + 10f(x)=3x​+10

Find the limit as x approaches c of the average rate of change of the function from c to x. -c = 4; f(x)=3xf ( x ) = \frac { 3 } { x }f(x)=x3​ A) −316- \frac { 3 } { 16 }−163​ B) −12- 12−12 C) 34\frac { 3 } { 4 }43​ D) Does not exist

Find the derivative of the function at the given value of x. - f(x)=x3+4x;x=−2f ( x ) = x ^ { 3 } + 4 x ; x = - 2f(x)=x3+4x;x=−2

Find the one-sided limit. - lim⁡x→2+(x2−3x−3)\lim _ { x \rightarrow 2 ^ { + } } \left( x ^ { 2 } - 3 x - 3 \right)limx→2+​(x2−3x−3)

Find the limit algebraically. - lim⁡x→1x3+5x2+3x−9x−1\lim _ { x \rightarrow 1 } \frac { x ^ { 3 } + 5 x ^ { 2 } + 3 x - 9 } { x - 1 }limx→1​x−1x3+5x2+3x−9​

Find the one-sided limit. - lim⁡x→3−x2−9x−3\lim _ { x \rightarrow3 ^ { - } } \frac { x ^ { 2 } - 9 } { x - 3 }limx→3−​x−3x2−9​

Solve the problem. -(a) What area does the integral ∫0π/2sin⁡xcos⁡xdx\int _ { 0 } ^ { \pi / 2 } \sin x \cos x \mathrm { dx }∫0π/2​sinxcosxdx represent? (b) Use a graphing utility to approximate the area to three decimal places.

Find the numbers at which f is continuous. At which numbers is f discontinuous? - f(x)=x+5x2−11x+24f ( x ) = \frac { x + 5 } { x ^ { 2 } - 11 x + 24 }f(x)=x2−11x+24x+5​

Find the limit algebraically. - lim⁡x→1(3x2−19)2\lim _ { x \rightarrow 1 } \left( 3 x ^ { 2 } - 19 \right) ^ { 2 }limx→1​(3x2−19)2

Solve the problem. -Provide a graph that illustrates the area represented by the integral. ∫23x2dx\int _ { 2 } ^ { 3 } x ^ { 2 } d x∫23​x2dx A) B) C) D)

Determine whether f is continuous at c. - f(x)={−2x+3,x<11,x=1;c=14x−1,x>1f ( x ) = \left\{ \begin{aligned}- 2 x + 3 , & x < 1 \\1 , & x = 1 ; \quad c = 1 \\4 x - 1 , & x > 1\end{aligned} \right.f(x)=⎩⎨⎧​−2x+3,1,4x−1,​x<1x=1;c=1x>1​