Explore all topics
Start Free Trial
Need Help? Contact us
back arrowfull exam logo
search
ai logoChat with AI
Servicesarrow
Discoverarrow

Topics

Explore all topics
Discover more topics

Exam 12: Geometry Problems: Complementary Angles, Collinear Points, and Similar Triangles

arrow
Exam 1: Line and Angle Relationships13 Questionsadd course
Exam 2: Parallel Lines13 Questionsadd course
Exam 3: Triangles16 Questionsadd course
Exam 4: Quadrilaterals14 Questionsadd course
Exam 5: Similar Triangles12 Questionsadd course
Exam 6: Circles10 Questionsadd course
Exam 7: Locus and Concurrence4 Questionsadd course
Exam 8: Areas of Polygons and Circles5 Questionsadd course
Exam 9: Surfaces and Solids4 Questionsadd course
Exam 10: Analytical Geometry8 Questionsadd course
Exam 11: Introduction to Trigonometry4 Questionsadd course
Exam 12: Geometry Problems: Complementary Angles, Collinear Points, and Similar Triangles916 Questionsadd course
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags
search iconSearch Question
flashcardsStudy Flashcards
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags

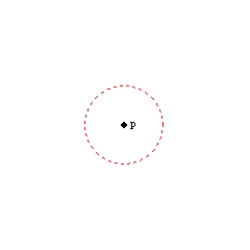-The locus of points in space that are at a distance of 1 inch from point P is the circle with center P and a radius length of 1 inch. -The locus of points in space that are at a distance of 1 inch from point P is the circle with center P and a radius length of 1 inch.

(True/False)
4.7/5
(39)
Question 441

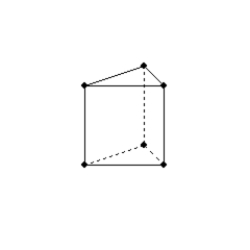-What is the total number of faces for a prism whose base is a decagon? -What is the total number of faces for a prism whose base is a decagon?

(Short Answer)
4.8/5
(24)
Question 442

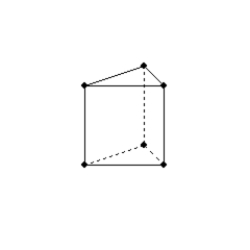-Where B is the area of the base and h is the length of altitude, the volume of a prism and cylinder can both be found by the formula V = Bh. -Where B is the area of the base and h is the length of altitude, the volume of a prism and cylinder can both be found by the formula V = Bh.

(True/False)
4.8/5
(28)
Question 443

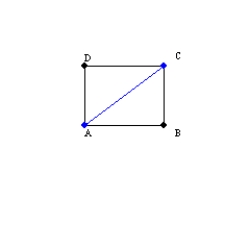-For a quadrilateral to be a rectangle, how must the diagonals be related? -For a quadrilateral to be a rectangle, how must the diagonals be related?

(Multiple Choice)
4.8/5
(25)
Question 444

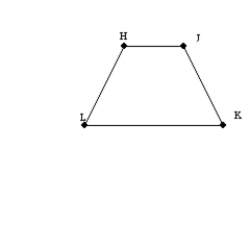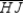-If M and N are the midpoints of nonparallel sides and of trapezoid HJKL, then . -If M and N are the midpoints of nonparallel sides -If M and N are the midpoints of nonparallel sides and of trapezoid HJKL, then . and -If M and N are the midpoints of nonparallel sides and of trapezoid HJKL, then . of trapezoid HJKL, then -If M and N are the midpoints of nonparallel sides and 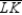of trapezoid HJKL, then . .

(True/False)
4.9/5
(34)
Question 445

To construct the midpoint of the horizontal line segment To construct the midpoint of the horizontal line segment , begin by marking off arcs of equal length from points A and B so that the arcs intersect both above and below . , begin by marking off arcs of equal length from points A and B so that the arcs intersect both above and below To construct the midpoint of the horizontal line segment 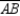, begin by marking off arcs of equal length from points A and B so that the arcs intersect both above and below . .

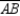(True/False)
4.7/5
(33)
Question 446

When two secants intersect at a point in the exterior of a circle, the measure of the angle formed is one-half the positive difference of the measures of the two intercepted arcs.

(True/False)
4.7/5
(32)
Question 447

Given that 1 foot = 12 inches, what is the number of square inches in 1 square foot?

(Essay)
4.8/5
(33)
Question 448

-In the figure, is said to be circumscribed about the circle. -In the figure, -In the figure, is said to be circumscribed about the circle. is said to be circumscribed about the circle.

(True/False)
4.8/5
(32)
Question 449

For a regular pentagon, find the measure of each central angle.

(Short Answer)
4.9/5
(37)
Question 450

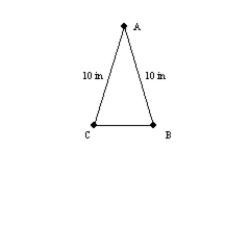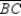-In the isosceles triangle shown, the legs are 10 inches each while m = 40°. Find the length of base correct to the nearest tenth of an inch. -In the isosceles triangle shown, the legs are 10 inches each while m -In the isosceles triangle shown, the legs are 10 inches each while m = 40°. Find the length of base correct to the nearest tenth of an inch. = 40°. Find the length of base -In the isosceles triangle shown, the legs are 10 inches each while m = 40°. Find the length of base correct to the nearest tenth of an inch. correct to the nearest tenth of an inch.

(Short Answer)
4.8/5
(29)
Question 451

-Suppose that . Which must be true? -Suppose that -Suppose that . Which must be true? . Which must be true?

(Multiple Choice)
4.9/5
(33)
Question 452

For what type of regular polygon does the apothem have a length equal to one-half the length of a side of the polygon?

(Short Answer)
4.8/5
(29)
Question 453

In the form In the form 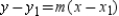, find the equation of the line that is the perpendicular- bisector of the line segment that joins (0,0) and (2a,2b). , find the equation of the line that is the perpendicular- bisector of the line segment that joins (0,0) and (2a,2b).

(Multiple Choice)
4.8/5
(43)
Question 454

-The locus of points in a plane that are equidistant from the sides of is the bisector of . -The locus of points in a plane that are equidistant from the sides of -The locus of points in a plane that are equidistant from the sides of is the bisector of . is the bisector of -The locus of points in a plane that are equidistant from the sides of 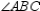is the bisector of . .

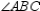(True/False)
4.7/5
(31)
Question 455

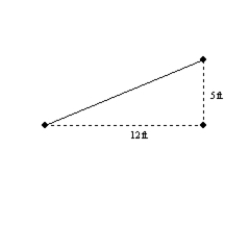-In a right triangle, the measures of the angles are , , and = 90°. With the lengths of the opposite sides being a, b, and c respectively, find tan . -In a right triangle, the measures of the angles are -In a right triangle, the measures of the angles are , , and = 90°. With the lengths of the opposite sides being a, b, and c respectively, find tan . , -In a right triangle, the measures of the angles are , , and = 90°. With the lengths of the opposite sides being a, b, and c respectively, find tan . , and -In a right triangle, the measures of the angles are , , and = 90°. With the lengths of the opposite sides being a, b, and c respectively, find tan . = 90°. With the lengths of the opposite sides being a, b, and c respectively, find tan -In a right triangle, the measures of the angles are , , and 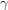= 90°. With the lengths of the opposite sides being a, b, and c respectively, find tan . .

(Essay)
4.8/5
(34)
Question 456

-In , m = 138°. Find m . -In -In , m = 138°. Find m . , m -In , m = 138°. Find m . = 138°. Find m -In , m = 138°. Find m . .

(Multiple Choice)
4.8/5
(35)
Question 457

Find the length of base in an isosceles triangle with perimeter 25 inches and length of leg 8 inches.

(Short Answer)
4.8/5
(31)
Question 458

-When the midpoints of the sides of a square RSTV are joined in order, quadrilateral MNPQ is formed. Being as specific as possible, what type of quadrilateral is MNPQ? -When the midpoints of the sides of a square RSTV are joined in order, quadrilateral MNPQ is formed. Being as specific as possible, what type of quadrilateral is MNPQ?

(Short Answer)
4.9/5
(37)
Question 459

-In the circle shown, m = (m m ). -In the circle shown, m -In the circle shown, m = (m m ). = -In the circle shown, m = (m m ). (m -In the circle shown, m = (m m ). -In the circle shown, m = (m m ). m -In the circle shown, m = (m m ). ).

(True/False)
4.9/5
(43)
Question 460
Showing 441 - 460 of 916
close modal

Filters

  • Essay(0)
  • Multiple Choice(0)
  • Short Answer(0)
  • True False(0)
  • Matching(0)