Explore all topics
Start Free Trial
Need Help? Contact us
back arrowfull exam logo
search
ai logoChat with AI
Servicesarrow
Discoverarrow

Topics

Explore all topics
Discover more topics

Exam 12: Geometry Problems: Complementary Angles, Collinear Points, and Similar Triangles

arrow
Exam 1: Line and Angle Relationships13 Questionsadd course
Exam 2: Parallel Lines13 Questionsadd course
Exam 3: Triangles16 Questionsadd course
Exam 4: Quadrilaterals14 Questionsadd course
Exam 5: Similar Triangles12 Questionsadd course
Exam 6: Circles10 Questionsadd course
Exam 7: Locus and Concurrence4 Questionsadd course
Exam 8: Areas of Polygons and Circles5 Questionsadd course
Exam 9: Surfaces and Solids4 Questionsadd course
Exam 10: Analytical Geometry8 Questionsadd course
Exam 11: Introduction to Trigonometry4 Questionsadd course
Exam 12: Geometry Problems: Complementary Angles, Collinear Points, and Similar Triangles916 Questionsadd course
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags
search iconSearch Question
flashcardsStudy Flashcards
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags

Where Where 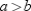, the distance between the points (a, c) and (b, c) is ( ) units. , the distance between the points (a, c) and (b, c) is ( Where , the distance between the points (a, c) and (b, c) is ( ) units. ) units.

(True/False)
4.8/5
(31)
Question 101

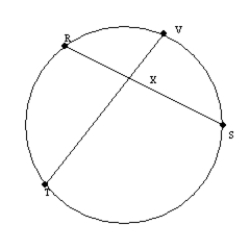-For the circle shown, chords and intersect at point X. With RX = 6, XS = 8, TX = x + 8 and XV = x, find the length of . -For the circle shown, chords -For the circle shown, chords and intersect at point X. With RX = 6, XS = 8, TX = x + 8 and XV = x, find the length of . and -For the circle shown, chords and intersect at point X. With RX = 6, XS = 8, TX = x + 8 and XV = x, find the length of . intersect at point X. With RX = 6, XS = 8, TX = x + 8 and XV = x, find the length of -For the circle shown, chords and 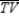intersect at point X. With RX = 6, XS = 8, TX = x + 8 and XV = x, find the length of . .

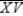(Short Answer)
4.9/5
(33)
Question 102

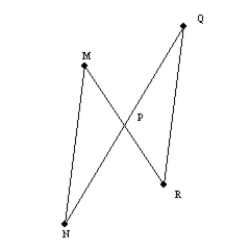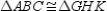- . In , is the median from vertex A to side . Likewise, is the median of from vertex G to side . How are and related? - - . In , is the median from vertex A to side . Likewise, is the median of from vertex G to side . How are and related? . In - . In 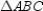, 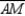is the median from vertex A to side 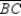. Likewise, is the median of from vertex G to side . How are and related? , - . In , is the median from vertex A to side . Likewise, is the median of from vertex G to side . How are and related? is the median from vertex A to side - . In , is the median from vertex A to side . Likewise, is the median of from vertex G to side . How are and related? . Likewise, - . In , is the median from vertex A to side . Likewise, is the median of from vertex G to side . How are and related? is the median of - . 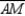In , is the median from vertex A to side . Likewise, is the median of from vertex G to side . How are and related? from vertex G to side - . In , is the median from vertex A to side . Likewise, is the median of from vertex G to side . How are and related? . How are - . In , is the median from vertex A to side . Likewise, is the median of from vertex G to side . How are and related? and - . In , is the median from vertex A to side . Likewise, is the median of from vertex G to side . How are and related? related?

(Essay)
4.8/5
(41)
Question 103

While the coordinate axes separate the Cartesian plane into 4 subsets (quadrants), the xy plane, xz plane, and yz plane separte Cartesian space into:

(Multiple Choice)
4.7/5
(40)
Question 104

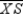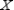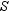In In , the line segment joins vertex to point on the opposite side in such a way that . In relation to , what name is given to ? , the line segment In , the line segment joins vertex to point on the opposite side in such a way that . In relation to , what name is given to ? joins vertex In , the line segment joins vertex to point on the opposite side in such a way that . In relation to , what name is given to ? 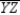to point In , the line segment joins vertex to point on the opposite side in such a way that . In relation to , what name is given to ? on the opposite side In , the line segment joins vertex to point on the opposite side in such a way that . In relation to , what name is given to ? in such a way that In , the line segment joins vertex to point on the opposite side in such a way that . In relation to , what name is given to ? . In relation to In , the line segment joins vertex to point on the opposite side in such a way that . In relation to , what name is given to ? , what name is given to In , the line segment joins vertex to point on the opposite side in such a way that 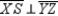. In relation to 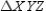, what name is given to ? ?

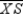(Short Answer)
4.8/5
(40)
Question 105

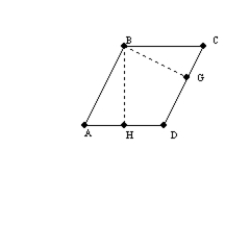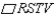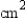-The area of is 35.1 . Choosing a side of RSTV that measures 4.5 cm as the base, what is the length of the corresponding altitude? -The area of -The area of is 35.1 . Choosing a side of RSTV that measures 4.5 cm as the base, what is the length of the corresponding altitude? is 35.1 -The area of is 35.1 . Choosing a side of RSTV that measures 4.5 cm as the base, what is the length of the corresponding altitude? . Choosing a side of RSTV that measures 4.5 cm as the base, what is the length of the corresponding altitude?

(Multiple Choice)
4.8/5
(36)
Question 106

If the length of each apothem of a square is a, then the length of each side is 2a.

(True/False)
4.8/5
(35)
Question 107

Given that 1 foot = 12 inches, how many cubic inches are in one cubic foot?

(Essay)
4.8/5
(40)
Question 108

-A right circular cone has a radius of 6 inches and an altitude of 8 inches. Use in order to find the exact total area of the cone. -A right circular cone has a radius of 6 inches and an altitude of 8 inches. Use -A right circular cone has a radius of 6 inches and an altitude of 8 inches. Use in order to find the exact total area of the cone. in order to find the exact total area of the cone.

(Essay)
4.9/5
(28)
Question 109

A pentagon has the same number of diagonals as it has sides.

(True/False)
4.8/5
(33)
Question 110

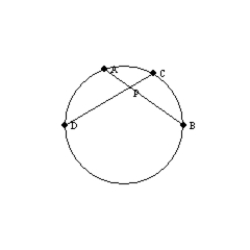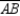-In the circle, chords and intersect at point P. If m = 63° and m = 75°, find m . -In the circle, chords -In the circle, chords and intersect at point P. If m = 63° and m = 75°, find m . and -In the circle, chords and intersect at point P. If m = 63° and m = 75°, find m . intersect at point P. If m -In the circle, chords and intersect at point P. If m = 63° and m = 75°, find m . = 63° and m -In the circle, chords and intersect at point P. If m 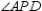= 63° and m = 75°, find m . = 75°, find m -In the circle, chords and intersect at point P. If m = 63° and m = 75°, find m . .

(Short Answer)
4.9/5
(34)
Question 111

Externally tangent circles P and Q touch at point T and have the line of centers Externally tangent circles P and Q touch at point T and have the line of centers 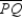. How does one construct the common internal tangent for circles P and Q? . How does one construct the common internal tangent for circles P and Q?

(Essay)
4.9/5
(36)
Question 112

If m If m = x and 90° < x < 180°, then is a(n): = x and 90° < x < 180°, then If m = x and 90° < x < 180°, then is a(n): is a(n):

(Multiple Choice)
4.8/5
(31)
Question 113

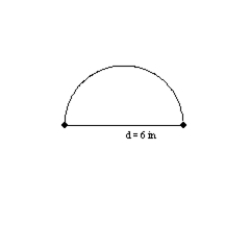-What is the exact volume for the solid that results when the semicircular region shown is rotated about its diameter of length 6 inches? -What is the exact volume for the solid that results when the semicircular region shown is rotated about its diameter of length 6 inches?

(Essay)
4.8/5
(34)
Question 114

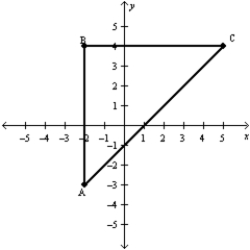-Find the area of , which has vertices at A(-2,-3), B(-2,4) and C(5,4). -Find the area of -Find the area of , which has vertices at A(-2,-3), B(-2,4) and C(5,4). , which has vertices at A(-2,-3), B(-2,4) and C(5,4).

(Multiple Choice)
4.9/5
(31)
Question 115

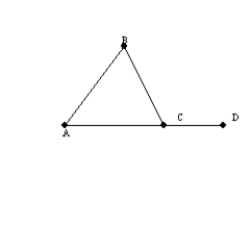-In (not shown), . Which statement is not necessarily true? -In -In 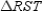(not shown), . Which statement is not necessarily true? (not shown), -In (not shown), . Which statement is not necessarily true? . Which statement is not necessarily true?

(Multiple Choice)
4.7/5
(36)
Question 116

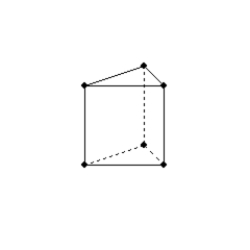-A prism and pyramid have congruent bases and the same length of altitude. If the volume of the pyramid is 24 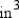, find the volume of the prism. -A prism and pyramid have congruent bases and the same length of altitude. If the volume of the pyramid is 24 -A prism and pyramid have congruent bases and the same length of altitude. If the volume of the pyramid is 24 , find the volume of the prism. , find the volume of the prism.

(Essay)
4.9/5
(31)
Question 117

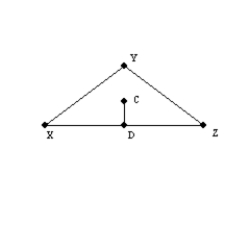-Which of the following lines (line segments) are concurrent? -Which of the following lines (line segments) are concurrent?

(Multiple Choice)
4.8/5
(31)
Question 118

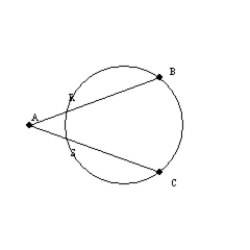-In the figure, secants and intersect the circle at points R and S respectively. If m = 36° and m : m = 4:1, find m . -In the figure, secants -In the figure, secants and intersect the circle at points R and S respectively. If m = 36° and m : m = 4:1, find m . and -In the figure, secants and intersect the circle at points R and S respectively. If m = 36° and m : m = 4:1, find m . intersect the circle at points R and S respectively. If m -In the figure, secants and intersect the circle at points R and S respectively. If m = 36° and m : m = 4:1, find m . = 36° and m 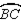-In the figure, secants and intersect the circle at points R and S respectively. If m = 36° and m : m = 4:1, find m . : m -In the figure, secants and intersect the circle at points R and S respectively. If m = 36° and m : m = 4:1, find m . = 4:1, find m -In the figure, secants and intersect the circle at points R and S respectively. If m = 36° and m : m 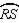= 4:1, find m . .

(Short Answer)
4.7/5
(35)
Question 119

In Cartesian space, the point (0,6,0) lies 6 units above the origin.

(True/False)
4.8/5
(31)
Question 120
Showing 101 - 120 of 916
close modal

Filters

  • Essay(0)
  • Multiple Choice(0)
  • Short Answer(0)
  • True False(0)
  • Matching(0)