Exam 6: Discrete Probability Distributions

A statistics student receives a grade of 90 on a statistics midterm. If the corresponding z-score Equals +1.5 and the standard deviation equals 7, determine the average grade on this exam.

A random variable from an experiment where outcomes are normally distributed can have

Replacement times for TV sets are normally distributed with a mean of 8.2 years and a standard Deviation of 1.1 years, (based on data from "Getting Things Fixed," Consumer Reports). (i. If you want to provide a warranty so that only 3% of the TV sets will be replaced before the Warranty expires, the length of warranty you would recommend would be 6.13 years. (ii. The probability that a randomly selected TV will be replaced after more than 10.0 years is 0)0505.

From past history, the scores on a statistics test are normally distributed with a mean of 70% and a Standard deviation of 5%. To earn a "B" on the test, a student must be in the top 15% of the class. What should a student score to receive a "B"?

A sample of 500 evening students revealed that their annual incomes from employment in industry During the day were normally distributed with a mean income of $30,000 and a standard deviation Of $3,000. (i. 250 students earned more than $30,000. (ii. 500 students earned between $20,000 and $40,000. (iii) 11 students earned more than $36,000.

Replacement times for TV sets are normally distributed with a mean of 8.2 years and a standard Deviation of 1.1 years, (based on data from "Getting Things Fixed," Consumer Reports). (i. The replacement time that separated the top 20% from the bottom 80% is 9.124 years. (ii. The probability that a randomly selected TV will be replaced in less than 4.0 years is 0.00.

(i. The formula to convert any normal distribution to the standard normal distribution is 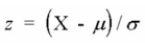(ii) The standardized z value measures distance from the mean in units of standard deviation. (iii) The area under a normal curve to the right of a z-score of zero is 1.

What is the proportion of the total area under the normal curve within plus and minus two standard Deviations of the mean?

For the normal distribution, the mean plus and minus 1.96 standard deviations will include about What percent of the observations?

(i. The formula to convert any normal distribution to the standard normal distribution is (ii) The standardized z value measures distance from the mean in units of standard deviation. (iii) The area under a normal curve to the right of a z-score of zero is a proportion of 0.50.

(i. About 95.5% percent of the area under the normal curve is within plus one and minus one Standard deviation of the mean. (ii. The normal distribution is a continuous probability distribution. (iii) In a standard normal distribution, µ = 0 and σ = 1.

Which of the following is true in a normal distribution?

One classic use of the normal distribution is inspired by a letter to Dear Abbey in which a wife Claimed to have given birth 308 days after a brief visit from her husband, who was serving in the Navy. The lengths of pregnancies are normally distributed with a mean of 268 days and a standard Deviation of 15 days. Given this information. (i. the probability of a pregnancy lasting 308 days or longer is 0.0038. (ii. The result suggests that the husband is not the father of the child.

A loaf of bread is normally distributed with a mean of 22 ounces and a standard deviation of ½ Ounces. (i. The probability that a loaf of bread is < 20 ounces is 0.0. (ii. The probability that a loaf of bread is > 21 ounces is 0.9772. (iii) The probability that a loaf of bread is < 24 ounces is 1.0.

Management is considering adopting a bonus system to increase production. One suggestion is to Pay a bonus on the highest 5 percent of production based on past experience. Past records Indicate that, on the average, 4,000 units of a small assembly are produced during a week. The Distribution of the weekly production is approximately normally distributed with a standard deviation Of 60 units. If the bonus is paid on the upper 5 percent of production, the bonus will be paid on how Many units or more?

One classic use of the normal distribution is inspired by a letter to Dear Abbey in which a wife Claimed to have given birth 308 days after a brief visit from her husband, who was serving in the Navy. The lengths of pregnancies are normally distributed with a mean of 268 days and a standard Deviation of 15 days. Given this information. (i. the probability of a pregnancy lasting 308 days or longer is 0.0038. (ii. The result suggests that the husband is the father of the child.

A sample of 500 evening students revealed that their annual incomes were normally distributed With a mean income of $50,000 and a standard deviation of $4,000. How many students earned Between $45,000 and $53,000?

A sample of 500 evening students revealed that their annual incomes were normally distributed With a mean income of $50,000 and a standard deviation of $4,000. How many students earned Between $47,000 and $53,000?

The average score of 100 students taking a statistics final was 70 with a standard deviation of 7. Assuming a normal distribution, approximately how many scored 90 or higher?

Ball-Bearings, Inc. produces ball bearings automatically on a Kronar BBX machine. For one of the Ball bearings, the mean diameter is set at 20.00 mm (millimetres). The standard deviation of the Production over a long period of time was computed to be 0.150 mm. What percent of the ball Bearings will have diameters 20.27 mm or more?