Exam 14: Multiple Integration
Exam 1: Preparation for Calculus125 Questions
Exam 2: Limits and Their Properties85 Questions
Exam 3: Differentiation193 Questions
Exam 4: Applications of Differentiation154 Questions
Exam 5: Integration184 Questions
Exam 6: Differential Equations93 Questions
Exam 7: Applications of Integration119 Questions
Exam 8: Integration Techniques and Improper Integrals130 Questions
Exam 9: Infinite Series181 Questions
Exam 10: Conics, Parametric Equations, and Polar Coordinates114 Questions
Exam 11: Vectors and the Geometry of Space130 Questions
Exam 12: Vector-Valued Functions85 Questions
Exam 13: Functions of Several Variables173 Questions
Exam 14: Multiple Integration143 Questions
Exam 15: Vector Anal142 Questions
Select questions type
Find the area of the portion of the surface  that lies above the region
that lies above the region 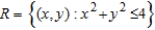 . Round your answer to two decimal places.
. Round your answer to two decimal places.
(Multiple Choice)
4.8/5  (30)
(30)
Consider the region 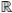 in the xy-plane bounded by the ellipse
in the xy-plane bounded by the ellipse  and the transformation
and the transformation  and
and 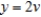 . Find
. Find 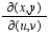 .
.
(Multiple Choice)
4.9/5  (32)
(32)
Evaluate the integral  by switching the order of integration. Round your answer to two decimal places.
by switching the order of integration. Round your answer to two decimal places.
(Multiple Choice)
4.9/5  (27)
(27)
Evaluate the iterated integral 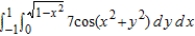 by converting to polar coordinates. Round your answer to four decimal places.
by converting to polar coordinates. Round your answer to four decimal places.
(Multiple Choice)
4.9/5  (38)
(38)
Evaluate the iterated integral  by converting to polar coordinates.
by converting to polar coordinates.
(Multiple Choice)
4.9/5  (31)
(31)
Use a double integral to find the area enclosed by the graph of 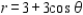 .
. 
(Multiple Choice)
4.7/5  (32)
(32)
Find the Jacobian for the indicated change of variables. 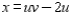 ,
, 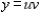
(Multiple Choice)
4.9/5  (40)
(40)
The area of a region R is given by the iterated integral 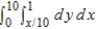 . Switch the order of integration and show that both orders yield the same area. What is this area?
. Switch the order of integration and show that both orders yield the same area. What is this area?
(Multiple Choice)
4.7/5  (28)
(28)
Identify the region of integration for the following integral. 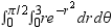
(Multiple Choice)
4.9/5  (33)
(33)
A company produces a spherical object of radius 17 centimeters. A hole of radius 4 centimeters is drilled through the center of the object. Find the outer surface area of the object.
(Multiple Choice)
4.8/5  (37)
(37)
Set up a triple integral for the volume of the solid bounded by the coordinate planes and the plane given below. 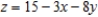
(Multiple Choice)
4.8/5  (33)
(33)
Find  of the center of mass of the solid of given density
of the center of mass of the solid of given density 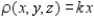 bounded by the graphs of the equations
bounded by the graphs of the equations 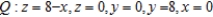 .
.
(Multiple Choice)
4.8/5  (36)
(36)
Determine the diameter of a hole that is drilled vertically through the center of the solid bounded by the graphs of the equations 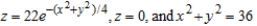 if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.
if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.
(Multiple Choice)
4.8/5  (35)
(35)
Showing 121 - 140 of 143
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)