Exam 14: Multiple Integration
Exam 1: Preparation for Calculus125 Questions
Exam 2: Limits and Their Properties85 Questions
Exam 3: Differentiation193 Questions
Exam 4: Applications of Differentiation154 Questions
Exam 5: Integration184 Questions
Exam 6: Differential Equations93 Questions
Exam 7: Applications of Integration119 Questions
Exam 8: Integration Techniques and Improper Integrals130 Questions
Exam 9: Infinite Series181 Questions
Exam 10: Conics, Parametric Equations, and Polar Coordinates114 Questions
Exam 11: Vectors and the Geometry of Space130 Questions
Exam 12: Vector-Valued Functions85 Questions
Exam 13: Functions of Several Variables173 Questions
Exam 14: Multiple Integration143 Questions
Exam 15: Vector Anal142 Questions
Select questions type
Use cylindrical coordinates to find the mass of the solid  where
where  .
.
(Multiple Choice)
4.8/5  (30)
(30)
Combine the sum of the two iterated integrals into a single integral by converting to polar coordinates. Evaluate the resulting iterated integral. 
(Multiple Choice)
4.8/5  (35)
(35)
Find the area of the portion of the surface 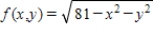 that lies above the region
that lies above the region 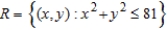 . Round your answer to two decimal places.
. Round your answer to two decimal places.
(Multiple Choice)
5.0/5  (35)
(35)
Find the area of the surface for the portion of the sphere 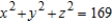 inside the cylinder
inside the cylinder 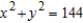 .
.
(Multiple Choice)
4.8/5  (33)
(33)
Find the area of the surface given by 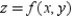 over the region R.
over the region R. 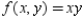
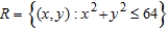
(Multiple Choice)
4.8/5  (31)
(31)
Set up and evaluate a double integral required to find the moment of inertia, I, about the given line, of the lamina bounded by the graphs of the following equations. Use a computer algebra system to evaluate the double integral. 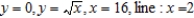

(Multiple Choice)
4.8/5  (32)
(32)
Suppose the population density of a city is approximated by the model  where x and y are measured in miles. Integrate the density function over the indicated circular region to approximate the population of the city. Round your answer to the nearest integer.
where x and y are measured in miles. Integrate the density function over the indicated circular region to approximate the population of the city. Round your answer to the nearest integer.
(Multiple Choice)
5.0/5  (29)
(29)
Use a double integral to find the area of the region inside the circle  and outside the cardioid
and outside the cardioid 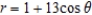 . Round your answer to two decimal places.
. Round your answer to two decimal places.
(Multiple Choice)
4.9/5  (42)
(42)
Convert the integral below from rectangular coordinates to both cylindrical and spherical coordinates, and evaluate the simpler iterated integral. 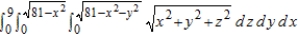
(Multiple Choice)
4.9/5  (35)
(35)
Find the area of the surface given by  over the region R.
over the region R. 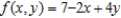
R: square with vertices
R: square with vertices 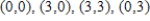
(Multiple Choice)
4.9/5  (38)
(38)
Find the area of the surface of the portion of the plane  in the first octant.
in the first octant.
(Multiple Choice)
4.8/5  (30)
(30)
Find the area of the surface given by 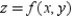 over the region R.
over the region R. 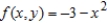
R: rectangle with vertices
R: rectangle with vertices 
(Multiple Choice)
4.8/5  (32)
(32)
Use a double integral in polar coordinates to find the volume of the solid in the first octant bounded by the graphs of the equations given below. 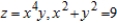
(Multiple Choice)
5.0/5  (38)
(38)
Find the average value of  over the region R, where R is a triangle with vertices
over the region R, where R is a triangle with vertices 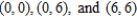 .
.
(Multiple Choice)
4.7/5  (31)
(31)
Find the Jacobian 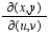 for the following change of variables:
for the following change of variables: 
(Multiple Choice)
4.9/5  (41)
(41)
Given 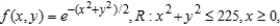 use polar coordinates to set up and evaluate the double integral
use polar coordinates to set up and evaluate the double integral 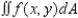 .
.
(Multiple Choice)
4.8/5  (38)
(38)
Find the center of mass of the solid bounded by  and
and 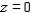 with density function
with density function 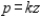 .
.
(Multiple Choice)
5.0/5  (42)
(42)
Sketch the image S in the uv-plane of the region R in the xy-plane using the given transformation. 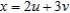
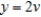
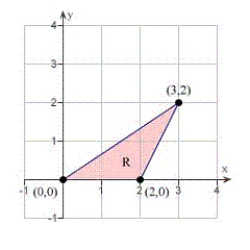
(Multiple Choice)
4.9/5  (29)
(29)
Showing 81 - 100 of 143
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)