Exam 8: Confidence Interval Estimation

TABLE 8-12 A poll was conducted by the marketing department of a video game company to determine the popularity of a new game that was targeted to be launched in three months. Telephone interviews with 1,500 young adults were conducted which revealed that 49% said they would purchase the new game. The margin of error was ±3 percentage points. -Referring to Table 8-12, the size of the population is 1,500.

TABLE 8-10 A university wanted to find out the percentage of students who felt comfortable reporting cheating by their fellow students. A survey of 2,800 students was conducted and the students were asked if they felt comfortable reporting cheating by their fellow students. The results were 1,344 answered "yes" and 1,456 answered "no". -Referring to Table 8-10, the critical value for a 99% confidence interval for this sample is ________.

TABLE 8-6 After an extensive advertising campaign, the manager of a company wants to estimate the proportion of potential customers that recognize a new product. She samples 120 potential consumers and finds that 54 recognize this product. She uses this sample information to obtain a 95% confidence interval that goes from 0.36 to 0.54. -Referring to Table 8-6, it is possible that the true proportion of people that recognize the product is not between 0.36 and 0.54.

TABLE 8-3 To become an actuary, it is necessary to pass a series of 10 exams, including the most important one, an exam in probability and statistics. An insurance company wants to estimate the mean score on this exam for actuarial students who have enrolled in a special study program. They take a sample of 8 actuarial students in this program and determine that their scores are: 2, 5, 8, 8, 7, 6, 5, and 7. This sample will be used to calculate a 90% confidence interval for the mean score for actuarial students in the special study program. -Referring to Table 8-3, if we use the same sample information to obtain a 95% confidence interval, the resulting interval would be narrower than the one obtained here with 90% confidence.

TABLE 8-5 A sample of salary offers (in thousands of dollars) given to management majors is: 48, 51, 46, 52, 47, 48, 47, 50, 51, and 59. Using this data to obtain a 95% confidence interval resulted in an interval from 47.19 to 52.61. -Referring to Table 8-5, it is possible that the mean of the population is between 47.19 and 52.61.

TABLE 8-8 The president of a university would like to estimate the proportion of the student population that owns a personal computer. In a sample of 500 students, 417 own a personal computer. -Referring to Table 8-8, a 99% confidence interval for the proportion of the student population who own a personal computer is from ________ to ________.

TABLE 8-8 The president of a university would like to estimate the proportion of the student population that owns a personal computer. In a sample of 500 students, 417 own a personal computer. -Referring to Table 8-8, the parameter of interest is the proportion of the student population who own a personal computer.

Private colleges and universities rely on money contributed by individuals and corporations for their operating expenses. Much of this money is put into a fund called an endowment, and the college spends only the interest earned by the fund. A recent survey of 8 private colleges in the United States revealed the following endowments (in millions of dollars): 60.2, 47.0, 235.1, 490.0, 122.6, 177.5, 95.4, and 220.0. What value will be used as the point estimate for the mean endowment of all private colleges in the United States?

TABLE 8-10 A university wanted to find out the percentage of students who felt comfortable reporting cheating by their fellow students. A survey of 2,800 students was conducted and the students were asked if they felt comfortable reporting cheating by their fellow students. The results were 1,344 answered "yes" and 1,456 answered "no". -Referring to Table 8-10, the parameter of interest is the total number of students in the population who feel comfortable reporting cheating by their fellow students.

TABLE 8-7 A hotel chain wants to estimate the mean number of rooms rented daily in a given month. The population of rooms rented daily is assumed to be normally distributed for each month with a standard deviation of 24 rooms. During February, a sample of 25 days has a sample mean of 37 rooms. -Referring to Table 8-7, a 99% confidence interval for the mean number of rooms rented daily in a given month is from ________ to ________.

For a given data set, the confidence interval will be wider for 95% confidence than for 90% confidence.

TABLE 8-10 A university wanted to find out the percentage of students who felt comfortable reporting cheating by their fellow students. A survey of 2,800 students was conducted and the students were asked if they felt comfortable reporting cheating by their fellow students. The results were 1,344 answered "yes" and 1,456 answered "no". -Referring to Table 8-10, a 99% confidence interval will contain 99% of the student population who feel comfortable reporting cheating by their fellow students.

The t distribution allows the calculation of confidence intervals for means for small samples when the population variance is not known, regardless of the shape of the distribution in the population.

TABLE 8-12 A poll was conducted by the marketing department of a video game company to determine the popularity of a new game that was targeted to be launched in three months. Telephone interviews with 1,500 young adults were conducted which revealed that 49% said they would purchase the new game. The margin of error was ±3 percentage points. -Referring to Table 8-12, what is the needed sample size to obtain a 95% confidence interval in estimating the percentage of the targeted young adults who will purchase the new game to within ±5%?

The head librarian at the Library of Congress has asked her assistant for an interval estimate of the mean number of books checked out each day. The assistant provides the following interval estimate: from 740 to 920 books per day. What is an efficient, unbiased point estimate of the number of books checked out each day at the Library of Congress?

TABLE 8-11 A sales and marketing management magazine conducted a survey on salespeople cheating on their expense reports and other unethical conduct. In the survey on 200 managers, 58% of the managers have caught salespeople cheating on an expense report, 50% have caught salespeople working a second job on company time, 22% have caught salespeople listing a "strip bar" as a restaurant on an expense report, and 19% have caught salespeople giving a kickback to a customer. -Referring to Table 8-11, construct a 95% confidence interval estimate of the population proportion of managers who have caught salespeople giving a kickback to a customer.

The county clerk wants to estimate the proportion of voters who will need special election facilities. Suppose a sample of 400 voters was taken. If 150 need special election facilities, calculate an 90% confidence interval for the population proportion.

A major department store chain is interested in estimating the mean amount its credit card customers spent on their first visit to the chain's new store in the mall. Fifteen credit card accounts were randomly sampled and analyzed with the following results: 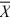= $50.50 and S = 20. Assuming the distribution of the amount spent on their first visit is approximately normal, what is the shape of the sampling distribution of the sample mean that will be used to create the desired confidence interval for μ?

It is desired to estimate the mean total compensation of CEOs in the Service industry. Data were randomly collected from 18 CEOs and the 95% confidence interval was calculated to be ($2,181,260, $5,836,180). Based on the interval above, do you believe the mean total compensation of CEOs in the Service industry is more than $3,000,000?

The t distribution allows the calculation of confidence intervals for means when the actual standard deviation is not known.