Question 1

Explain what is meant by a p-value.

Accepted Answer

A p-value is the probability of getting

Question 2

When testing a hypothesis involving population proportions,an increase in sample size will result in a smaller chance of making a Type I statistical error.

Accepted Answer

A) True 
 B)False

Question 3

The executive director of the United Way believes that more than 24 percent of the employees in the high-tech industry have made voluntary contributions to the United Way.In order to test this statistically,the appropriate null and alternative hypotheses are:
H0 :   ≤ .24
HA :   > .24

Accepted Answer

A) True 
 B)False

Question 4

In conducting a hypothesis test where the conclusion is to reject the null hypothesis,then either a correct decision has been made or else a Type I error.

Accepted Answer

A) True 
 B)False

Question 5

A large tire manufacturing company has claimed that its top line tire will average more than 80,000 miles.If a consumer group wished to test this claim,the research hypothesis would be: Ha : μ > 80,000 miles.

Accepted Answer

A) True 
 B)False

Question 6

The director of the city Park and Recreation Department claims that the mean distance people travel to the city's greenbelt is more than 5.0 miles.Assume that the population standard deviation is known to be 1.2 miles and the significance level to be used to test the hypothesis is 0.05 when a sample size of n = 64 people are surveyed.Given this information,if the sample mean is 15.90 miles,the null hypothesis should be rejected.

Accepted Answer

A) True 
 B)False

Question 7

The probability of a Type II error decreases as the "true" population value gets farther from the hypothesized population value,given that everything else is held constant.

Accepted Answer

A) True 
 B)False

Question 8

A major package delivery company claims that at least 95 percent of the packages it delivers reach the destination on time.As part of the evidence in a lawsuit against the package company,a random sample of n = 200 packages was selected.A total of 188 of these packages were delivered on time.Using a significance level of 0.05,the critical value for this hypothesis test is approximately 0.90.

Accepted Answer

A) True 
 B)False

Question 9

The Center on Budget and Policy Priorities (www.cbpp.org)reported that average out-of-pocket medical expenses for prescription drugs for privately insured adults with incomes over 200% of the poverty level was $173 in 2002.Suppose an investigation was conducted in 2012 to determine whether the increased availability of generic drugs,Internet prescription drug purchases,and cost controls have reduced out-of-pocket drug expenses.The investigation randomly sampled 196 privately insured adults with incomes over 200% of the poverty level,and the respondents' 2012 out-of-pocket medical expenses for prescription drugs were recorded.These data are in the file Drug Expenses.Based on the sample data,can it be concluded that 2012 out-of-pocket prescription drug expenses are lower than the 2002 average reported by the Center on Budget and Policy Priorities? Use a level of significance of 0.01 to conduct the hypothesis test.

Accepted Answer

A) Because t = -2.69 is less than -2.3456,do not reject H0
Conclude that 2012 average out-of-pocket prescription drug expenses are not lower than the 2002 average. 
B) Because t = -2.69 is less than -2.3456,reject H0
Conclude that 2012 average out-of-pocket prescription drug expenses are lower than the 2002 average. 
C) Because t = -1.69 is less than -0.8712,reject H0
Conclude that 2012 average out-of-pocket prescription drug expenses are lower than the 2002 average. 
D) Because t = -1.69 is less than -0.8712,do not reject H0 
A) Because t = -2.69 is less than -2.3456,do not reject H0
Conclude that 2012 average out-of-pocket prescription drug expenses are not lower than the 2002 average. 
B) Because t = -2.69 is less than -2.3456,reject H0
Conclude that 2012 average out-of-pocket prescription drug expenses are lower than the 2002 average. 
C) Because t = -1.69 is less than -0.8712,reject H0
Conclude that 2012 average out-of-pocket prescription drug expenses are lower than the 2002 average. 
D) Because t = -1.69 is less than -0.8712,do not reject H0

Question 10

The Gordon Beverage Company bottles soft drinks using an automatic filling machine.When the process is running properly,the mean fill is 12 ounces per can.The machine has a known standard deviation of 0.20 ounces.Each day,the company selects a random sample of 36 cans and measures the volume in each can.They then test to determine whether the filling process is working properly.The test is conducted using a 0.05 significance level.Using the test statistic approach,what conclusion should the company reach if the sample mean is 12.02 ounces? What type of statistical error may have been committed?

Accepted Answer

The company will take action if it deter

Question 11

A small city is considering breaking away from the county school system and starting its own city school system.City leaders believe that more than 60 percent of residents support the idea.A poll of n = 215 residents is taken and 134 people say they support starting a city school district.Using a 0.10 level of significance,conduct a hypothesis test to determine whether this poll supports the belief of city leaders.

Accepted Answer

Since "more than 60 percent" is a strict

Question 12

According to data from the Environmental Protection Agency,the average daily water consumption for a household of four people in the United States is approximately at least 243 gallons.(Source: http://www.catskillcenter.org/programs/csp/H20/Lesson3/house3.htm)Suppose a state agency plans to test this claim using an alpha level equal to 0.05 and a random sample of 100 households with four people. Calculate the probability of committing a Type II error if the true population mean is 230 gallons.Assume that the population standard deviation is known to be 40 gallons.

Accepted Answer

A) 0.0331 
B) 0.0712 
C) 0.0537 
D) 0.1412 
A) 0.0331 
B) 0.0712 
C) 0.0537 
D) 0.1412

Question 13

Which of the following will be helpful if the decision maker wishes to reduce the chance of making a Type II error?

Accepted Answer

A) Increase the level of significance at which the hypothesis test is conducted. 
B) Increase the sample size. 
C) Both A and B will work. 
D) Neither A nor B will be effective. 
A) Increase the level of significance at which the hypothesis test is conducted. 
B) Increase the sample size. 
C) Both A and B will work. 
D) Neither A nor B will be effective.

Question 14

The cost of a college education has increased at a much faster rate than costs in general over the past twenty years.In order to compensate for this,many students work part- or full-time in addition to attending classes.At one university,it is believed that the average hours students work per week exceeds 20.To test this at a significance level of 0.05,a random sample of n = 20 students was selected and the following values were observed: 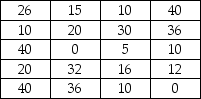  Based on these sample data,the critical value expressed in hours:

Accepted Answer

A) is approximately equal to 25.26 hours. 
B) is approximately equal to 25.0 hours. 
C) cannot be determined without knowing the population standard deviation. 
D) is approximately 22 hours. 
A) is approximately equal to 25.26 hours. 
B) is approximately equal to 25.0 hours. 
C) cannot be determined without knowing the population standard deviation. 
D) is approximately 22 hours.

Question 15

If the Type I error (α)for a given test is to be decreased,then for a fixed sample size n:

Accepted Answer

A) the Type II error (β)will also decrease. 
B) the Type II error (β)will increase. 
C) the power of the test will increase. 
D) a one-tailed test must be utilized. 
A) the Type II error (β)will also decrease. 
B) the Type II error (β)will increase. 
C) the power of the test will increase. 
D) a one-tailed test must be utilized.

Question 16

When the hypothesized proportion is close to 0.50,the spread in the sampling distribution of   is greater than when the hypothesized proportion is close to 0.0 or 1.0.

Accepted Answer

A) True 
 B)False

Question 17

The state insurance commissioner believes that the mean automobile insurance claim filed in her state exceeds $1,700.To test this claim,the agency has selected a random sample of 20 claims and found a sample mean equal to $1,733 and a sample standard deviation equal to $400.They plan to conduct the test using a 0.05 significance level.Given this,the appropriate null and alternative hypotheses are
H0 :   ≤ $1,700
HA :   > $1,700

Accepted Answer

A) True 
 B)False

Question 18

Of the two types of statistical errors,the one that decision makers have most control over is Type I error.

Accepted Answer

A) True 
 B)False

Question 19

Explain why an increase in sample size will reduce the probability of a Type II error but will not impact the probability of a Type I error.

Accepted Answer

The probability of a Type I error is set

Question 20

The cost of a college education has increased at a much faster rate than costs in general over the past twenty years.In order to compensate for this,many students work part- or full-time in addition to attending classes.At one university,it is believed that the average hours students work per week exceeds 20.To test this at a significance level of 0.05,a random sample of n = 20 students was selected and the following values were observed: 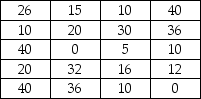  Based on these sample data,which of the following statements is true?

Accepted Answer

A) The standard error of the sampling distribution is approximately 3.04. 
B) The test statistic is approximately t = 0.13. 
C) The research hypothesis that the mean hours worked exceeds 20 is not supported by these sample data. 
D) All of the above are true. 
A) The standard error of the sampling distribution is approximately 3.04. 
B) The test statistic is approximately t = 0.13. 
C) The research hypothesis that the mean hours worked exceeds 20 is not supported by these sample data. 
D) All of the above are true.

Explain what is meant by a p-value.

When testing a hypothesis involving population proportions,an increase in sample size will result in a smaller chance of making a Type I statistical error.

The executive director of the United Way believes that more than 24 percent of the employees in the high-tech industry have made voluntary contributions to the United Way.In order to test this statistically,the appropriate null and alternative hypotheses are: H₀ : ≤ .24 H_A : > .24

In conducting a hypothesis test where the conclusion is to reject the null hypothesis,then either a correct decision has been made or else a Type I error.

A large tire manufacturing company has claimed that its top line tire will average more than 80,000 miles.If a consumer group wished to test this claim,the research hypothesis would be: H_a : μ > 80,000 miles.

The probability of a Type II error decreases as the "true" population value gets farther from the hypothesized population value,given that everything else is held constant.

Which of the following will be helpful if the decision maker wishes to reduce the chance of making a Type II error?

If the Type I error (α)for a given test is to be decreased,then for a fixed sample size n:

When the hypothesized proportion is close to 0.50,the spread in the sampling distribution of is greater than when the hypothesized proportion is close to 0.0 or 1.0.

Of the two types of statistical errors,the one that decision makers have most control over is Type I error.

Explain why an increase in sample size will reduce the probability of a Type II error but will not impact the probability of a Type I error.

The Where,Why,and How of Data Collection

Graphs,Charts and Tablesdescribing Your Data

Describing Data Using Numerical Measures

Introduction to Probability

Discrete Probability Distributions

Introduction to Continuous Probability Distributions

Introduction to Sampling Distributions

Estimating Single Population Parameters

Estimation and Hypothesis Testing for Two Population Parameters

Hypothesis Tests and Estimation for Population Variances

Analysis of Variance

Goodness-Of-Fit Tests and Contingency Analysis

Introduction to Linear Regression and Correlation Analysis

Multiple Regression Analysis and Model Building

Analyzing and Forecasting Time-Series Data

Introduction to Nonparametric Statistics

Introduction to Quality and Statistical Process Control

Filters

Exam 9: Introduction to Hypothesis Testing

Explain what is meant by a p-value.

When testing a hypothesis involving population proportions,an increase in sample size will result in a smaller chance of making a Type I statistical error.

The executive director of the United Way believes that more than 24 percent of the employees in the high-tech industry have made voluntary contributions to the United Way.In order to test this statistically,the appropriate null and alternative hypotheses are: H0 : ≤ .24 HA : > .24

In conducting a hypothesis test where the conclusion is to reject the null hypothesis,then either a correct decision has been made or else a Type I error.

A large tire manufacturing company has claimed that its top line tire will average more than 80,000 miles.If a consumer group wished to test this claim,the research hypothesis would be: Ha : μ > 80,000 miles.

The probability of a Type II error decreases as the "true" population value gets farther from the hypothesized population value,given that everything else is held constant.

Which of the following will be helpful if the decision maker wishes to reduce the chance of making a Type II error?

If the Type I error (α)for a given test is to be decreased,then for a fixed sample size n:

When the hypothesized proportion is close to 0.50,the spread in the sampling distribution of is greater than when the hypothesized proportion is close to 0.0 or 1.0.

Of the two types of statistical errors,the one that decision makers have most control over is Type I error.

Explain why an increase in sample size will reduce the probability of a Type II error but will not impact the probability of a Type I error.

The Where,Why,and How of Data Collection

Graphs,Charts and Tablesdescribing Your Data

Describing Data Using Numerical Measures

Introduction to Probability

Discrete Probability Distributions

Introduction to Continuous Probability Distributions

Introduction to Sampling Distributions

Estimating Single Population Parameters

Estimation and Hypothesis Testing for Two Population Parameters

Hypothesis Tests and Estimation for Population Variances

Analysis of Variance

Goodness-Of-Fit Tests and Contingency Analysis

Introduction to Linear Regression and Correlation Analysis

Multiple Regression Analysis and Model Building

Analyzing and Forecasting Time-Series Data

Introduction to Nonparametric Statistics

Introduction to Quality and Statistical Process Control

Filters

The executive director of the United Way believes that more than 24 percent of the employees in the high-tech industry have made voluntary contributions to the United Way.In order to test this statistically,the appropriate null and alternative hypotheses are: H₀ : ≤ .24 H_A : > .24

A large tire manufacturing company has claimed that its top line tire will average more than 80,000 miles.If a consumer group wished to test this claim,the research hypothesis would be: H_a : μ > 80,000 miles.