Exam 11: Hypothesis Tests and Estimation for Population Variances
Exam 1: The Where,Why,and How of Data Collection167 Questions
Exam 2: Graphs,Charts and Tablesdescribing Your Data139 Questions
Exam 3: Describing Data Using Numerical Measures138 Questions
Exam 4: Introduction to Probability125 Questions
Exam 5: Discrete Probability Distributions161 Questions
Exam 6: Introduction to Continuous Probability Distributions122 Questions
Exam 7: Introduction to Sampling Distributions136 Questions
Exam 8: Estimating Single Population Parameters174 Questions
Exam 9: Introduction to Hypothesis Testing183 Questions
Exam 10: Estimation and Hypothesis Testing for Two Population Parameters121 Questions
Exam 11: Hypothesis Tests and Estimation for Population Variances69 Questions
Exam 12: Analysis of Variance162 Questions
Exam 13: Goodness-Of-Fit Tests and Contingency Analysis105 Questions
Exam 14: Introduction to Linear Regression and Correlation Analysis139 Questions
Exam 15: Multiple Regression Analysis and Model Building152 Questions
Exam 16: Analyzing and Forecasting Time-Series Data133 Questions
Exam 17: Introduction to Nonparametric Statistics103 Questions
Exam 18: Introduction to Quality and Statistical Process Control43 Questions
Select questions type
One of the most important aspects of quality improvement is the idea of reducing the variability in a product or service.For instance,a major bank has worked to reduce the variability in the service time at the drive-through.The managers believe that the standard deviation in service time should not exceed 30 seconds.To test whether this goal is being achieved,a random sample of n = 25 cars is selected each week and the service time for each car is measured.Last week,the mean time was 345 seconds with a standard deviation equal to 38 seconds.Given this information,if the significance level is 0.10,the critical value from the chi-square table is about 34.3.
(True/False)
4.9/5  (31)
(31)
A PC company uses two suppliers for rechargeable batteries for its notebook computers.Two factors are important quality features of the batteries: mean use time and variation.It is desirable that the mean use time be high and the variability be low.Recently,the PC maker conducted a test on batteries from the two suppliers.In the test,9 randomly selected batteries from Supplier 1 were tested and 12 randomly selected batteries from Supplier 2 were tested.The following results were observed:
Supplier 1 Supplier 2
n1 = 9 n2 = 12  1 = 67.25 min
1 = 67.25 min  2 = 72.4 min
s1 = 11.2 min s2 = 9.9 min
Based on these sample results,can the PC maker conclude that a difference exists between the two batteries with respect to the population mean use time? Test using a 0.10 level of significance.
2 = 72.4 min
s1 = 11.2 min s2 = 9.9 min
Based on these sample results,can the PC maker conclude that a difference exists between the two batteries with respect to the population mean use time? Test using a 0.10 level of significance.
(Essay)
4.9/5  (35)
(35)
Assume a sample of size n = 12 has been collected.To perform a hypothesis test of a population variance using a 0.05 level of significance,where the null hypothesis is:
H0 : σ2 = 25
The upper tail critical value is 21.92.
(True/False)
4.9/5  (36)
(36)
A one-tailed hypothesis test for a population variance always has the rejection region in the upper tail.
(True/False)
4.8/5  (31)
(31)
In a two-tailed hypothesis test for the difference between two population variances,if s1 = 3 and s2 = 5,then the test statistic is F = 2.7778.
(True/False)
4.9/5  (32)
(32)
When conducting a one-tailed hypothesis test of a population variance using a sample size of n = 24 and a 0.10 level of significance,the critical value is:
(Multiple Choice)
4.9/5  (37)
(37)
The managers for a vegetable canning facility claim the standard deviation for the ounces per can on the new automated line is less than for the older manual line.Given this,the correct null and alternative hypotheses for performing the statistical test are:
H0 : σ1 = σ2
Ha : σ1 ≠ σ2
(True/False)
4.8/5  (35)
(35)
The F test statistic for testing whether the variances of two populations are the same is always positive.
(True/False)
4.8/5  (38)
(38)
The null hypothesis that two population variances are equal will tend to be rejected if the ratio of the sample variances from each population is substantially larger than 1.0.
(True/False)
4.9/5  (34)
(34)
If a one-tailed F-test is employed when testing a null hypothesis about two population variances,the test statistic is an F-value formed by taking the ratio of the two sample variances so that the sample variance predicted to be larger is placed in the numerator.
(True/False)
4.9/5  (33)
(33)
A sample of n observations is taken from a normally distributed population to estimate the population variance.The degrees of freedom for the chi-square distribution are n-2.
(True/False)
4.8/5  (31)
(31)
The logic behind the F-test for testing whether two populations have equal variances is to determine whether sample variances computed from random samples selected from the two populations differ due to sampling error,or whether the difference is more than can be attributed to sampling error alone,in which case,we conclude that the populations have different variances.
(True/False)
4.8/5  (29)
(29)
The test statistic that is used when testing a null hypothesis for a population variance is the standard normal z-value.
(True/False)
4.9/5  (37)
(37)
When a hypothesis test is to be conducted regarding a population variance,the test statistic will be:
(Multiple Choice)
4.8/5  (37)
(37)
A contract calls for the strength of a steel rod to stand up to pressure of 200 lbs per square inch on average.The contract also requires that the variability in strength for individual steel rods be no more than 5 pounds per square inch.If a random sample of n = 15 rods is selected and the sample standard deviation is 6.7 pounds,the test statistic is approximately χ2 = 25.138.
(True/False)
4.7/5  (39)
(39)
For a given significance level,increasing the sample size will tend to increase the chi-square critical value used in testing the null hypothesis about a population variance.
(True/False)
4.8/5  (30)
(30)
One of the major automobile makers has developed two new engines.At question is whether the two engines have the same variability with respect to miles per gallon.To test this,the following information is available:
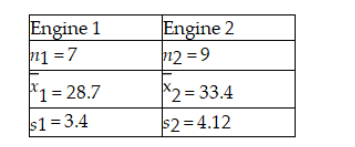 Based on this situation and the information provided,the appropriate null and alternative hypotheses are:
H0 :
Based on this situation and the information provided,the appropriate null and alternative hypotheses are:
H0 : 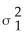 ≠
≠ 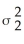 Ha :
Ha : 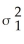 =
= 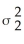
(True/False)
4.8/5  (28)
(28)
When using a chi-square test for the variance of one population,we are assuming that the population is normally distributed.
(True/False)
4.9/5  (33)
(33)
A potato chip manufacturer has two packaging lines and wants to determine if the variances differ between the two lines.They take samples of n1 = 10 bags from line 1 and n2 = 8 bags from line 2.To perform the hypothesis test at the 0.05 level of significance,the critical value is F = 3.68.
(True/False)
4.9/5  (33)
(33)
Showing 21 - 40 of 69
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)