Exam 12: Analysis of Variance
Exam 1: What Is Statistics83 Questions
Exam 2: Describing Data: Frequency Tables, Frequency Distributions, and Graphic Presentation132 Questions
Exam 3: Describing Data: Numerical Measures124 Questions
Exam 4: Describing Data: Displaying and Exploring Data113 Questions
Exam 5: A Survey of Probability Concepts134 Questions
Exam 6: Discrete Probability Distributions131 Questions
Exam 7: Continuous Probability Distributions135 Questions
Exam 8: Sampling Methods and the Central Limit Theorem117 Questions
Exam 9: Estimation and Confidence Intervals131 Questions
Exam 10: One-Sample Tests of Hypothesis110 Questions
Exam 11: Two-Sample Tests of Hypothesis98 Questions
Exam 12: Analysis of Variance134 Questions
Exam 13: Correlation and Linear Regression138 Questions
Exam 14: Multiple Regression Analysis135 Questions
Exam 15: Nonparametric Methods: Nominal Level Hypothesis Tests181 Questions
Exam 16: Nonparametric Methods: Analysis of Ordinal Data138 Questions
Exam 17: Index Numbers137 Questions
Exam 18: Time Series and Forecasting139 Questions
Exam 19: Statistical Process Control and Quality Management136 Questions
Exam 20: An Introduction to Decision Theory115 Questions
Select questions type
Given the following ANOVA table for three treatments each with six observations: 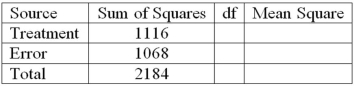 What is the decision regarding the null hypothesis?
What is the decision regarding the null hypothesis?
Free
(Multiple Choice)
4.7/5  (34)
(34)
Correct Answer:
A
When H0: is rejected in ANOVA, we compute ____________ to identify pairs of means that differ.
Free
(Short Answer)
4.7/5  (39)
(39)
Correct Answer:
confidence intervals
A manufacturer of automobile transmissions uses two different processes. Management ordered a study of the production costs to see if there is a difference between the two processes. A summary of the findings is shown next. 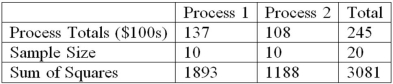 What is the critical value of F at the 1% level of significance?
What is the critical value of F at the 1% level of significance?
Free
(Multiple Choice)
4.8/5  (47)
(47)
Correct Answer:
B
A bottle cap manufacturer with four machines and six operators wants to see if variation in production is due to the machines and/or the operators. The ANOVA table follows. 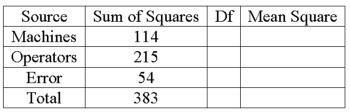
(Essay)
4.8/5  (27)
(27)
A bottle cap manufacturer with four machines and six operators wants to see if variation in production is due to the machines and/or the operators. The ANOVA table follows.  What is the critical value of F for the operator block effect at the 1% level of significance?
B.6 at the 0.01 significance level, with a 5 degrees of freedom numerator and a 15 degrees of freedom denominator.
What is the critical value of F for the operator block effect at the 1% level of significance?
B.6 at the 0.01 significance level, with a 5 degrees of freedom numerator and a 15 degrees of freedom denominator.
(Essay)
4.9/5  (37)
(37)
If a confidence interval for the difference between a pair of treatment means includes 0, then we reject the null hypothesis that there is no difference in the pair of treatment means.
(True/False)
5.0/5  (29)
(29)
To employ ANOVA, the populations should have approximately equal standard deviations.
(True/False)
5.0/5  (30)
(30)
A manufacturer of automobile transmissions uses three different processes. Management ordered a study of the production costs to see if there is a difference among the three processes. A summary of the findings is shown next.  In an ANOVA table, what are the total degrees of freedom?
In an ANOVA table, what are the total degrees of freedom?
(Multiple Choice)
4.9/5  (37)
(37)
A company compared the variance of salaries for employees who have been employed for 5 years or less with employees who have been employed for 10 years or more. They randomly selected 21 employees with 5 years or less experience and 15 employees with 10 years or more experience. The standard deviation for the group with 5 years or less experience was $2,225; the standard deviation for the group with 10 years or more experience was $1,875. What is the F test statistic for the hypothesis test?
(Essay)
4.9/5  (34)
(34)
Two accounting professors decided to compare the variance of their grading procedures. To accomplish this, they each graded the same 10 exams, with the following results: 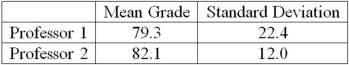 At the 1% level of significance, what is the decision?
At the 1% level of significance, what is the decision?
(Multiple Choice)
4.7/5  (43)
(43)
Two accounting professors decided to compare the variance of their grading procedures. To accomplish this, they each graded the same 10 exams, with the following results: 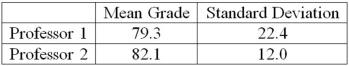 What is the critical value of F at the 0.01 level of significance?
What is the critical value of F at the 0.01 level of significance?
(Multiple Choice)
4.9/5  (35)
(35)
To test the hypothesis that two population variances are equal, a random sample of size 13 was selected from the first population, and a random sample of size 21 was selected from the second population. What are the degrees of freedom to test the hypothesis?
(Essay)
4.8/5  (32)
(32)
The null hypothesis for an ANOVA analysis comparing four treatment means is rejected. The four sample means are 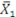 = 10,
= 10,  = 12,
= 12, 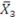 = 15,
= 15,  = 18. The sample size for each treatment is the same. If (
= 18. The sample size for each treatment is the same. If ( 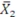 -
- 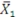 ) is significantly different from zero, then ___________.
) is significantly different from zero, then ___________.
(Multiple Choice)
4.8/5  (26)
(26)
A bottle cap manufacturer with four machines and six operators wants to see if variation in production is due to the machines and/or the operators. The ANOVA table follows. 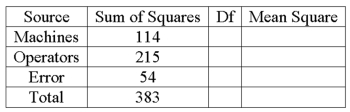 What is the computed value of F for the machines?
What is the computed value of F for the machines?
(Essay)
4.9/5  (33)
(33)
A large department store examined a sample of the 18 credit card sales and recorded the amounts charged for each of three types of credit cards: MasterCard, Visa, and Discover. Six MasterCard sales, seven Visa, and five Discover sales were recorded. The store used an ANOVA to test if the mean sales for each credit card were equal. What are the degrees of freedom for the F statistic?
(Multiple Choice)
4.8/5  (34)
(34)
Two accounting professors decided to compare the variance of their grading procedures. To accomplish this, they each graded the same 10 exams, with the following results: 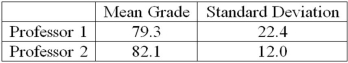 The calculated F ratio is ______.
The calculated F ratio is ______.
(Multiple Choice)
4.7/5  (34)
(34)
What distribution does the F distribution approach as the sample size increases?
(Multiple Choice)
4.9/5  (22)
(22)
The annual dividend rates for a random sample of 16 companies in three different industries, utilities, banking, and insurance were recorded. The ANOVA comparing the mean annual dividend rate among three industries rejected the null hypothesis that the dividend rates were equal. The mean square error (MSE) was 3.36. The following table summarized the results:  When comparing the mean annual dividend rate for companies in the utilities and insurance industries, which of the following 95% confidence interval can be constructed?
When comparing the mean annual dividend rate for companies in the utilities and insurance industries, which of the following 95% confidence interval can be constructed?
(Multiple Choice)
4.9/5  (26)
(26)
In a study of protein breakfast bars, five bars from each of three brands were tested to see if the mean amount of protein per bar differs among the brands. The F critical value for α = 0.05 is _____.
(Short Answer)
4.9/5  (39)
(39)
ANOVA is a statistical approach used to determine whether or not _________.
(Multiple Choice)
4.8/5  (39)
(39)
Showing 1 - 20 of 134
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)