Exam 13: Analysis of Variance and Experimental Design
Exam 1: Data and Statistics98 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Presentations64 Questions
Exam 3: Descriptive Statistics: Numerical Measures156 Questions
Exam 4: Introduction to Probability138 Questions
Exam 5: Discrete Probability Distributions122 Questions
Exam 6: Continuous Probability Distributions165 Questions
Exam 7: Sampling and Sampling Distributions131 Questions
Exam 8: Interval Estimation131 Questions
Exam 9: Hypothesis Tests133 Questions
Exam 10: Statistical Inference About Means and Proportions With Two Populations121 Questions
Exam 11: Inferences About Population Variances91 Questions
Exam 12: Tests of Goodness of Fit and Independence80 Questions
Exam 13: Analysis of Variance and Experimental Design113 Questions
Exam 14: Simple Linear Regression140 Questions
Exam 15: Multiple Regression106 Questions
Exam 16: Regression Analysis: Model Building75 Questions
Exam 17: Index Numbers52 Questions
Exam 18: Forecasting67 Questions
Exam 19: Nonparametric Methods81 Questions
Exam 20: Statistical Methods for Quality Control30 Questions
Exam 21: Decision Analysis65 Questions
Exam 22: Sample Survey63 Questions
Select questions type
Exhibit 13-2
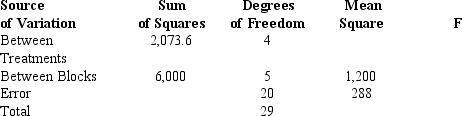 -Refer to Exhibit 13-2.The null hypothesis for this ANOVA problem is
-Refer to Exhibit 13-2.The null hypothesis for this ANOVA problem is
(Multiple Choice)
4.8/5  (32)
(32)
Five drivers were selected to test drive 2 makes of automobiles.The following table shows the number of miles per gallon for each driver driving each car.
 Consider the makes of automobiles as treatments and the drivers as blocks,test to see if there is any difference in the miles/gallon of the two makes of automobiles.Let = .05.
Consider the makes of automobiles as treatments and the drivers as blocks,test to see if there is any difference in the miles/gallon of the two makes of automobiles.Let = .05.
(Essay)
4.8/5  (34)
(34)
Exhibit 13-3
To test whether or not there is a difference between treatments A,B,and C,a sample of 12 observations has been randomly assigned to the 3 treatments.You are given the results below.
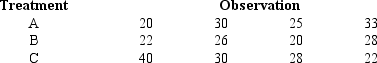 -Refer to Exhibit 13-3.The mean square within treatments (MSE)equals
-Refer to Exhibit 13-3.The mean square within treatments (MSE)equals
(Multiple Choice)
4.8/5  (39)
(39)
Exhibit 13-4
In a completely randomized experimental design involving five treatments,13 observations were recorded for each of the five treatments (a total of 65 observations).The following information is provided.
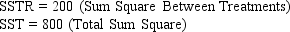 -Refer to Exhibit 13-4.If at 95% confidence we want to determine whether or not the means of the five populations are equal,the p-value is
-Refer to Exhibit 13-4.If at 95% confidence we want to determine whether or not the means of the five populations are equal,the p-value is
(Multiple Choice)
4.8/5  (30)
(30)
In an analysis of variance,one estimate of 2 is based upon the differences between the treatment means and the
(Multiple Choice)
4.8/5  (39)
(39)
In order to compare the life expectancies of three different brands of tires,ten tires of each brand were randomly selected and were subjected to standard wear testing procedures.Information regarding the three brands is shown below.
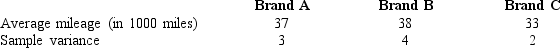 Use the above data and test to see if the mean mileage for all three brands of tires is the same.Let = 0.05.Use both the critical value and p-value approaches.
Use the above data and test to see if the mean mileage for all three brands of tires is the same.Let = 0.05.Use both the critical value and p-value approaches.
(Essay)
4.8/5  (38)
(38)
The heating bills for a selected sample of houses using various forms of heating are given below (values are in dollars).
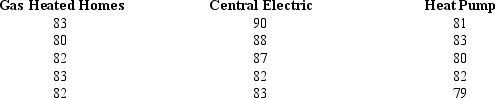 At = 0.05,test to see if there is a significant difference among the average bills of the homes.Use both the critical and p-value approaches.
At = 0.05,test to see if there is a significant difference among the average bills of the homes.Use both the critical and p-value approaches.
(Essay)
4.8/5  (32)
(32)
In order to compare the life expectancies of three different brands of printers,eight printers of each brand were randomly selected.Information regarding the three brands is shown below.
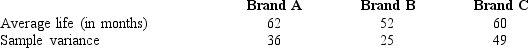 a.Compute the overall mean.
b.State the null and alternative hypotheses to be tested.
c.Show the complete ANOVA table for this test including the test statistic.
d.The null hypothesis is to be tested at 95% confidence.Determine the critical value for this test.What do you conclude?
e.Determine the p-value and use it for the test.
a.Compute the overall mean.
b.State the null and alternative hypotheses to be tested.
c.Show the complete ANOVA table for this test including the test statistic.
d.The null hypothesis is to be tested at 95% confidence.Determine the critical value for this test.What do you conclude?
e.Determine the p-value and use it for the test.
(Essay)
4.8/5  (38)
(38)
The heating bills for a selected sample of houses using various forms of heating are given below.(Values are in dollars. )
 a.At = 0.05,test to see if there is a significant difference among the average heating bills of the homes.Use the p-value approach.
b.Test the above hypotheses using the critical value approach.Let = .05.
a.At = 0.05,test to see if there is a significant difference among the average heating bills of the homes.Use the p-value approach.
b.Test the above hypotheses using the critical value approach.Let = .05.
(Essay)
5.0/5  (39)
(39)
The required condition for using an ANOVA procedure on data from several populations is that the
(Multiple Choice)
4.8/5  (41)
(41)
Exhibit 13-7
The following is part of an ANOVA table that was obtained from data regarding three treatments and a total of 15 observations.
 -Refer to Exhibit 13-7.The conclusion of the test is that the means
-Refer to Exhibit 13-7.The conclusion of the test is that the means
(Multiple Choice)
4.7/5  (27)
(27)
Part of an ANOVA table involving 8 groups for a study is shown below.
 a.Complete all the missing values in the above table and fill in the blanks.
b.Use = 0.05 to determine if there is any significant difference among the means of the eight groups.
a.Complete all the missing values in the above table and fill in the blanks.
b.Use = 0.05 to determine if there is any significant difference among the means of the eight groups.
(Essay)
4.9/5  (44)
(44)
When an analysis of variance is performed on samples drawn from K populations,the mean square between treatments (MSTR)is
(Multiple Choice)
4.8/5  (38)
(38)
A term that means the same as the term "variable" in an ANOVA procedure is
(Multiple Choice)
4.8/5  (36)
(36)
Exhibit 13-7
The following is part of an ANOVA table that was obtained from data regarding three treatments and a total of 15 observations.
 -Refer to Exhibit 13-7.The mean square between treatments (MSTR)is
-Refer to Exhibit 13-7.The mean square between treatments (MSTR)is
(Multiple Choice)
4.9/5  (34)
(34)
In a completely randomized experimental design,7 experimental units were used for the first treatment,9 experimental units for the second treatment,and 14 experimental units for the third treatment.Part of the ANOVA table for this experiment is shown below.
 a.Fill in all the blanks in the above ANOVA table.
b.At 95% confidence using both the critical value and p-value approaches,test to see if there is a significant difference among the means.
a.Fill in all the blanks in the above ANOVA table.
b.At 95% confidence using both the critical value and p-value approaches,test to see if there is a significant difference among the means.
(Essay)
4.7/5  (39)
(39)
Exhibit 13-5
Part of an ANOVA table is shown below.
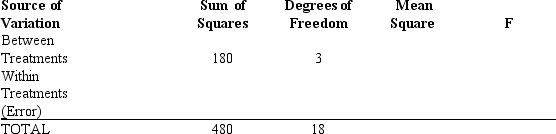 -Refer to Exhibit 13-5.The mean square between treatments (MSTR)is
-Refer to Exhibit 13-5.The mean square between treatments (MSTR)is
(Multiple Choice)
4.8/5  (29)
(29)
In a completely randomized experimental design,18 experimental units were used for the first treatment,10 experimental units for the second treatment,and 15 experimental units for the third treatment.Part of the ANOVA table for this experiment is shown below.
 a.Fill in all the blanks in the above ANOVA table.
b.At 95% confidence,test to see if there is a significant difference among the means.
a.Fill in all the blanks in the above ANOVA table.
b.At 95% confidence,test to see if there is a significant difference among the means.
(Essay)
4.8/5  (39)
(39)
Exhibit 13-3
To test whether or not there is a difference between treatments A,B,and C,a sample of 12 observations has been randomly assigned to the 3 treatments.You are given the results below.
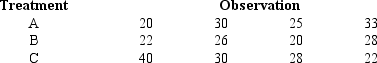 -Refer to Exhibit 13-3.The null hypothesis is to be tested at the 1% level of significance.The p-value is
-Refer to Exhibit 13-3.The null hypothesis is to be tested at the 1% level of significance.The p-value is
(Multiple Choice)
4.8/5  (39)
(39)
Random samples were selected from three populations.The data obtained are shown below.Please note that the sample sizes are not equal.
 a.Compute the overall mean.
b.At 95% confidence using the critical value and p-value approach,test to see if there is a significant difference among the means.
a.Compute the overall mean.
b.At 95% confidence using the critical value and p-value approach,test to see if there is a significant difference among the means.
(Essay)
4.8/5  (39)
(39)
Showing 21 - 40 of 113
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)