Exam 13: Queuing Theory

Exhibit 13. The following questions refer to the information and output below. The university computer lab has 10 computers which are constantly being used by students.Users need help from the one lab assistant fairly often.Students ask for help at a Poisson rate of with an average of 4 requests per hour for any one computer.The assistant answers questions as quickly as possible and the service time follows an exponential distribution with mean of 1 minute per help session.The following queuing analysis spreadsheet was developed from this information. 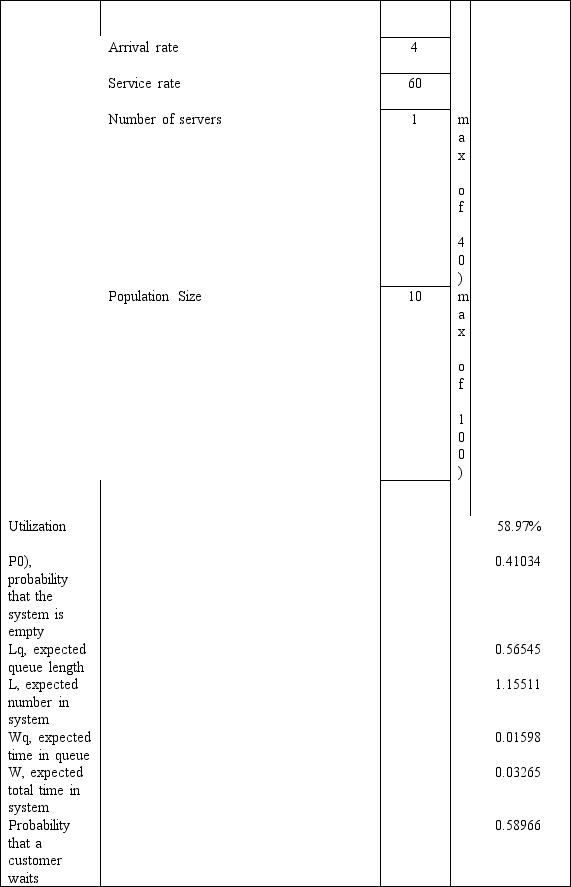-Refer to Exhibit 13.6.Based on this report how much time do students spend getting help before they can resume work on their computers?

If a service system has a constant service time,Poisson arrival rates and 2 servers its Kendall notation is

Which type of queuing system are you likely to encounter at a grocery store?

Exhibit 13. The following questions refer to the information and output below. A grocery store can serve an average of 360 customers per hour.The service times are exponentially distributed.The store has 4 checkout lines each of which serves 90 customers per hour.Customers arrive at the store at a Poisson rate of 240 customers per hour.The following queuing analysis spreadsheet was developed from this information. 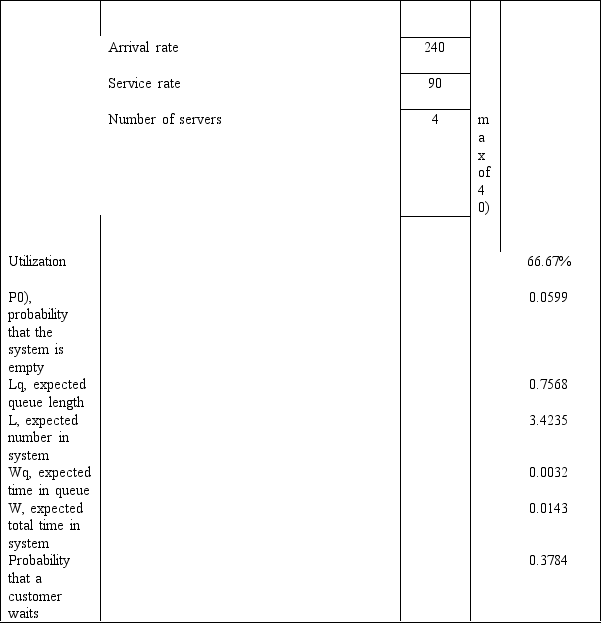-Refer to Exhibit 13.4.What is the Kendall notation for this system?

A Poisson distribution shape can be described as

In the Kendall notation M/G/4,the number 4 indicates

If the service rate decreases as the arrival rate remains constant,then,in general

Exhibit 13. The following questions refer to the information and output below. A computer printer in a large administrative office has a printer buffer memory to store printing jobs)capacity of 3 jobs.If the buffer is full when a user wants to print a file the user is told that the job cannot be printed and to try again later.There are so many users in this office that we can assume that there is an infinite calling population.Jobs arrive at the printer at a Poisson rate of 55 jobs per hour and take an average of 1 minute to print.Printing times are exponentially distributed.The following queuing analysis spreadsheet was developed from this information. -Refer to Exhibit 13.5.Based on this report what is the probability that a computer user will be told to resubmit a print job at a later time?

Exhibit 13. The following questions refer to the information and output below. The university computer lab has 10 computers which are constantly being used by students.Users need help from the one lab assistant fairly often.Students ask for help at a Poisson rate of with an average of 4 requests per hour for any one computer.The assistant answers questions as quickly as possible and the service time follows an exponential distribution with mean of 1 minute per help session.The following queuing analysis spreadsheet was developed from this information. -Refer to Exhibit 13.6.Based on this report what is the probability that a student will not get instantaneous help?

Exhibit 13.3 The following questions refer to the information below. A company has recorded the following customer inter-arrival times and service times for 10 customers at one of its single teller service lines.Assume the data are exponentially distributed and the 10 data points represent a reasonable sample. All time in minutes Customer Inter-arrival Service 1 11.08 2.20 2 2.50 2.50 3 6.00 1.10 4 5.75 14.50 5 8.50 2.00 6 4.15 2.70 7 15.50 5.00 8 13.00 8.50 9 10.50 5.00 10 6.00 1.50 -Refer to Exhibit 13.3.What is the mean arrival rate per hour?

Exhibit 13.1 The following questions are based on the output below. A store currently operates its service system with 1 operator.Arrivals follow a Poisson distribution and service times are exponentially distributed.The following spreadsheet has been developed for the system. -Refer to Exhibit 13.1.What is the probability that a customer can go directly into service without waiting in line?

Joe's Copy Center has 10 copiers.They break down and require service quite often.Time between breakdowns follows an exponential distribution for each copier.The repair person services machines as quickly as possible,but the service time follows an exponential distribution.What type of system is it?

An arrival process is memoryless if

Exhibit 13. The following questions refer to the information and output below. The university computer lab has 10 computers which are constantly being used by students.Users need help from the one lab assistant fairly often.Students ask for help at a Poisson rate of with an average of 4 requests per hour for any one computer.The assistant answers questions as quickly as possible and the service time follows an exponential distribution with mean of 1 minute per help session.The following queuing analysis spreadsheet was developed from this information. -Refer to Exhibit 13.6.What is the Kendall notation for this system?

Exhibit 13.3 The following questions refer to the information below. A company has recorded the following customer inter-arrival times and service times for 10 customers at one of its single teller service lines.Assume the data are exponentially distributed and the 10 data points represent a reasonable sample. All time in minutes Customer Inter-arrival Service 1 11.08 2.20 2 2.50 2.50 3 6.00 1.10 4 5.75 14.50 5 8.50 2.00 6 4.15 2.70 7 15.50 5.00 8 13.00 8.50 9 10.50 5.00 10 6.00 1.50 -Refer to Exhibit 13.3.What is the mean service rate per hour?

A company has recorded the following list of service rates customers/hour)for one of its servers.What is the mean service time for this server? Customers / hour: 4,4,5,6,5,4,3,4,3,5,5,6

Exhibit 13.2 The following questions refer to the information and output below. A barber shop has one barber who can give 12 haircuts per hour.Customers arrive at a rate of 8 customers per hour.Customer inter-arrival times and service times are exponentially distributed.The following queuing analysis spreadsheet was developed from this information. -Refer to Exhibit 13.2.Based on this report what is the average total time spent waiting for a haircut and getting a haircut?

The number of arrivals to a store follows a Poisson distribution with mean λ = 10/hour.What is the mean inter-arrival time?

Exhibit 13.2 The following questions refer to the information and output below. A barber shop has one barber who can give 12 haircuts per hour.Customers arrive at a rate of 8 customers per hour.Customer inter-arrival times and service times are exponentially distributed.The following queuing analysis spreadsheet was developed from this information. -Refer to Exhibit 13.2.Based on this report what is the average waiting time before the barber begins a customer's haircut?

The service times for a grocery store with one checkout line have a mean of 3 minutes and a standard deviation of 20 seconds.Customer arrivals at the checkout stand follow a Poisson distribution.What type of system is it?