Exam 4: Sensitivity Analysis and the Simplex Method

Slack variables

Exhibit 4.2 The following questions correspond to the problem below and associated Analytic Solver Platform sensitivity report. Robert Hope received a welcome surprise in this management science class;the instructor has decided to let each person define the percentage contribution to their grade for each of the graded instruments used in the class.These instruments were: homework,an individual project,a mid-term exam,and a final exam.Robert's grades on these instruments were 75,94,85,and 92,respectively.However,the instructor complicated Robert's task somewhat by adding the following stipulations: · homework can account for up to 25% of the grade,but must be at least 5% of the grade; · the project can account for up to 25% of the grade,but must be at least 5% of the grade; · the mid-term and final must each account for between 10% and 40% of the grade but cannot account for more than 70% of the grade when the percentages are combined;and · the project and final exam grades may not collectively constitute more than 50% of the grade.The following LP model allows Robert to maximize his numerical grade. Let ^W₁ ^{= weight assigned to homework} W₂ = weight assigned to the project W₃ = weight assigned to the mid-term W₄ = weight assigned to the final MAX: ^75W₁ ^{+ 94W}₂ ^{+ 85W}₃ ^{+ 92W}₄ Subject to: ^W₁ ^{+ W}₂ ^{+ W}₃ ^{+ W}₄ ^{= 1} W₃ + W₄ ≤ 0.70 W₃ + W₄ ≥ 0.50 0.05 ≤ W₁ ≤ 0.25 0.05 ≤ W₂ ≤ 0.25 0.10 ≤ W₃ ≤ 0.40 0.10 ≤ W₄ ≤ 0.40 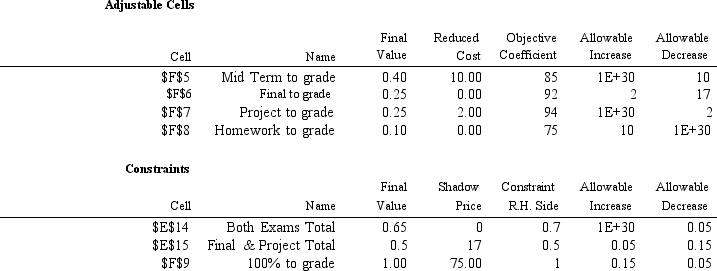-Refer to Exhibit 4.2.Based on the Analytic Solver Platform sensitivity report information,Robert has been approved by his instructor to increase the total weight allowed for the project and final exam to 0.50 plus the allowable increase.When Robert re-solves his model,what will his new final grade score be?

The solution to an LP problem is degenerate if

Exhibit 4.1 The following questions are based on the problem below and accompanying Analytic Solver Platform sensitivity report. Carlton construction is supplying building materials for a new mall construction project in Kansas.Their contract calls for a total of 250,000 tons of material to be delivered over a three-week period.Carlton's supply depot has access to three modes of transportation: a trucking fleet,railway delivery,and air cargo transport.Their contract calls for 120,000 tons delivered by the end of week one,80% of the total delivered by the end of week two,and the entire amount delivered by the end of week three.Contracts in place with the transportation companies call for at least 45% of the total delivered be delivered by trucking,at least 40% of the total delivered be delivered by railway,and up to 15% of the total delivered be delivered by air cargo.Unfortunately,competing demands limit the availability of each mode of transportation each of the three weeks to the following levels all in thousands of tons): Week TruckingLimits Railway Limits Air Cargo Limits 1 45 60 15 2 50 55 10 3 55 45 5 Costs \ per 1000 tons) \ 200 \ 140 \ 400 The following is the LP model for this logistics problem. Let ^X_ij ^{= amount shipped by mode i in week j} where i = 1Truck),2Rail),3Air)and j = 1,2,3 _Let WL_ij = weekly limit of mode i in week j as provided in above table) 200X₁₁ + X₁₂ + X₁₃)+ 140X₂₁ + X₂₂ + X₂₃)+ 500X₃₁ + MIN: X + X ) Subject to: 32 33 ^X_ij ^{? WL} _ij ^{for all i and j} Weekly limits by mode X₁₁ + X₁₂ + X₁₃ + X₂₁ + X₂₂ + X₂₃ + X₃₁ + X₃₂ + X₃₃ ? 250 Total at end of three weeks ^X₁₁ ^{+ X}₂₁ ^{+ X}₃₁ ^{+ X}₁₂ ^{+ X}₂₂ ^{+ X}₃₂ ^{? 200} Total at end of two weeks ^X₁₁ ^{+ X}₂₁ ^{+ X}₃₁ ^{? 120} Total at end of first week ^X₁₁ ^{+ X}₁₂ ^{+ X}₁₃ ^{? 0.45*250} Truck mix requirement ^X₂₁ ^{+ X}₂₂ ^{+ X}₂₃ ^{? 0.40*250} Rail mix requirement ^X₃₁ ^{+ X}₃₂ ^{+ X}₃₃ ^{? 0.15*250} Air mix limit X_ij ? 0 for all i and j Final Reduced Objective Allowable Allowable Value Cost Coefficient Increase Decrease Cell Name \ \ 6 Week 1 by Truck 45 0 200 360 1+30 \ \ 6 Week 1 by Rail 60 0 140 360 1+30 \ \ 6 Week 1 by Air 15 0 500 1+30 360 \ \ 7 Week 2 by Truck 50 0 200 0 1+30 \ \ 7 Week 2 by Rail 55 0 140 0 1+30 \ \ 7 Week 2 by Air 0 360 500 1+30 360 \ \ 8 Week 3 by Truck 13 0 200 1+30 0 \ \ 8 Week 3 by Rail 12 0 140 60 0 \ F\ 8 Week 3 by Air 0 360 500 1+30 360 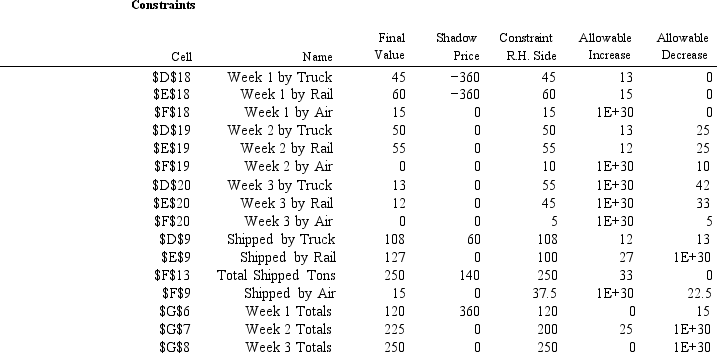-Refer to Exhibit 4.1.Should the company negotiate for additional air delivery capacity?

The simplex method of linear programming LP):

If the shadow price for a resource is 0 and 150 units of the resource are added what happens to the objective function value?

A formulation has 10 variables and 4 constraints not counting non-negativity).How many variables are basic?

A binding less than or equal to ≤)constraint in a maximization problem means

When a solution is degenerate the shadow prices and their ranges

The shadow price of a nonbinding constraint is

The allowable increase for a constraint is

Which of the following statements is false concerning either of the Allowable Increase and Allowable Decrease columns in the Sensitivity Report?

The reduced cost for a changing cell decision variable)is

The optimization technique that locates solutions in the interior of the feasible region is known as

The sensitivity analysis provides information about which of the following?

A variable with a final value equal to its simple lower or upper bound and a reduced cost of zero indicates that

Finding a robust solution to an LP problem

If the allowable increase for a constraint is 100 and we add 110 units of the resource what happens to the objective function value?

When a manager considers the effect of changes in an LP model's coefficients he/she is performing

A farmer is planning his spring planting.He has 20 acres on which he can plant a combination of Corn,Pumpkins and Beans.He wants to maximize his profit but there is a limited demand for each crop.Each crop also requires fertilizer and irrigation water both of which are in short supply.The following table summarizes the data for the problem. $\begin{array}{r|rcccc}\text { Crop } & \begin{array}{c}\text { Profit per } \\\text { Acre } \$)\end{array} & \begin{array}{c}\text { Yield per } \\\text { Acre 1b) }\end{array} & \begin{array}{c}\text { Maximum } \\\text { Demønd lb) }\end{array} & \begin{array}{c}\text { Imigation } \\\text { acre ft) }\end{array} & \begin{array}{c}\text { Fertilizer } \\\text { poundls/acre) }\end{array} \\\hline \text { Corn } & 2,100 & 21,000 & 200,000 & 2 & 500 \\\text { Pumpkin } & 900 & 10,000 & 180,000 & 3 & 400 \\\text { Beans } & 1,050 & 3,500 & 80,000 & 1 & 300\end{array}$ Based on the following Analytic Solver Platform sensitivity output,how much can the price of Corn drop before it is no longer profitable to plant corn? $\text { Changing Cells }$ Final Rechiced Objective Allowable Allowable Cell Name Value Cost Coefficient Increase Decrease \B \4 Acres of Corn 9.52 0 2100 1+30 350 \C \4 Acres of Pumplin 0 -500.01 899.99 500.01 1+30 \ D\ 4 Acres of Peans 1079 0 1050 210 375.00