Exam 10: Conservation of Angular Momentum
Exam 1: Systems of Measurement86 Questions
Exam 2: Motion in One Dimension103 Questions
Exam 3: Motion in Two and Three Dimensions67 Questions
Exam 4: Newtons Laws117 Questions
Exam 5: Applications of Newtons Laws75 Questions
Exam 6: Work and Energy71 Questions
Exam 7: Conservation of Energy73 Questions
Exam 8: Systems of Particles and Conservation of Linear Momentum107 Questions
Exam 9: Rotation119 Questions
Exam 10: Conservation of Angular Momentum67 Questions
Exam 11: Gravity90 Questions
Exam 12: Static Equilibrium and Elasticity65 Questions
Exam 13: Fluids91 Questions
Exam 14: Oscillations138 Questions
Exam 15: Wave Motion122 Questions
Exam 16: Superposition and Standing Waves125 Questions
Exam 17: Temperature and the Kinetic Theory of Gases85 Questions
Exam 18: Heat and the First Law of Thermodynamics114 Questions
Exam 19: The Second Law of Thermodynamics61 Questions
Exam 20: Thermal Properties and Processes54 Questions
Select questions type
A constant torque of 15 N · m acts for 3.0 s on a system of mass 2.0 kg. The change in angular momentum of the system during this period of time is
(Multiple Choice)
4.7/5  (32)
(32)
In a playground there is a small merry-go-round of radius 1.25 m and mass 175 kg. Assume the merry-go-round to be a uniform disc. A child of mass 45 kg runs at a speed of 3.0 m/s tangent to the rim of the merry-go-round (initially at rest) and jumps on. If we neglect friction, what is the angular speed of the merry-go-round after the child has jumped on and is standing at its outer rim?
(Multiple Choice)
4.8/5  (32)
(32)
A woman sits on a spinning piano stool with her arms folded. When she extends her arms, which of the following occurs?
(Multiple Choice)
4.9/5  (38)
(38)
If the distance between two hydrogen atoms of H2 is 0.1 nm, what is its E0r?
(Multiple Choice)
4.9/5  (32)
(32)
Large meteors can impact the earth with speeds of 80,000 km/h. If such a meteor were to impact at a point on the plane of Earth's equator, resulting in a change of Earth's angular momentum by 80 × 1012 kg • m2/s (an insignificant change, thankfully), calculate the mass of the meteor. (The radius of Earth = 6.38 106 m.)
(Multiple Choice)
4.7/5  (34)
(34)
A particle of mass m is moving with a velocity 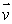 , in the yz plane as shown. The vector that most nearly represents the angular momentum about the x axis is
, in the yz plane as shown. The vector that most nearly represents the angular momentum about the x axis is 
(Multiple Choice)
4.9/5  (39)
(39)
Use the figure to the right to answer the next problem.
A ball of mass of mass m is tied with a light string to a vertical post of radius Rp and slides on a frictionless horizontal surface. As the ball travels around the post, the string wraps around the post, shortening the length. At t = 0 s, its speed is vo and radius is ro. 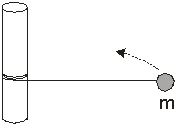 -As the string wraps around the post, which of the following statements is true?
-As the string wraps around the post, which of the following statements is true?
(Multiple Choice)
4.8/5  (36)
(36)
A force  x in the negative x direction is applied to a particle in the xy plane. The arrow that best represents the torque produced by
x in the negative x direction is applied to a particle in the xy plane. The arrow that best represents the torque produced by 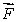 x on the particle with respect to the origin is
x on the particle with respect to the origin is 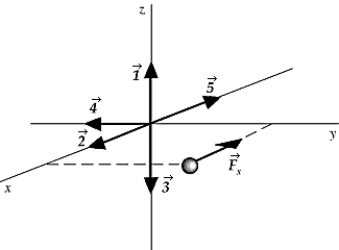
(Multiple Choice)
4.9/5  (35)
(35)
A torque is applied to a bolt by hanging a weight w from the end of the wrench, as shown. The coordinate axis along which the torque vector is directed is 
(Multiple Choice)
4.8/5  (30)
(30)
An athletic can twirl a baton so that it reaches an angular velocity of 12 rad/s from rest in 0.5 s. If the length of a uniform baton is 0.8 m and the mass is 0.5 kg, what is the torque needed to reach the angular velocity?
(Multiple Choice)
4.8/5  (43)
(43)
We would like to compare the angular momentum of Mars about its axis of rotation with that of Earth's. The mass of Mars is 11% that of Earth, with a radius 53% that of Earth, and a rotational period 103% that of Earth. Assuming both planets to be uniform spheres calculate the ratio of the angular momentum of Mars to that of Earth.
(Multiple Choice)
4.9/5  (34)
(34)
A disc with moment of inertia I1 = 40 kg.m2 and angular velocity 1 = 20 rad/s is dropped on to a stationary second disc along the axis of rotation. The second disc has moment of inertia I2 = 60 kg.m2. How much rotational kinetic energy is lost?
(Multiple Choice)
4.9/5  (32)
(32)
A woman sits on a stool that can turn friction-free about its vertical axis. She is handed a spinning bicycle wheel that has angular momentum 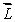 0 and she turns it over (that is, through 180º). She thereby acquires an angular momentum of magnitude
0 and she turns it over (that is, through 180º). She thereby acquires an angular momentum of magnitude 
(Multiple Choice)
4.8/5  (40)
(40)
A spinning bicycle wheel with a loaded rim (essentially a hoop) is supported by a line at one end of its axle. The radius of the wheel is 0.305 m, and the wheel has a mass of 3.63 kg. It is spinning at 80.0 rad/s, and the center of mass is 15.2 cm from the point of support. The angular velocity of precession is
(Multiple Choice)
4.7/5  (41)
(41)
A wheel of moment of inertia 0.136 kg · m2 is spinning with an angular speed of 5000 rad/s. A torque is applied about an axis perpendicular to the spin axis. If the applied torque has a magnitude of 67.8 N · m, the angular velocity of precession will be
(Multiple Choice)
4.8/5  (33)
(33)
A hoop rotates about an axis through its center with an angular velocity of 40.0 rad/s. If the rotational kinetic energy of the hoop is 400 J, its angular momentum is
(Multiple Choice)
4.8/5  (32)
(32)
The angular momentum vector for a spinning wheel lies along its axle and is pointed east. To make this vector point south, it is necessary to exert a force on the east end of the axle in which direction?
(Multiple Choice)
4.8/5  (30)
(30)
The energy-level diagram that most closely represents a rotating molecule with constant moment of inertia is 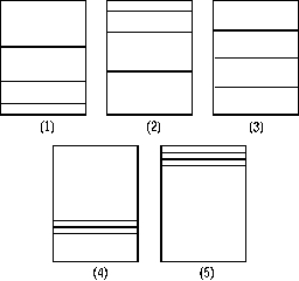
(Multiple Choice)
4.7/5  (30)
(30)
A gyroscopic wheel spins clockwise as shown. The set of vectors that correctly describes the directions of the torque  , angular momentum
, angular momentum  , and angular velocity of precession
, and angular velocity of precession  p, is
p, is 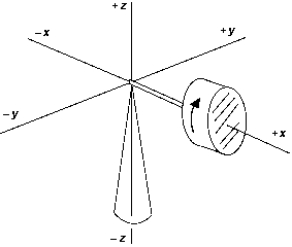
(Multiple Choice)
4.9/5  (32)
(32)
Showing 41 - 60 of 67
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)